Mothers Major Depression when Children were at Age 2, 3, 5, and 9 - PDF document

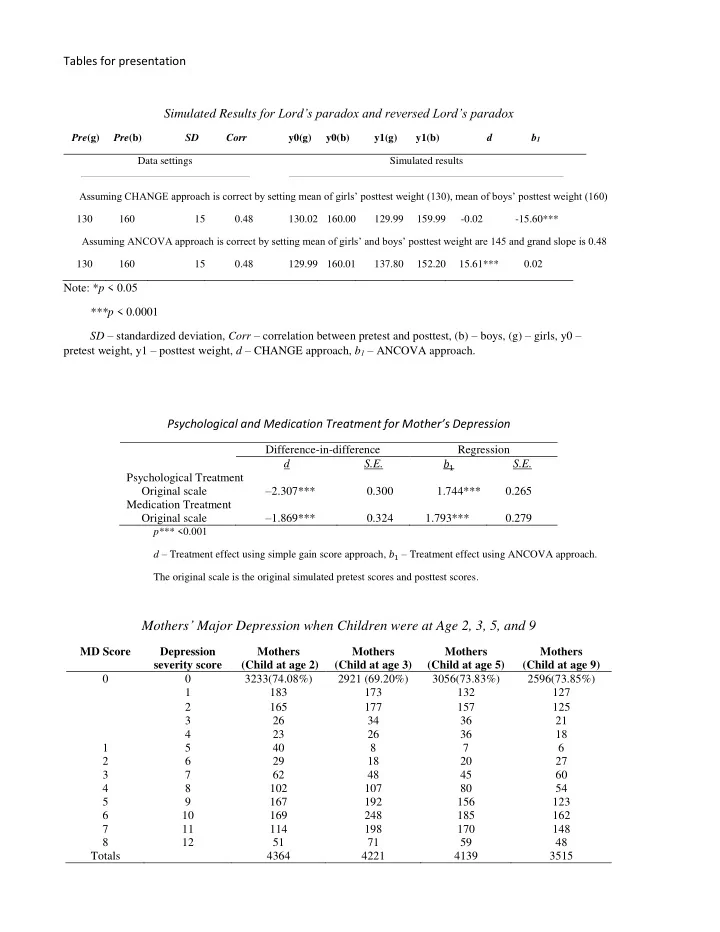
Tables for presentation Simulated Results for Lord’s paradox and reversed Lord’s paradox Pre (g) Pre (b) SD Corr y0(g) y0(b) y1(g) y1(b) d b 1 Data settings Simulated results Assuming CHANGE approach is correct by setting mean of girls’ posttest weight (130), mean of boys’ posttest weight (160) 130 160 15 0.48 130.02 160.00 129.99 159.99 -0.02 -15.60*** Assuming ANCOVA approach is correct by setting mean of girls’ and boys’ posttest weight are 145 and grand slope is 0.48 130 160 15 0.48 129.99 160.01 137.80 152.20 15.61*** 0.02 Note: * p < 0.05 ***p < 0.0001 SD – standardized deviation, Corr – correlation between pretest and posttest, (b) – boys, (g) – girls, y0 – pretest weight, y1 – posttest weight, d – CHANGE approach, b 1 – ANCOVA approach. Psychological and Medication Treatment for Mother ’s Depression Difference-in-difference Regression 𝑐 1 d S.E. S.E. Psychological Treatment – 2.307*** Original scale 0.300 1.744*** 0.265 Medication Treatment – 1.869*** Original scale 0.324 1.793*** 0.279 p *** <0.001 d – Treatment effect using simple gain score approach, 𝑐 1 – Treatment effect using ANCOVA approach. The original scale is the original simulated pretest scores and posttest scores. Mothers ’ Major Depression when Children were at Age 2, 3, 5, and 9 MD Score Depression Mothers Mothers Mothers Mothers severity score (Child at age 2) (Child at age 3) (Child at age 5) (Child at age 9) 0 0 3233(74.08%) 2921 (69.20%) 3056(73.83%) 2596(73.85%) 1 183 173 132 127 2 165 177 157 125 3 26 34 36 21 4 23 26 36 18 1 5 40 8 7 6 2 6 29 18 20 27 3 7 62 48 45 60 4 8 102 107 80 54 5 9 167 192 156 123 6 10 169 248 185 162 7 11 114 198 170 148 8 12 51 71 59 48 Totals 4364 4221 4139 3515

Simulation Results for Lord’s Paradox and Reversed Paradox, Varying Normality, SD, Slope Correlation, & Independence of Pretest Weight from Gender 𝒄 𝟐 Normality SD (g) SD (b) Corr (g) Corr (b) y0(g) y0(b) y1(g) y1(b) d Data settings Simulated results Pretest means are different: mean of girls’ pretest weight (130), mean of boys’ pretest weight (160) Assuming the simple gain score null H 0 is correct, with mean of girls’ posttest weight to 130, & mean of boys’ posttest weight to 160 Yes 15 15 0.48 0.48 130.02 160.00 129.99 159.99 -0.02 -15.60*** Yes 5 15 0.48 0.48 130.01 160.00 130.00 159.99 0.00 -15.60*** Yes 15 15 0 0.48 130.02 160.00 129.98 159.99 -0.03 -22.81*** Yes 15 15 0.48 0 130.02 160.01 129.99 159.99 -0.01 -22.79*** Yes 5 15 0 0.48 130.01 160.00 129.99 159.99 0.00 -17.05*** Yes 5 15 0.48 0 130.01 160.01 130.00 159.99 0.01 -28.54*** No 15 15 0.48 0.48 85.00 92.50 82.00 88.00 1.50 -5.96*** No 5 15 0.48 0.48 85.00 92.50 82.00 88.00 1.50 -5.97*** No 15 15 0.48 0 85.00 92.50 82.00 88.00 1.51 -5.97*** No 15 15 0 0.48 85.00 92.50 82.01 88.00 1.50 -5.97*** No 5 15 0 0.48 85.00 92.50 82.00 88.00 1.50 -5.98*** No 5 15 0.48 0 85.00 92.50 82.00 88.00 1.51 -5.99*** Assuming the ANCOVA null H 0 is correct by setting means of girls’ and boys’ posttest weights to those predicted by slope of 0.48 Yes 15 15 0.48 0.48 129.99 160.01 137.80 152.20 15.61*** 0.02 Yes 5 15 0.48 0.48 130.00 160.01 137.80 152.20 15.61*** 0.05 No 5 15 0.48 0.48 85.00 92.50 83.56 86.44 4.63 -2.86 No 15 15 0.48 0.48 84.99 92.50 83.56 86.44 4.63 -2.84 Pretest means are the same: mean of girls’ pretest weight (145), mean of boys’ pretest weight (145) Assuming the simple gain score null H 0 is correct by setting the means of girls’ and boys’ posttest weights to 145 Yes 5 15 0.48 0.48 145.00 145.01 145.00 145.00 0.01 0.00 Yes 15 15 0.48 0.48 144.99 145.01 145.00 145.00 0.01 0.00 No 5 15 0.48 0.48 88.75 88.75 85.00 85.00 0.01 0.00 No 15 15 0.48 0.48 88.74 88.75 85.00 85.00 0.01 0.00 Assuming alternative H A is correct, setting the mean of girls’ posttest weight to 130 & the mean of boys’ posttest weight to 160 Yes 5 15 0 0.48 145.01 145.00 129.99 159.99 -30.00*** -30.00*** Yes 15 15 0 0.48 145.02 145.00 129.98 159.99 -30.03*** -30.02*** Yes 5 15 0.48 0 145.01 145.01 130.00 159.99 -29.99*** -30.00*** Yes 15 15 0.48 0 145.02 145.01 129.99 159.99 -30.01*** -30.01*** Yes 5 15 0.48 0.48 145.01 145.00 130.00 159.99 -30.00*** -30.00*** Yes 15 15 0.48 0.48 145.02 145.00 129.99 159.99 -30.02*** -30.01*** No 5 15 0 0.48 88.75 88.75 82.00 88.00 -6.00* -5.99*** No 15 15 0 0.48 88.75 88.75 82.01 88.00 -6.00* -5.99*** No 5 15 0.48 0 88.75 88.75 82.00 88.00 -5.99* -5.99*** No 15 15 0.48 0 88.75 88.75 82.00 88.00 -5.99* -5.99*** No 5 15 0.48 0.48 88.75 88.75 82.00 88.00 -6.00* -5.99*** No 15 15 0.48 0.48 88.75 88.75 82.00 88.00 -6.00* -5.99*** Note: * p < .05. ***p < .0001 Normality – normal distribution, SD – standard deviation, Corr – correlation between pretest and posttest, (b) – boys, (g) – girls, y0 – pretest weight, y1 – posttest weight, d – CHANGE approach, 𝑐 1 – ANCOVA approach. The first bold font is Lord’s paradox and the second bold font is Lord’ s paradox reversed.

Table 3 Simulation Results on Varying Pretest and Posttest Different for Girls b 1 y0(g) y1(g) y0(g) y0(b) y1(g) y1(b) d Data setting Simulated data Analysis results 130 130 130.02 160.00 129.99 159.99 -0.02 -15.60*** 135 130 135.02 160.00 129.99 159.99 -5.02*** -18.00*** 140 130 140.02 160.00 129.99 159.99 -10.02*** -20.40*** 145 130 145.02 160.00 129.99 159.99 -15.02*** -22.81*** 150 130 150.02 160.00 129.99 159.99 -20.02*** -25.21*** 155 130 155.02 160.00 129.99 159.99 -25.02*** -27.61*** 160 130 160.02 160.00 129.99 159.99 -30.02*** -30.01*** 130 135 130.02 160.00 134.99 159.99 4.98*** -10.60*** 135 135 135.02 160.00 134.99 159.99 -0.02 -13.00*** 140 135 140.02 160.00 134.99 159.99 -5.02*** -15.40*** 145 135 145.02 160.00 134.99 159.99 -10.02*** -17.81*** 150 135 150.02 160.00 134.99 159.99 -15.02*** -20.21*** 155 135 155.02 160.00 134.99 159.99 -20.02*** -22.61*** 160 135 160.02 160.00 134.99 159.99 -25.02*** -25.01*** 130 140 130.02 160.00 139.99 159.99 9.98*** -5.60*** 135 140 135.02 160.00 139.99 159.99 4.98*** -8.00*** 140 140 140.02 160.00 139.99 159.99 -0.02 -10.40*** 145 140 145.02 160.00 139.99 159.99 -5.02*** -12.81*** 150 140 150.02 160.00 139.99 159.99 -10.02*** -15.21*** 155 140 155.02 160.00 139.99 159.99 -15.02*** -17.61*** 160 140 160.02 160.00 139.99 159.99 -20.02*** -20.01*** 130 145 130.02 160.00 144.99 159.99 14.98*** -0.60 135 145 135.02 160.00 144.99 159.99 9.98*** -3.00* 140 145 140.02 160.00 144.99 159.99 4.98*** -5.40*** 145 145 145.02 160.00 144.99 159.99 -0.02 -7.81*** 150 145 150.02 160.00 144.99 159.99 -5.02*** -10.21*** 155 145 155.02 160.00 144.99 159.99 -10.02*** -12.61*** 160 145 160.02 160.00 144.99 159.99 -15.02*** -15.01*** 130 150 130.02 160.00 149.99 159.99 19.98*** 4.40** 135 150 135.02 160.00 149.99 159.99 14.98*** 2.00

140 150 140.02 160.00 149.99 159.99 9.98*** -0.40 145 150 145.02 160.00 149.99 159.99 4.98*** -2.81* 150 150 150.02 160.00 149.99 159.99 -0.02 -5.21*** 155 150 155.02 160.00 149.99 159.99 -5.02*** -7.61*** 160 150 160.02 160.00 149.99 159.99 -10.02*** -10.01*** 130 155 130.02 160.00 154.99 159.99 24.98*** 9.40*** 135 155 135.02 160.00 154.99 159.99 19.98*** 7.00*** 140 155 140.02 160.00 154.99 159.99 14.98*** 4.60*** 2.19 a 145 155 145.02 160.00 154.99 159.99 9.98*** 150 155 150.02 160.00 154.99 159.99 4.98*** -0.21 155 155 155.02 160.00 154.99 159.99 -0.02 -2.61* 160 155 160.02 160.00 154.99 159.99 -5.02*** -5.01*** 130 160 130.02 160.00 159.99 159.99 29.98*** 14.40*** 135 160 135.02 160.00 159.99 159.99 24.98*** 12.00*** 140 160 140.02 160.00 159.99 159.99 19.98*** 9.60*** 145 160 145.02 160.00 159.99 159.99 14.98*** 7.19*** 150 160 150.02 160.00 159.99 159.99 9.98*** 4.79*** 155 160 155.02 160.00 159.99 159.99 4.98*** 2.39* 160 160 160.02 160.00 159.99 159.99 -0.02 -0.01 Note: a p < .1, * p < .05, **p < .001, ***p < .0001 (b) – boys, (g) – girls, y0 – pretest weight, y1 – posttest weight, d – CHANGE approach, 𝑐 1 – ANCOVA approach. Data setting: boys pretest/posttest mean = 160, slope for boys/girls = 0.48, standard deviation = 15. The italic font are results in opposite directions. The first bold font is Lord’s paradox and the second bold font is Lord’s paradox reversed.

Cross-Lagged Panel Model of M others’ Depression Featuring Wave 3, 4, and 5 PSY_TRT3 PSY_TRT4 Depression3 Depression4 Depression5 MED_TRT3 MED_TRT4 Depression3 Depression4 Depression5 Latent Growth Model of M others’ Depression Featuring Wave 3, 4, and 5 Depression3 Intercept Depression4 PSY_TRT3 Slope 1 Depression5 PSY_TRT4 Slope 2 Depression3 Intercept MED_TRT3 Depression4 Slope 1 MED_TRT4 Depression5 Slope 2