Meshing Formally introd by Voronoi in 1908 Meshing Delaunay 1934 - PDF document

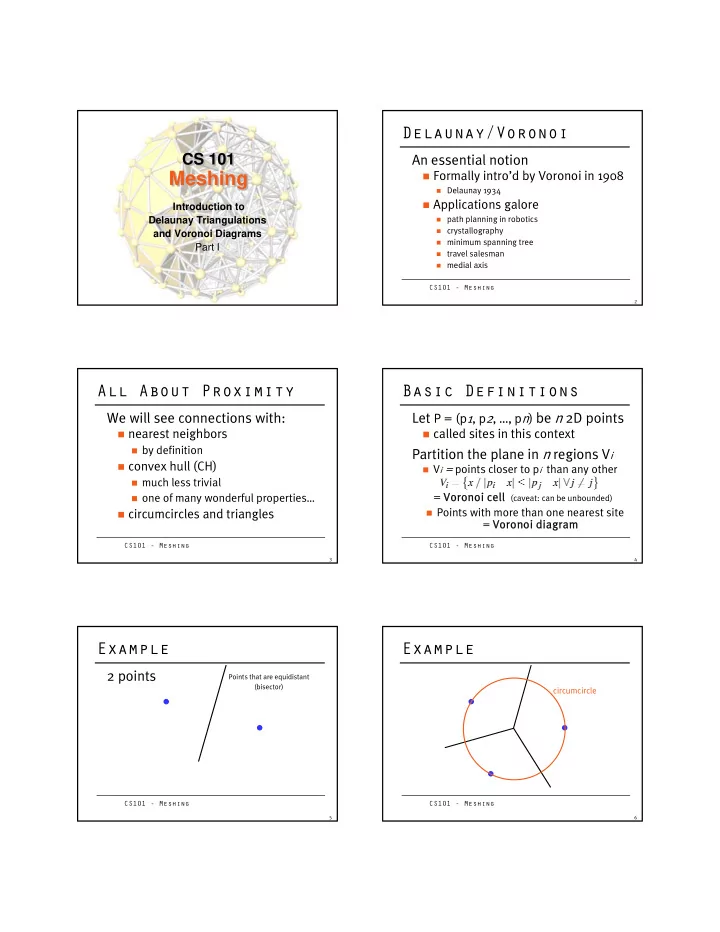
Delaunay/Voronoi CS 101 An essential notion CS 101 Meshing � Formally intro’d by Voronoi in 1908 Meshing � Delaunay 1934 � Applications galore Introduction to Delaunay Triangulations � path planning in robotics � crystallography and Voronoi Diagrams � minimum spanning tree Part I � travel salesman � medial axis CS101 - Meshing 2 All About Proximity Basic Definitions We will see connections with: Let P = (p 1 , p 2 , …, p n ) be n 2D points � nearest neighbors � called sites in this context � by definition Partition the plane in n regions V i � convex hull (CH) � V i = points closer to p i than any other � much less trivial � one of many wonderful properties… = Voro Vorono noi cell ell (caveat: can be unbounded) � circumcircles and triangles � Points with more than one nearest site = Voro Vorono noi dia iagra gram CS101 - Meshing CS101 - Meshing 3 4 Example Example 2 points Points that are equidistant (bisector) circumcircle CS101 - Meshing CS101 - Meshing 5 6

Voronoi cell Voronoi Cell Definition using halfplanes � let be the halfplane: � boundary = bisector of the two sites is in the halfplane � � Then we have: � Consequence: convex cells convex cells CS101 - Meshing CS101 - Meshing 7 8 Voronoi Diagram Example Once we have defined the cell � boundary made out of edges � Voronoi edges � 2 closest sites � Voronoi edges linking vertices � Voronoi vertices � multiple closest sites � remember: cell complex… Four points are cocircular… CS101 - Meshing CS101 - Meshing 9 10 Example Voronoi Example I CS101 - Meshing CS101 - Meshing 11 12

Voronoi Example II Voronoi in Nature? CS101 - Meshing CS101 - Meshing 13 14 Voronoi Properties Some Applications Too many to mention… Motion Planning � cell always convex � what path should a robot follow to stay as far as possible from sites? � if V vertex of degree 3, circumcenter � if p j n.n. of p i they share a V-edge Ambulance Dispatcher � which hospital is closest to scene? � cell unbounded iff site on CH � proof? Farthest point sampling � think about a point at infinity � where to put a new Starbuck’s? � p on CH iff closest point from inf. point CS101 - Meshing CS101 - Meshing 15 16 Delaunay Triangulation (Rough) Examples Definition: � DT is the (straight-line) dual of VD � dual in the sense of: � V cell -> D vertex � V edge -> D edge � V vertex -> D triangle � careful: what happen when 4 cocircular points? An example from the sea… CS101 - Meshing CS101 - Meshing 17 18

Realtime Demo Example in 3D click here CS101 - Meshing CS101 - Meshing 19 20 Properties Proof? Too many… • If ab is in DT, V(a) and V(b) share an edge e. Pick x on e, and trace a circle center on x � “same” as Voronoi through a and b. if there’s a site c in or on the � e.g., if p j n.n. of p i (p i, p j ) is a D-edge circle, x would be in V(c) too! So no site inside. � important property ( Empty Circle ): •Suppose there’s an empty circle through a and b centered on a point x. x is on bisector btw a For every D-edge (p i, p j ), there’s a and b (equidistant). Now wiggle x a bit while circle through p i and p j that doesn’t keeping the circle empty—along bisector. contain ANY other vertex. Clearly, x is on a V-edge, thus ab D-edge. The reverse holds too. CS101 - Meshing CS101 - Meshing 21 22 Applications Numerical Properties Nearest neighbor search: In 2D: maximizes minimum angle � NNG ⊆ DT � stronger: best lexicographic sequence of triangle angles � i.e., if b n.n. of a, then ab is in DT � great for numerics (as detailed later) Medial Axis – generalization (grassfire) � Edelsbrunner ’87 Minimum Spanning Tree � ain’t true in 3D…. � MST is a subgraph of DT � suppose (a,b) edge of MST, and assume (a.b) NOT Delaunay; prove contradiction (use empty circle…) CS101 - Meshing CS101 - Meshing 23 24

Connection to CH Lifting Map We already saw link Lift points (x, y) to paraboloid (x, y, x 2 + y 2 ) � placement of origin irrelevant � CH of sites goes along D-edges DT = CH of 3D points But what if we think higher dim… � I.e., 2D plane and sites are projections of something 3D � great property, valid in nD CS101 - Meshing CS101 - Meshing 25 26 Proof Same for Voronoi? Equation of tangent plane at (a,b): Almost… VD = proj. of tangent envelope Shift upwards by r 2 : Intersection with paraboloid? Before… Now, imagine you have a face (p1,p2,p3) of 3D CH � other vertices are at least r 2 away, right? � thus, they project OUTSIDE of the projected circle � bingo: empty circle, thus the face maps to D-triangle. CS101 - Meshing CS101 - Meshing 27 28