MAT 129 Precalculus Chapter 11 Notes Conics Analytic Geometry, - PowerPoint PPT Presentation

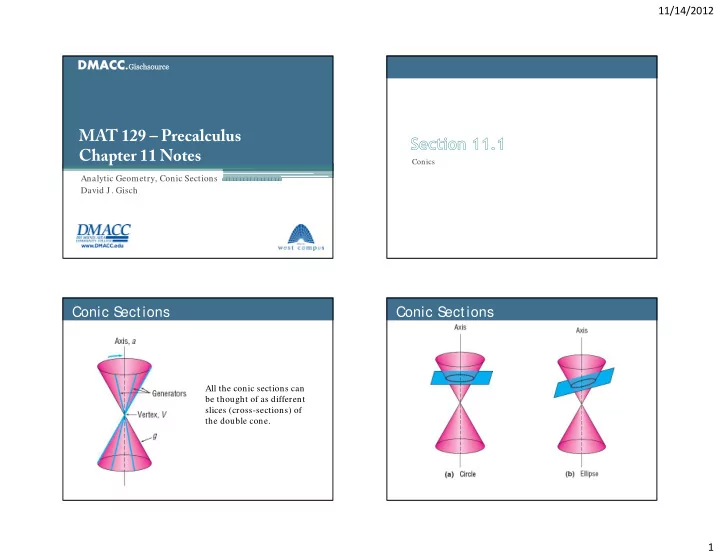
11/14/2012 MAT 129 – Precalculus Chapter 11 Notes Conics Analytic Geometry, Conic Sections David J. Gisch Conic S ections Conic S ections All the conic sections can be thought of as different slices (cross-sections) of the double cone. 1

11/14/2012 2 The Parabola The Parabola ections The Parabola Conic S

11/14/2012 The Parabola The Parabola Example 11.2.1: Find an equation of the parabola with Example 11.2.2: Find the equation of a parabola with vertex at �0, 0� and focus at �3, 0� . Graph the equation. vertex at �0, 0� and focus at �8, 0� . Graph the equation. The Parabola The Parabola Example 11.2.3: The equation of a parabola is � � � 24� . Graph the equation and find the vertex and directrix. 3

11/14/2012 The Parabola The Parabola Example 11.2.5: The equation of a parabola is � � � �8� , Example 11.2.4: Find the equation of a parabola with a focus at �0, 6� and directrix at � � �6 . Graph the equation. Graph the equation and state the focus, vertex and directrix. The Parabola The Parabola Example 11.2.6: Find the equation of a parabola with a Example 11.2.7: The equation of a parabola is � � � 4� � 4� � 0 . Graph the equation and state the focus, vertex at �4, �3� and focus �4, 0� . Graph the equation. vertex, and directrix. 4

11/14/2012 S pecial Properties of the Parabola S pecial Properties of a Parabola The Parabola Example 11.2.8 Cont. Example 11.2.8: A satellite dish measures 8 feet across at its opening and is 3 feet deep at its center. Where should the receiver be placed? 5

11/14/2012 The Ellipse An ellipse is a collection of all points in the plane, the sum of whose distances from two fixed points, called the foci, is constant. OR. For any point P in the plane and foci � � and � � the equation � � � , � � � � � , � � �, where c is a constant, must be satisfied. The Ellipse The Ellipse The Ellipse • The foci, center, and vertices on the minor axis form a right triangle. This allows you to use trig and the Pythagorean theorem to find missing values. • Also notice that the hypotenuse is half the length of the major axis and b is half the minor axis. Major horizontal axis, equation Major horizontal axis, equation � � � � � � � � � � � � � � � � � 1, ��� � � � � � � � � � � � 1, ��� � � � � � � � � 6

11/14/2012 The Parabola The Parabola Example 11.3.1: Find an equation of an ellipse centered at Example 11.3.2: Find the equation of an ellipse centered at the origin with a focus of �4, 0� and vertex at �8, 0� . Graph the origin with a major axis of length 10 and a minor axis the equation. of length 6. Graph the equation. The Parabola The Ellipse Example 11.3.3: The equation of an ellipse is � � 36 � � � 9 � 1 Find the foci, center, and vertices. Graph the ellipse. 7

11/14/2012 The Parabola The Parabola Example 11.3.4: Find an equation of an ellipse centered at Example 11.3.5: Find an equation of an ellipse centered at 2, 3 , with a focus of �2, 5� and a major axis of 6. Graph �1, 2 , with a focus of �0, 2� and a vertex at �0, 5� . Graph the equation. the equation. The Parabola S pecial Properties of the Ellipse Example 11.3.6: The equation of an ellipse is 4� � � � � � 32� � 4� � 52 � 0 Find the foci, center, and vertices. Graph the ellipse. 8

11/14/2012 The Parabola Whispering Room Cont. Example 11.3.7: The whispering gallery in the Museum of Science and Industry in Chicago is 47.3 feet long. The distance from the center of the room to the foci is 20.3 feet. Find an equation that describes the shape of the room. How high is the room at its center? The Hyperbola The Hyperbola 9

11/14/2012 S pecial Properties of Hyperbola S pecial Properties of Hyperbola General Form of Conics Polar Equations of Conics 10

11/14/2012 Polar Equations of Conics Polar Equations of Conics • All conics fail the vertical line test except for a “vertical” parabola. Thus, they are not functions in the traditional x-y plane. • However, conics are all functions when in polar form. Parametric Equations Parametric Equations We essentially break an equation into two pieces; one that models the x values, and one that models the y values. In doing so, each part is a function but when combined we can graph curves that are not normally functions, like the conics. 11

11/14/2012 The Parabola The Parabola Example 11.7.1: Graph the parametric equation Example 11.7.2: Graph the parametric equation � � � 2� � , � � � 3�, �2 � � � 2 � � � 3 cos � , � � � 3 sin � , �� � � � � � � � � � � �2 �� � � �1 2 0 0 � 1 2 2 � Uses of Parametric Equations Parametric Equations Example 11.7.3: Including her height, Mara is on a cliff that • One fun use of parametric equations is the science of projectiles. is 150 feet high. She decides to become a drunk and get heavily involved in drugs and thus throws her dreams off of the cliff at an angle of 38° and a velocity of 25 feet per second. a) Write a set of parametric equations modeling the flight of the dreams. b) Graph the equations on a TI calculator c) Determine the length of time that her dreams will be in the air. • Here we can model a projectile with the parametric equations � � � � 1 2 �� � � � � sin � � � � � � � � � cos � �, d) How far did her dreams fly horizontally? where � is the force of gravity ( 32 �� � ⁄ or 9.8 � � ⁄ ), � is the angle to e) At what time will her dreams be at their peak height the horizontal, � is the height from the “ground,” and � � is the initial and what is that height? velocity of the projectile. 12

11/14/2012 Mara’s Dreams Mara’s Dreams Parametric Equations The Bullet Example 11.7.4: An M16 machine gun has a muzzle velocity ⁄ . You are standing on level ground and shoot of 3,110 �� � a bullet with an angle of 30° with the horizontal. a) Write a set of parametric equations modeling the flight of the bullet. b) Graph the equations on a TI calculator c) Determine the length of time that the bullet will be in the air. d) How far did her bullet fly horizontally? e) At what time will the bullet be at its peak height and what is that height? 13

11/14/2012 The Bullet Angry Proj ectiles R ectangular to Parametric Equations R ectangular to Parametric Equations Example 11.7.5: Find the parametric equation of Example 11.7.6: Find the parametric equation of � � � � � 4 � � � � � 16 � 1 14

11/14/2012 Parametric Equations R ectangular to Parametric Equations Example 11.7.7: In the quintessential movie moment, you and your adversary, who are 15 feet apart, both see the object of supreme importance to completing the climax of the plot. Suddenly the lights go out. Your adversary takes off at a heading of 87° at 4 �� � ⁄ . At the same time, you take off at a heading of 97° at 4.2 �� � ⁄ . a) Write a parametric equation for each persons movement. b) Assuming you both were aiming correctly for the object, what are its coordinates? c) Who gets to the object first? And at what time? Movie Moment R ectangular to Parametric Equations Example 11.7.8: A boat leaves the port on a heading of 45° going 40 knots per hour. At the same time a another boat takes off from a port 40 knots east of the first port, at a heading of 100° at 60 knots per hour. a) Write a parametric equation for each boats movement. b) Will the boats hit each other? c) If yes, when? If not, when will they be the closest (to the nearest half hour)? 15

11/14/2012 16 hip Happens S