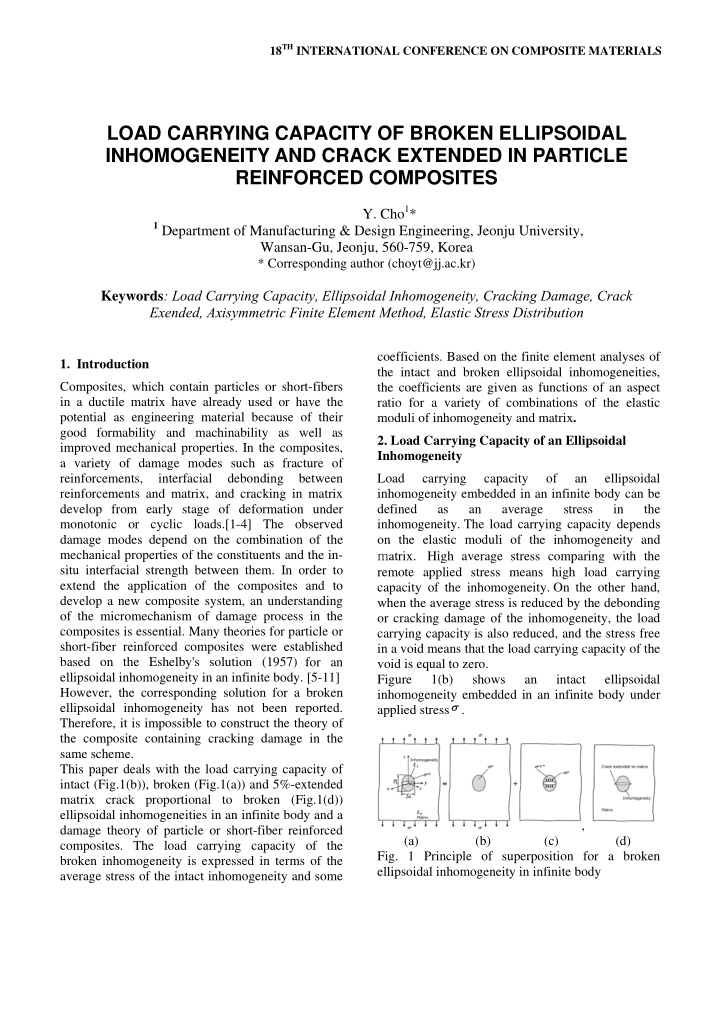



18 TH INTERNATIONAL CONFERENCE ON COMPOSITE MATERIALS LOAD CARRYING CAPACITY OF BROKEN ELLIPSOIDAL INHOMOGENEITY AND CRACK EXTENDED IN PARTICLE REINFORCED COMPOSITES Y. Cho 1 * 1 Department of Manufacturing & Design Engineering, Jeonju University, Wansan-Gu, Jeonju, 560-759, Korea * Corresponding author (choyt@jj.ac.kr) Keywords : Load Carrying Capacity, Ellipsoidal Inhomogeneity, Cracking Damage, Crack Exended, Axisymmetric Finite Element Method, Elastic Stress Distribution coefficients. Based on the finite element analyses of 1. Introduction the intact and broken ellipsoidal inhomogeneities, Composites, which contain particles or short-fibers the coefficients are given as functions of an aspect in a ductile matrix have already used or have the ratio for a variety of combinations of the elastic potential as engineering material because of their moduli of inhomogeneity and matrix . good formability and machinability as well as 2. Load Carrying Capacity of an Ellipsoidal improved mechanical properties. In the composites, Inhomogeneity a variety of damage modes such as fracture of reinforcements, interfacial debonding between Load carrying capacity of an ellipsoidal reinforcements and matrix, and cracking in matrix inhomogeneity embedded in an infinite body can be develop from early stage of deformation under defined as an average stress in the monotonic or cyclic loads.[1-4] The observed inhomogeneity. The load carrying capacity depends damage modes depend on the combination of the on the elastic moduli of the inhomogeneity and m atrix. High average stress comparing with the mechanical properties of the constituents and the in- situ interfacial strength between them. In order to remote applied stress means high load carrying extend the application of the composites and to capacity of the inhomogeneity. On the other hand, develop a new composite system, an understanding when the average stress is reduced by the debonding of the micromechanism of damage process in the or cracking damage of the inhomogeneity, the load composites is essential. Many theories for particle or carrying capacity is also reduced, and the stress free short-fiber reinforced composites were established in a void means that the load carrying capacity of the based on the Eshelby's solution (1957) for an void is equal to zero. ellipsoidal inhomogeneity in an infinite body. [5-11] Figure 1(b) shows an intact ellipsoidal However, the corresponding solution for a broken inhomogeneity embedded in an infinite body under applied stress σ . ellipsoidal inhomogeneity has not been reported. Therefore, it is impossible to construct the theory of the composite containing cracking damage in the same scheme. This paper deals with the load carrying capacity of intact (Fig.1(b)), broken (Fig.1(a)) and 5%-extended matrix crack proportional to broken (Fig.1(d)) ellipsoidal inhomogeneities in an infinite body and a , damage theory of particle or short-fiber reinforced (a) (b) (c) (d) composites. The load carrying capacity of the Fig. 1 Principle of superposition for a broken broken inhomogeneity is expressed in terms of the ellipsoidal inhomogeneity in infinite body average stress of the intact inhomogeneity and some
* due to internal stress * , cp cp cp The elastic stiffness tensor of the infinite body Fig.1(c) are σ σ * and σ z x y L − p τ cp * − τ p σ on the crack surface, due to , and (matrix) and the inhomogeneity are denoted by z yz yz 0 τ − τ . Therefore, we have cp * p due to L , respectively. The stress of the ellipsoidal zx zx and 1 ⎡ ⎤ h 1 0 0 0 0 σ p 13 ⎢ ⎥ inhomogeneity is uniform and given by the h 0 1 0 0 0 ⎢ ⎥ 13 + = + = h Eshelby's equivalent inclusion method as well 0 0 1 0 0 0 (3) k ( 1 h ) ⎢ ⎥ 33 + h ⎢ 0 0 0 1 0 0 ⎥ known. [1] 44 ⎢ + ⎥ h 0 0 0 0 1 0 σ = − − + − − − σ p 1 1 44 L ( S I )[( L L ) S L ] L ( S I ) L ⎢ ⎥ (1) ⎣ 0 0 0 0 0 1 ⎦ 0 1 0 0 1 0 Where, S is Eshelby's tensor which is expressed as a in the matrix form. Furthermore, in the case of the h = h function of shape of the inhomogeneity and axisymmetric ellipsoidal inhomogeneity, and 13 23 Possino's ratio of the matrix. = h h relations are obtained. As a result, once the 44 55 For an ellipsoidal inhomogeneity cracked in the three components, h 33 , h 13 and h 44 are obtained, the cross section of xy -plane as shown in Fig. 1(a), the average stress of the broken ellipsoidal stress distribution in the inhomogeneity seems to be inhomogeneity can be evaluated by Eqs.(1) and (2). complex and its solution have not been reported as Out of the three components, h 33 and h 13 are far as the authors traced references. Figure 1 shows determined by the analysis under uniaxial tension, the principle of superposition for a cracked and h 44 is by the analysis under pure shear. ellipsoidal inhomogeneity in an infinite body. The average stress in the inhomogeneity represents 3. Numerical Procedure its load carrying capacity, and the difference Elastic stress analyses of intact and damaged between the average stresses of the intact and broken ellipsoidal inhomogeneities embedded in an infinite inhomogeneities indicates the loss of load carrying body under uniaxial tension as shown in Fig.1 were capacity due to the cracking damage. As shown in carried out based on the axisymmetric finite element Fig. 1(a), the stress state in the broken method using the quadrilateral 8-node isoparametric σ cp inhomogeneity is given by the sum of the elements. Mesh division was carried out for a wide σ σ σ p p cp * stresses and , where the stress is in the ellipsoidal domain including an inhomogeneity in σ and p the center, and uniaxial uniform tensile stress in the intact inhomogeneity under the applied stress z -axis direction is applied on the surface of the σ cp * is the broken inhomogeneity subjected to domain as boundary condition. The size of the − σ p internal stress on the crack surface. Therefore, inhomogeneity is denoted by 2 a and 2 b in r ( x and y ) the average stress of the broken inhomogeneity is direction and z direction, respectively. On the same expressed by finite element meshes, the node points on the z - and σ = σ + σ = σ + σ = + σ = σ cp p cp * p p p p h ( I h ) k (2) r -axes were fixed for the intact inhomogeneity, and Where h is a coefficient expressing the reduction of the node points on the z - and r -axes except crack average stress due to the cracking damage of an plane were fixed for the cracked inhomogeneity. In ellipsoidal inhomogeneity, k is the ratio of the the analyses, an aspect ratio ( b/a ) of the average stresses of the broken and intact inhomogeneity and the combination in the elastic inhomogeneities and I was unit tensor. Once h is the moduli of the inhomogeneity and matrix were determined, the load carrying capacity of the broken widely changed. The average stress in the intact and inhomogeneity can be evaluated inclusion method. cracked inhomogeneities were calculated and used Based on the axisymmetric finite element analyses as the load carrying capacity. [12] of the intact and broken ellipsoidal inhomogeneities, the matrix h is obtained as a function of an aspect 4. Numerical Results and Discussion ratio the inhomogeneity and combination of elastic moduli of the inhomogeneity and matrix. [12] 4.1 Stress distribution in and around an The components of the stresses are given inhomogeneity ⎣ ⎦ by , ⎣ ⎦ and σ = σ p σ p σ p τ p τ p τ p p σ = σ cp σ cp σ cp τ cp τ cp τ cp Stress distributions of the intact and cracked , , , , , cp , , , , , x y z yz zx xy x y z yz zx xy ⎣ ⎦ inhomogeneities are shown for the case in which σ ∗ σ ∗ σ ∗ τ ∗ τ ∗ τ ∗ . The average stress σ ∗ = cp cp cp cp cp cp cp , , , , , x y z yz zx xy Young’s modulus ratio is E p /E m =5.0 and Poisson’s components in the broken inhomogeneity shown in
Recommend
More recommend