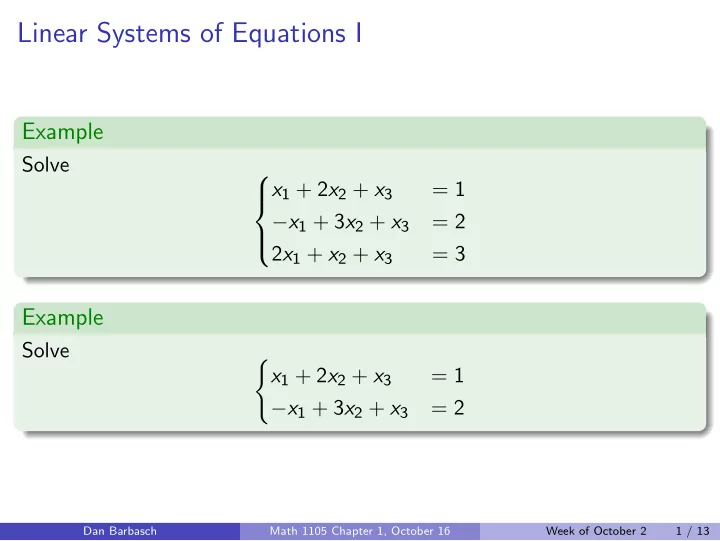
Linear Systems of Equations I Example Solve x 1 + 2 x 2 + x 3 = - PowerPoint PPT Presentation

Linear Systems of Equations I Example Solve  x 1 + 2 x 2 + x 3 = 1   − x 1 + 3 x 2 + x 3 = 2  2 x 1 + x 2 + x 3 = 3  Example Solve � x 1 + 2 x 2 + x 3 = 1 − x 1 + 3 x 2 + x 3 = 2 Dan Barbasch Math 1105 Chapter 1, October 16 Week of October 2 1 / 13

Linear Systems of Equations II Exercise Solve  − x + y = 1   3 x − 2 y = 2  2 x − y = − 2  Find the triangle determined by the three lines in the linear system. Dan Barbasch Math 1105 Chapter 1, October 16 Week of October 2 2 / 13

Answer Continued I Answer. Instead of carrying along the x , y we just record the coefficients. This is called the Gauss-Jordan Method. − 1 1 | 1 3 − 2 | 2 2 − 1 | − 2 1 Add 3 tinmes row one to row 2 2 2 times row one to row 3. − 1 1 | 1 0 1 | 5 0 1 | 0 Dan Barbasch Math 1105 Chapter 1, October 16 Week of October 2 3 / 13

Answer Continued II Answer. 1 subtract row two from rows one and rows three − 1 0 | − 4 0 1 | 5 0 0 | − 5 The system has NO solution. The first equation is x = 4 the second y = 5 and the third 0 = − 5. Graphically the three equations are three lines. To have a solution they must meet in a single point. They clearly don’t. You can find the intersections of each two of the three lines by solving the systems � � � − x + y = 1 − x + y = 1 2 x − y = 2 3 x − 2 y = 2 2 x − y = − 2 3 x − 2 y = − 2 Dan Barbasch Math 1105 Chapter 1, October 16 Week of October 2 4 / 13

Another Example I Example 2 3 − 4 | 5 Convert into a system of equations. Solve the system. 1 − 1 2 | 6 Dan Barbasch Math 1105 Chapter 1, October 16 Week of October 2 5 / 13

Another Example II Answer. � 2 x − 4 y + 5 z = 5 x − y + 2 z = 6 � 1 � − 1 2 6 1 Interchange the two equations: 2 − 4 5 5 2 Add ( − 2) × (row one) to (row two); the pivot is the 1 corresponding � 1 � − 1 2 6 to x : 0 − 2 1 − 7 3 Divide (row two) by − 2 and add to (ow one); the pivot is the 1 � 1 � � 1 � − 1 2 6 0 3 / 2 19 / 2 corresponding to y : 0 1 − 1 / 2 7 / 2 0 1 1 / 2 7 / 2 Dan Barbasch Math 1105 Chapter 1, October 16 Week of October 2 6 / 13

Answer Continued There are no rows left to use. Interpret the result: x = 19 − 3 z y = 7 − z . 2 2 There are infinitely many real solutions. You can choose z arbitrary, then x , y are determined by the formulas above. Exercise Do the same exercise using z as the pivot in equation 2. You need not divide by ( − 2) any more, and the arithmetic is easier. See your notes from class. The answer should be x , z in terms of y . Dan Barbasch Math 1105 Chapter 1, October 16 Week of October 2 7 / 13

Application Exercise (67 in 2.3, Toys) One hundred toys are to be given out to a group of children. A ball costs $2, a doll costs $3, and a car costs $4. A total of $295 was spent on the toys. a. A ball weighs 12 oz, a doll 16 oz, and a car 18 oz. The total weight of all the toys is 1542 oz. Find how many of each toy there are. b. Now suppose the weight of a ball, doll, and car are 11, 15, and 19 oz, respectively. If the total weight is still 1542 oz, how many solutions are there now? c. Keep the weights as in part b, but change the total weight to 1480 oz. How many solutions are there? d. Give the solution to part c that has the smallest number of cars. e. . Give the solution to part c that has the largest number of cars. Dan Barbasch Math 1105 Chapter 1, October 16 Week of October 2 8 / 13

Matrices Definition A matrix is a tableau with m rows and n columns, each entry a number. There are operations you can perform on matrices of the same size: 1 addition 2 Multiplication by a scalar. There is a multiplication operation which is more complicated. Dan Barbasch Math 1105 Chapter 1, October 16 Week of October 2 9 / 13

Exercise 48 in 2.3 trends. 48. 46. Educational Attainment The table below gives the educa- tional attainment of the U.S. population 25 years and older since 1970. Source: U. S. Census Bureau. Male Female Percentage Percentage Percentage Percentage with 4 with 4 with 4 with 4 Years of Years of Years of Years of High School College High School College Year or More or More or More or More 1970 55.0 14.1 55.4 8.2 1980 69.2 20.9 68.1 13.6 1990 77.7 24.4 77.5 18.4 2000 84.2 27.8 84.0 23.6 2008 85.9 30.1 87.2 28.8 a. Write a matrix for the educational attainment of males. b. Write a matrix for the educational attainment of females. c. Use the matrices from parts a and b to write a matrix show- ing the difference in educational attainment between males and females since 1970. Dan Barbasch Math 1105 Chapter 1, October 16 Week of October 2 10 / 13

Management, 40 in 2.3 Exercise There are three convenience stores in Folsom. This week, store I sold 88 loaves of bread, 48 qt of milk, 16 jars of peanut butter, and 112 lb of cold cuts. Store II sold 105 loaves of bread, 72 qt of milk, 21 jars of peanut butter, and 147 lb of cold cuts. Store III sold 60 loaves of bread, 40 qt of milk, no peanut butter, and 50 lb of cold cuts. a. Use a 4 × 3 matrix to express the sales information for the three stores. b. During the following week, sales on these products at all stores increased by 25%; Write the sales matrix for that week. c. During the following week, sales on these products at store I increased by 25%; sales at store II increased by 1 / 3 ; and sales at store III increased by 10%. Write the sales matrix. d. Write a matrix that represents total sales over the two-week period. Dan Barbasch Math 1105 Chapter 1, October 16 Week of October 2 11 / 13

Matrix Multiplication Example (Cost Analysis, 45 in 2.4) The Mundo Candy Company makes three types of chocolate candy: Cheery Cherry, Mucho Mocha, and Almond Delight. The company produces its products in San Diego, Mexico City, and Managua using two main ingredients: chocolate and sugar. a. Each kilogram of Cheery Cherry requires 0.5kg of sugar and 0.2kg of chocolate; each kilogram of Mucho Mocha requires 0.4kg of sugar and 0.3kg of chocolate; and each kilogram of Almond Delight requires 0.3kg of sugar and 0.3kg of chocolate. Put this information into a 2 × 3 matrix called A , labeling the rows and columns. b. The cost of 1kg of sugar is $4 in San Diego, $2 in Mexico City, and $1 in Managua. The cost of 1kg of chocolate is $3 in San Diego, $5 in Mexico City, and $7 in Managua. Put this information into a matrix called B . c. Write a matrix C which represents the ingredient cost of producing each type of candy in each city. Dan Barbasch Math 1105 Chapter 1, October 16 Week of October 2 12 / 13

EXERCISE Exercise How much does it cost to produce CC in Mexico City? What about the other cities? COST = (c of S) × (amt of S) + (c of C) × (amt of C) CC in MC: 2 × 0 . 5 + 5 × 0 . 2 . How do we organize the calculation so we get all the answers at once? Dan Barbasch Math 1105 Chapter 1, October 16 Week of October 2 13 / 13

ANSWER Multiplication of matrices: cS cC CC MM AD SD 4 3 aS 0 . 5 0 . 4 0 . 3 MC 2 5 aC 0 . 2 0 . 3 0 . 3 M 1 7 CC MM AD SD 4 · 0 . 5 + 3 · 0 . 2 4 · 0 . 4 + 3 · 0 . 3 4 · 0 . 3 + 3 · 0 . 3 MC 2 · 0 . 5 + 5 · 0 . 2 2 · 0 . 4 + 5 · 0 . 3 2 · 0 . 3 + 5 · 0 . 3 M 1 · 0 . 5 + 7 · 0 . 2 1 · 0 . 4 + 7 · 0 . 3 1 · 0 . 3 + 7 · 0 . 3 Dan Barbasch Math 1105 Chapter 1, October 16 Week of October 2 14 / 13