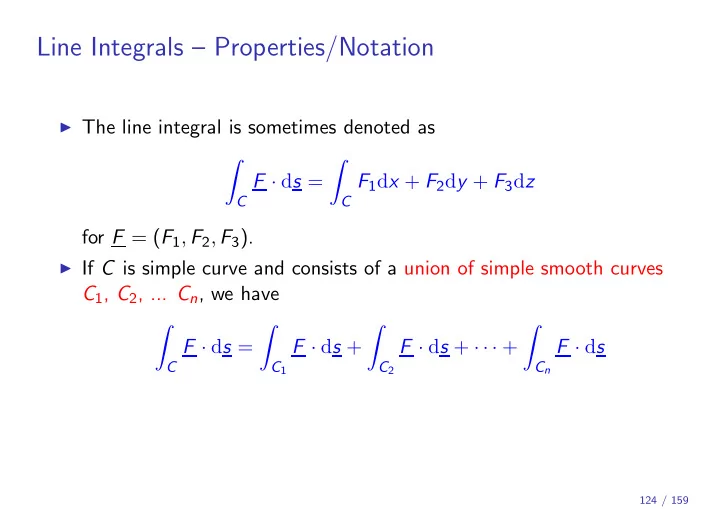
Line Integrals Properties/Notation The line integral is sometimes - PowerPoint PPT Presentation

Line Integrals – Properties/Notation ◮ The line integral is sometimes denoted as � � F · d s = F 1 d x + F 2 d y + F 3 d z C C for F = ( F 1 , F 2 , F 3 ). ◮ If C is simple curve and consists of a union of simple smooth curves C 1 , C 2 , ... C n , we have � � � � F · d s = F · d s + F · d s + · · · + F · d s C C 1 C 2 C n 124 / 159

Line Integral of a Gradient If f is differentiable a scalar function and C is a smooth curve then � ∇ f · d s = f ( r ( b )) − f ( r ( a )) C A vector field F such that F = ∇ f for some differentiable scalar function f , is called conservative vector field and the function f is called potential of F . Example: The gravitational field of a mass M , reads g = − GM where r = ( x , y , z ) r 3 r is conservative, as the function V = GM r is a potential of g . 125 / 159

Line Integral of Conservative Fields Let F = ∇ f conservative field. The identity � ∇ f · d s = f ( r ( b )) − f ( r ( a )) C highlights the following: BIG IDEA: The value of the line integral of a conservative vector field is independent of the path C ! It only depends on the value of the potential at the initial and final points. If r ( a ) = r ( b ) then � ∇ f · d s = 0 C for any simple smooth curve C . 126 / 159

Parametrisation of surfaces A simple curve in space can be parameterised by a vector function of one variable r ( t ) = ( x ( t ) , y ( t ) , z ( t )) a ≤ t ≤ b . How do we parameterise a surface? Consider the sphere of radius 1 centered at the origin. In spherical coordinates it can be described as r = 1 , 0 ≤ θ < 2 π, 0 ≤ φ ≤ π or (formally) as r ( θ, φ ) = (cos θ sin φ, sin θ sin φ, cos φ ) BIG IDEA: A (simple & smooth) surface can be parameterised by a vector function of 2 variables! 127 / 159

Parametrisation of surfaces Thus, in general, a surface S can be parameterised as r ( u , v ) = ( x ( u , v ) , y ( u , v ) , z ( u , v )) , a ≤ u ≤ b , c ≤ v ≤ d . Example: What is a parametrisation of a cylinder of radius 2 and height 2, whose lower basis lies in the xy -plane? Answer: Cylindrical Coordinates: r ( u , v ) = (2 cos u , 2 sin u , v ) 0 ≤ u < 2 π and 0 ≤ v ≤ 2 128 / 159