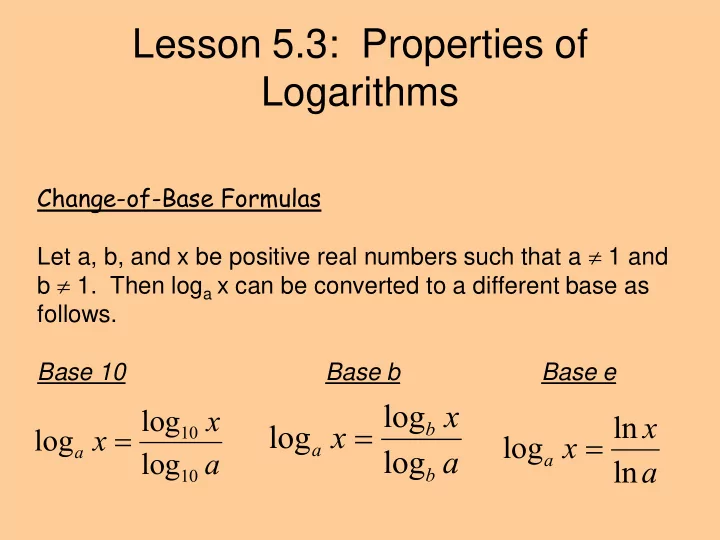
Lesson 5.3: Properties of Logarithms Change-of-Base Formulas Let - PowerPoint PPT Presentation

Lesson 5.3: Properties of Logarithms Change-of-Base Formulas Let a, b, and x be positive real numbers such that a  1 and b  1. Then log a x can be converted to a different base as follows. Base 10 Base b Base e log x log x ln x  log x b  10 log a x  log a x a log a log a ln a b 10

Ex 1: Rewrite each log as a ratio of common logs and natural logs.  ln 30  log 30 log 4 30 10 A. log 4 ln 4 10  log 17  ln 17 10 B. log 2 17 log 2 ln 2 10  ln x  log x log 6 x 10 C. ln 6 log 10 6

Properties of Logarithms Let a be a positive number such that a  1, and let n be a real number. If u and v are positive real numbers, the following properties are true. Logs with base a 1.Log a (uv) = log a u + log a v Need to be the same! 2.Log a u/v = log a u – log a v 3. Log a u n = n log a u

Properties of Logarithms Let a be a positive number such that a  1, and let n be a real number. If u and v are positive real numbers, the following properties are true. Natural Logs 1. ln (uv) = ln u + ln v 2. ln u/v = ln u – ln v 3. ln u n = n ln u

Ex 2: Write each logarithm in terms of ln 2 and ln 3 a f     ln 2 3 ln 2 ln 3 A. ln 6  F H I  F H I 2 ln 2 K K ln B. ln 2/27 3 3 27   3 3 ln 2 ln   ln 2 3 ln 3

Ex 3: Expand each logarithmic expression.    3 log 5 log x log y A. log 4 5x 3 y 4 4 4    log 5 3 log x log y 4 4 4 x  3 5    ln 3 x 5 ln 7 ln B. 7 a f 1    ln 3 x 5 ln 7 2 a f 1    ln 3 x 5 ln 7 2

Ex 4: Condense each logarithmic expression. a f 1   A. log x 3 log x 1 10 10 2 1 a f 3    log x log x 1 2 10 10 a f 3    log x log x 1 10 10 a f 3   log 10 x x 1

Ex 4: Condense each logarithmic expression. b g   B. 2 ln x 2 ln x a f 2    ln x 2 ln x a f 2  ln x 2  x

Ex 4: Condense each logarithmic expression. b g 1   log x log x 1 C. 2 2 3 a f l q 1   log x x 1 2 3 a f a f 1     log 2 x x 1 log 2 x x 1 3 3 Homework: p.393 #3-15, 39-78 all mult. of 3