Lecture 10: Discretionary policy and time-inconsistency of monetary - PDF document

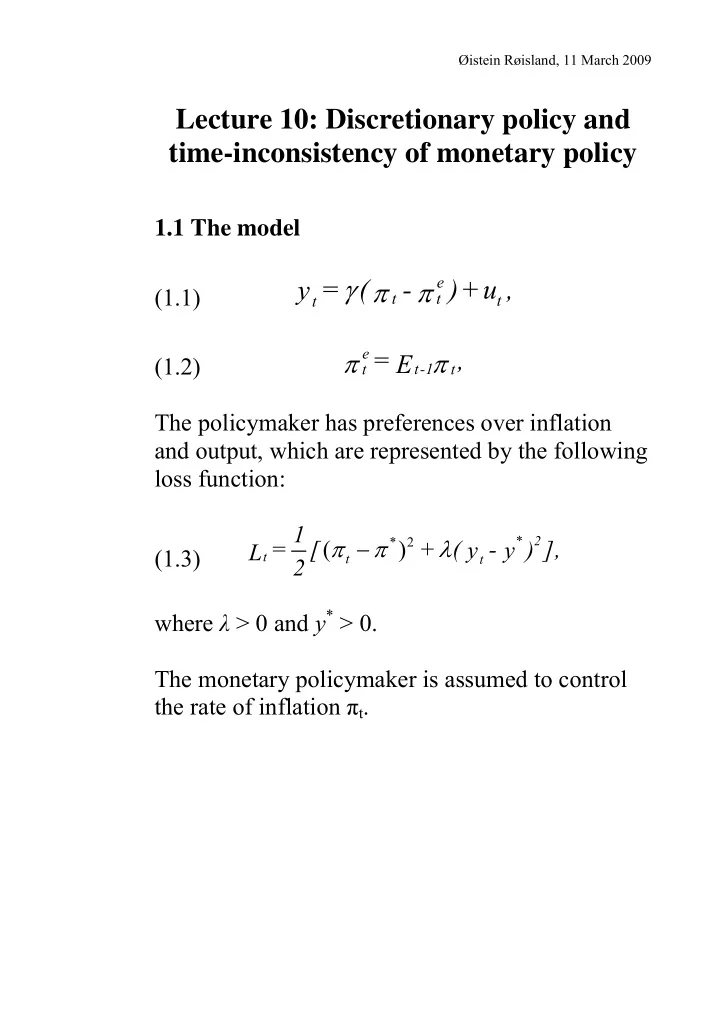
Øistein Røisland, 11 March 2009 Lecture 10: Discretionary policy and time-inconsistency of monetary policy 1.1 The model γ π e y t = ( - π ) + u , (1.1) t t t π e = π , E (1.2) t t-1 t The policymaker has preferences over inflation and output, which are represented by the following loss function: 1 π − π λ * 2 * 2 = [ ( ) + ( y - y ) ], L (1.3) t t t 2 where λ > 0 and y * > 0. The monetary policymaker is assumed to control the rate of inflation π t .

Discretionary policy The discretionary solution can be found by minimizing L t with respect to π t and subject to (1.1) and (1.2). This results in the following first order condition: π − π + γλ − = * * ( ) ( y y ) 0 (1.4) t t This gives the following solutions for inflation and output: λγ π + λγ * * π = - u , y (1.5) t t λγ 2 1+ 1 y = u . t t 1+ λγ 2 (1.6) Commitment Solution under commitment found by minimizing e , which gives the L t with respect to both π t and π t following first-order conditions π − π + γλ − + θ − = * * ( ) ( y y ) 0 (1.7) t t t 1 − γλ − − θ = * E [ ( y y )] 0 (1.8) − − t 1 t t 1 2

where θ − is the Lagrange multiplier corresponding t 1 to (1.2). These give the following outcome: λγ π * π = - u , (1.9) t t λγ 2 1+ 1 y t = u . (1.10) t + λγ 2 1 [An alternative way derive the optimal policy under commitment, is to assume that the central bank commits to an “inflation rule” of the form π = − a bu (1.11) t t Inserting this in (1.3), making use of (1.1) and (1.2), and minimising with respect to a and b gives λγ = π = + * a , b . λγ 2 1 3

Solutions to the time-inconsistency problem A. Reputation • "trigger strategy o . Barro and Gordon (1983b). • uncertainty about the type of central bank o Backus and Driffil (1985 B. Delegation As a compromise between credibility and flexibility, Rogoff (1985) suggested that the government should appoint a "conservative" central banker, that is, a central banker with the following preferences: 1 π − π * 2 * 2 cb cb = [ ( ) + λ ( y - y ) ], L (1.12) t t t 2 where λ cb is the central banker's subjective weight attached to output stability, λ cb < λ , C. Optimal contracts • Walsh (1995) and Persson and Tabellini (1993) 4

(1.13) 1 π − π λ * 2 π * 2 cb = [ ( ) + ( - ] + c , y y ) L t t t t 2 • Svensson (1997) 1 2 * 2 λ = [( π - π g + ( - ] ) y y ) L t t (1.14) t 2 Inflation in Norway and USA 16 14 Devaluation May 1986 12 Inflation 10 Norway 8 6 4 Inflation USA 2 Volcker 0 1979-1987 -2 1970 1975 1980 1985 1990 1995 2000 2005 5