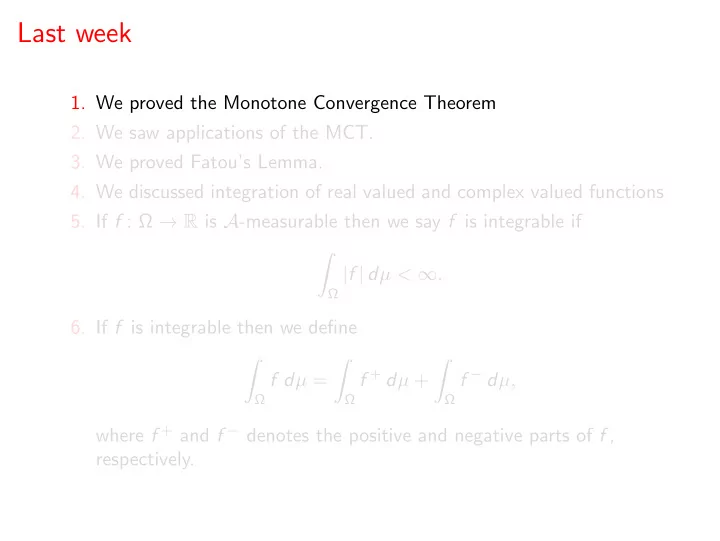
Last week 1. We proved the Monotone Convergence Theorem 2. We saw - PowerPoint PPT Presentation

Last week 1. We proved the Monotone Convergence Theorem 2. We saw applications of the MCT. 3. We proved Fatou’s Lemma. 4. We discussed integration of real valued and complex valued functions 5. If f : Ω → R is A -measurable then we say f is integrable if � | f | d µ < ∞ . Ω 6. If f is integrable then we define � � � f + d µ + f − d µ, f d µ = Ω Ω Ω where f + and f − denotes the positive and negative parts of f , respectively.

Last week 1. We proved the Monotone Convergence Theorem 2. We saw applications of the MCT. 3. We proved Fatou’s Lemma. 4. We discussed integration of real valued and complex valued functions 5. If f : Ω → R is A -measurable then we say f is integrable if � | f | d µ < ∞ . Ω 6. If f is integrable then we define � � � f + d µ + f − d µ, f d µ = Ω Ω Ω where f + and f − denotes the positive and negative parts of f , respectively.

Last week 1. We proved the Monotone Convergence Theorem 2. We saw applications of the MCT. 3. We proved Fatou’s Lemma. 4. We discussed integration of real valued and complex valued functions 5. If f : Ω → R is A -measurable then we say f is integrable if � | f | d µ < ∞ . Ω 6. If f is integrable then we define � � � f + d µ + f − d µ, f d µ = Ω Ω Ω where f + and f − denotes the positive and negative parts of f , respectively.

Last week 1. We proved the Monotone Convergence Theorem 2. We saw applications of the MCT. 3. We proved Fatou’s Lemma. 4. We discussed integration of real valued and complex valued functions 5. If f : Ω → R is A -measurable then we say f is integrable if � | f | d µ < ∞ . Ω 6. If f is integrable then we define � � � f + d µ + f − d µ, f d µ = Ω Ω Ω where f + and f − denotes the positive and negative parts of f , respectively.

Last week 1. We proved the Monotone Convergence Theorem 2. We saw applications of the MCT. 3. We proved Fatou’s Lemma. 4. We discussed integration of real valued and complex valued functions 5. If f : Ω → R is A -measurable then we say f is integrable if � | f | d µ < ∞ . Ω 6. If f is integrable then we define � � � f + d µ + f − d µ, f d µ = Ω Ω Ω where f + and f − denotes the positive and negative parts of f , respectively.

Last week 1. We proved the Monotone Convergence Theorem 2. We saw applications of the MCT. 3. We proved Fatou’s Lemma. 4. We discussed integration of real valued and complex valued functions 5. If f : Ω → R is A -measurable then we say f is integrable if � | f | d µ < ∞ . Ω 6. If f is integrable then we define � � � f + d µ + f − d µ, f d µ = Ω Ω Ω where f + and f − denotes the positive and negative parts of f , respectively.

Last week 1. We proved the Monotone Convergence Theorem 2. We saw applications of the MCT. 3. We proved Fatou’s Lemma. 4. We discussed integration of real valued and complex valued functions 5. If f : Ω → R is A -measurable then we say f is integrable if � | f | d µ < ∞ . Ω 6. If f is integrable then we define � � � f + d µ + f − d µ, f d µ = Ω Ω Ω where f + and f − denotes the positive and negative parts of f , respectively.

Today 1. We will prove the Dominated Convergence Theorem. 2. We will prove that if f : [ a , b ] → C is continuous, then the Riemann and Lebesgue integrals of f agree, in symbols: � b � f ( x ) dx = f d µ. a [ a , b ] 3. We will look at some computational examples.

Today 1. We will prove the Dominated Convergence Theorem. 2. We will prove that if f : [ a , b ] → C is continuous, then the Riemann and Lebesgue integrals of f agree, in symbols: � b � f ( x ) dx = f d µ. a [ a , b ] 3. We will look at some computational examples.

Today 1. We will prove the Dominated Convergence Theorem. 2. We will prove that if f : [ a , b ] → C is continuous, then the Riemann and Lebesgue integrals of f agree, in symbols: � b � f ( x ) dx = f d µ. a [ a , b ] 3. We will look at some computational examples.