Last Lecture: Review EEE118: Electronic Devices and Circuits 1 - PDF document

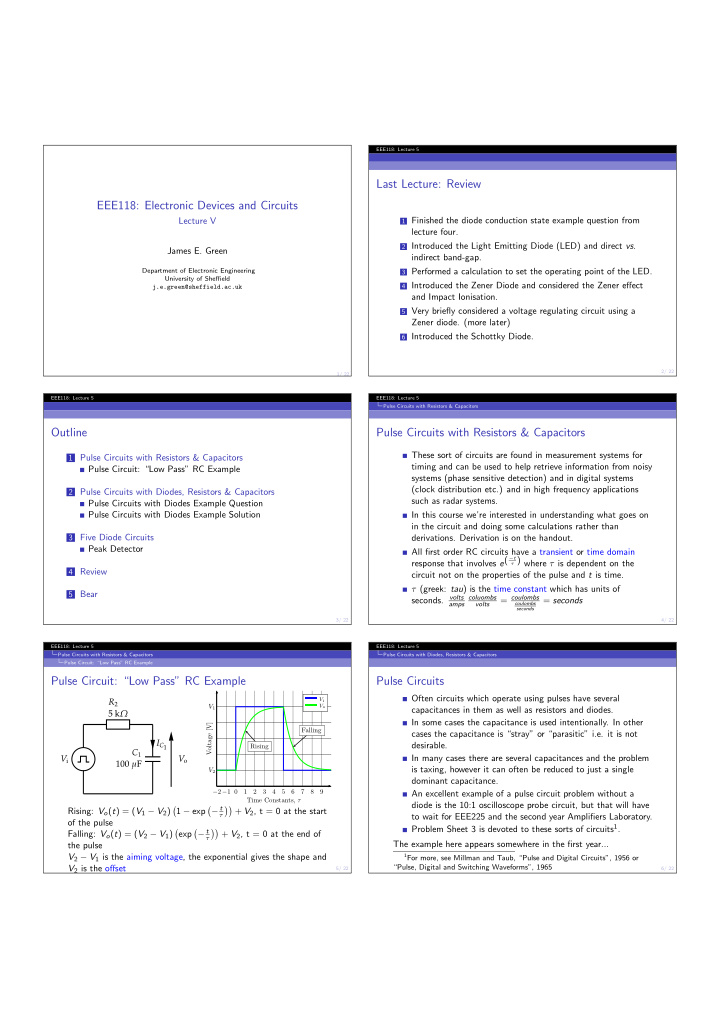
EEE118: Lecture 5 Last Lecture: Review EEE118: Electronic Devices and Circuits 1 Finished the diode conduction state example question from Lecture V lecture four. 2 Introduced the Light Emitting Diode (LED) and direct vs. James E. Green indirect band-gap. Department of Electronic Engineering 3 Performed a calculation to set the operating point of the LED. University of Sheffield 4 Introduced the Zener Diode and considered the Zener effect j.e.green@sheffield.ac.uk and Impact Ionisation. 5 Very briefly considered a voltage regulating circuit using a Zener diode. (more later) 6 Introduced the Schottky Diode. 2/ 22 1/ 22 EEE118: Lecture 5 EEE118: Lecture 5 Pulse Circuits with Resistors & Capacitors Outline Pulse Circuits with Resistors & Capacitors These sort of circuits are found in measurement systems for 1 Pulse Circuits with Resistors & Capacitors timing and can be used to help retrieve information from noisy Pulse Circuit: “Low Pass” RC Example systems (phase sensitive detection) and in digital systems (clock distribution etc.) and in high frequency applications 2 Pulse Circuits with Diodes, Resistors & Capacitors such as radar systems. Pulse Circuits with Diodes Example Question Pulse Circuits with Diodes Example Solution In this course we’re interested in understanding what goes on in the circuit and doing some calculations rather than 3 Five Diode Circuits derivations. Derivation is on the handout. Peak Detector All first order RC circuits have a transient or time domain response that involves e ( − t τ ) where τ is dependent on the 4 Review circuit not on the properties of the pulse and t is time. τ (greek: tau ) is the time constant which has units of 5 Bear volts coluombs = coulombs seconds. = seconds amps volts coulombs seconds 3/ 22 4/ 22 EEE118: Lecture 5 EEE118: Lecture 5 Pulse Circuits with Resistors & Capacitors Pulse Circuits with Diodes, Resistors & Capacitors Pulse Circuit: “Low Pass” RC Example Pulse Circuit: “Low Pass” RC Example Pulse Circuits Often circuits which operate using pulses have several V i R 2 V 1 V o capacitances in them as well as resistors and diodes. 5 k Ω In some cases the capacitance is used intentionally. In other Voltage [V] Falling cases the capacitance is “stray” or “parasitic” i.e. it is not I C 1 desirable. Rising C 1 In many cases there are several capacitances and the problem V i V o 100 µ F is taxing, however it can often be reduced to just a single V 2 dominant capacitance. − 2 − 1 0 1 2 3 4 5 6 7 8 9 An excellent example of a pulse circuit problem without a Time Constants, τ diode is the 10:1 oscilloscope probe circuit, but that will have − t � � �� Rising: V o ( t ) = ( V 1 − V 2 ) 1 − exp + V 2 , t = 0 at the start τ to wait for EEE225 and the second year Amplifiers Laboratory. of the pulse Problem Sheet 3 is devoted to these sorts of circuits 1 . − t � � �� Falling: V o ( t ) = ( V 2 − V 1 ) exp + V 2 , t = 0 at the end of τ The example here appears somewhere in the first year... the pulse V 2 − V 1 is the aiming voltage, the exponential gives the shape and 1 For more, see Millman and Taub, “Pulse and Digital Circuits”, 1956 or V 2 is the offset “Pulse, Digital and Switching Waveforms”, 1965 5/ 22 6/ 22

EEE118: Lecture 5 EEE118: Lecture 5 Pulse Circuits with Diodes, Resistors & Capacitors Pulse Circuits with Diodes, Resistors & Capacitors Pulse Circuits with Diodes Example Question Pulse Circuits with Diodes Example Solution The input, V i , is a single 0 to 10 V pulse of 5 seconds duration. A Pulse Circuit Example graph may or may not be provided so it’s a good idea to learn how R 2 to interpret a description of the waveform. D 1 5 k Ω 11 10 Input Voltage, V in 9 8 I C 1 7 C 1 R 1 V i V o 6 100 µ F 20 k Ω Voltage [V] 5 4 3 2 1 Assumptions: 0 When D 1 is conducting it has 0 V across it ( not 0.7 V) − 1 D 1 has no series resistance. − 2 − 3 C 1 is initially discharged so V o is initially 0 V, unless the − 3 − 2 − 1 0 1 2 3 4 5 6 7 8 9 10 11 12 13 14 question says otherwise. Time, t [s] 7/ 22 8/ 22 EEE118: Lecture 5 EEE118: Lecture 5 Pulse Circuits with Diodes, Resistors & Capacitors Pulse Circuits with Diodes, Resistors & Capacitors Pulse Circuits with Diodes Example Solution Pulse Circuits with Diodes Example Solution 11 2 . 2 Description of Operation 10 Input Voltage, V in 2 . 0 Capacitor Current, I C 9 1 . 8 t ≥ 0 & t ≤ 5 seconds Output Voltage, V o I pk = V in 8 1 . 6 Capacitor Current, I C [mA] R 2 V in = 10 V, the diode is forward biassed. C 1 is charged by V in 7 1 . 4 6 1 . 2 through R 2 causing V o to rise exponentially towards a maximum or Voltage [V] 5 1 . 0 aiming voltage. I C 1 is initially a maximum value at t = 0, it then 4 0 . 8 falls exponentially towards zero. Some of the current in R 2 flows in 3 0 . 6 R 1 complicating the problem! 2 0 . 4 1 0 . 2 0 0 t > 5 seconds − 1 � � − t �� − 0 . 2 V o = A 1 1 − exp The pulse has ended, V in = 0. The capacitor is now the source of − 2 τ 1 − 0 . 4 − 3 − 0 . 6 energy in the circuit. The diode is reverse biased. It stops − 3 − 2 − 1 0 1 2 3 4 5 6 7 8 9 10 11 12 13 14 conducting when the current in it falls to zero at t = 5 s. The Time, t [s] capacitor can not discharge through R 2 because the diode is high A 1 is 8 V in this case and I pk is 2 mA. τ 1 the time constant is impedance. C 1 discharges through R 1 only. Because R 1 is larger determined by the components involved τ 1 = ( R 2 // R 1 ) C 1 . The than R 2 // R 1 we should expect C 1 to take longer to discharge than expressions for exponential rise to maximum and exponential decay to charge. are derived in the handout. 9/ 22 10/ 22 EEE118: Lecture 5 EEE118: Lecture 5 Pulse Circuits with Diodes, Resistors & Capacitors Pulse Circuits with Diodes, Resistors & Capacitors Pulse Circuits with Diodes Example Solution Pulse Circuits with Diodes Example Solution t ≥ 0 & t ≤ 5 seconds 11 2 . 2 10 Input Voltage, V in 2 . 0 Capacitor Current, I C C 1 charges up from V in , current flows from V in through R 2 9 1 . 8 Output Voltage, V o I pk = V in 8 1 . 6 Capacitor Current, I C [mA] into C 1 and R 1 R 2 7 1 . 4 V o rises exponentially towards a maximum. � − t � 6 V o = A 2 exp 1 . 2 τ 2 Voltage [V] I C starts at a maximum and falls exponentially as the voltage 5 1 . 0 across R 2 falls due to the voltage across C 1 getting larger. 4 0 . 8 3 0 . 6 Since C 1 is initially (at t = 0) discharged, V o = 0 V so the 2 0 . 4 biggest value of I C 1 is at t = 0 and is given by Ohm’s law 1 0 . 2 V in − V o 0 0 . R 2 − 1 � � − t �� I pk = V o − 0 . 2 V o = A 1 1 − exp The aiming voltage of V o is the voltage that would exist − 2 τ 1 R 1 − 0 . 4 − 3 − 0 . 6 across the capacitor if it had been charged up for a long time − 3 − 2 − 1 0 1 2 3 4 5 6 7 8 9 10 11 12 13 14 and the voltages and currents had reached a steady state. In Time, t [s] this case the capacitor can not charge to a voltage greater I C changes direction, hence negative values. τ 2 is larger than τ 1 . than the potential division of V in by R 2 and R 1 . The negative peak current is given by Ohm’s law using the voltage R 1 A 1 = V in across C 1 at t = 5 s and R 1 . I pk = − 7 . 999 20 × 10 3 = − 399 . 95 µ A. R 1 + R 2 11/ 22 12/ 22