
4/8/2018 [1] PE Prof. Mor M. Peretz Analog Electronic Circuits 361-1-3671 M I C T HE C ENTER FOR P OWER E LECTRONICS AND M IXED -S IGNAL IC, B EN -G URION U NIVERSITY BGU Analog Electronic Circuits Prof. Mor M. Peretz The Center for Power Electronics and Mixed-Signal IC Department of Electrical and Computer Engineering Ben-Gurion University of the Negev, ISRAEL Emails: morp@bgu.ac.il Website: http://www.ee.bgu.ac.il/~pemic http://www.ee.bgu.ac.il/~analog [2] PE Prof. Mor M. Peretz Analog Electronic Circuits 361-1-3671 M I BGU C T HE C ENTER FOR P OWER E LECTRONICS AND M IXED -S IGNAL IC, B EN -G URION U NIVERSITY Lesson #3 Outline • Dynamic limitations of OpAmps – Open-loop response – Gain-Bandwidth product • Drawing A OL , 1/ β , β A OL • Closed-loop response – The GBW trade-off • Resistive circuits • Frequency-dependent circuits • Transient response – Slew-rate [3] PE Prof. Mor M. Peretz Analog Electronic Circuits 361-1-3671 M I BGU C T HE C ENTER FOR P OWER E LECTRONICS AND M IXED -S IGNAL IC, B EN -G URION U NIVERSITY Open-loop response C C + A 1 A 2 1 V CC A 1 =g m1 R eq A 2 i B 1 - IN- R eq C eq Q 1 Q 2 IN+ V out i out1 V out1 Q 3 Q 4 C eq g m1 g m1 R eq V EE R eq V out1 V out2 V out V + C eq + ( V + - V - ) A 1 V out1 A 2 + + V - LM324 (Texas Instruments, National) 1
4/8/2018 [4] PE Prof. Mor M. Peretz Analog Electronic Circuits 361-1-3671 M I C T HE C ENTER FOR P OWER E LECTRONICS AND M IXED -S IGNAL IC, B EN -G URION U NIVERSITY BGU Open-loop response R eq V out1 A 1 A 2 A 3 = A OL V out2 V out A [ dB ] V + C eq + ( V + - V - ) A 1 V out1 A 2 + + V - -20 [dB/dec] V out1 C eq f [ Hz ] f c g m1 R eq 𝐵 𝐵 𝑃𝑀 = f unity 1 1+𝑘 𝑔 2 π R eq C eq 𝑔𝐷 [5] PE Prof. Mor M. Peretz Analog Electronic Circuits 361-1-3671 M I BGU C T HE C ENTER FOR P OWER E LECTRONICS AND M IXED -S IGNAL IC, B EN -G URION U NIVERSITY Gain-Bandwidth Product - GBW 𝐵 𝑃𝑀 𝐵 𝐷𝑀 = 𝐻 A 1 A 2 A 3 = A OL A [ dB ] 1 + 𝛾𝐵 𝑃𝑀 -20 [dB/dec] 𝛾 = 1, 𝐻 = 1 𝐵 𝑃𝑀 𝐵 𝐷𝑀 = 1 + 𝐵 𝑃𝑀 f c f [ Hz ] 𝐻𝐶𝑋 = 𝐵 𝑃𝑀 𝑔 𝑑 = 𝑔 𝑣𝑜𝑗𝑢𝑧 1 f unity 2 π R eq C eq [6] PE Prof. Mor M. Peretz Analog Electronic Circuits 361-1-3671 M I BGU C T HE C ENTER FOR P OWER E LECTRONICS AND M IXED -S IGNAL IC, B EN -G URION U NIVERSITY Loop-Gain Nyquist Criterion 𝐵 𝑃𝑀 𝑡 𝐵 𝐷𝑀 = 𝐻 𝑡 1 + 𝛾𝐵 𝑃𝑀 𝑡 • The system is unstable if the characteristic equation {1+ β A OL (s)} has roots in the right half of the complex plane • Nyquist criterion is a test for location of {1+ β A OL (s)} roots • Nyquist criterion can be viewed on the frequency domain (Bode) 2
4/8/2018 [7] PE Prof. Mor M. Peretz Analog Electronic Circuits 361-1-3671 M I C T HE C ENTER FOR P OWER E LECTRONICS AND M IXED -S IGNAL IC, B EN -G URION U NIVERSITY BGU Loop-gain on the frequency domain |LG| [dB] f A f X’ in X out X in X e + - A OL G X f f β +180 f 0 In negative feedback o o systems 180 ( 180 ) At f 0 [8] PE Prof. Mor M. Peretz Analog Electronic Circuits 361-1-3671 M I BGU C T HE C ENTER FOR P OWER E LECTRONICS AND M IXED -S IGNAL IC, B EN -G URION U NIVERSITY Bode plot Phase margin [dB] A A 1 f f 0 o o m ( 180 ) 180 m | A | 1 | A | 1 -180 [9] PE Prof. Mor M. Peretz Analog Electronic Circuits 361-1-3671 M I BGU C T HE C ENTER FOR P OWER E LECTRONICS AND M IXED -S IGNAL IC, B EN -G URION U NIVERSITY Graphical representation of β A OL conventional method A [ dB ] AB [ dB ] A AB f [ Hz ] B [ dB ] f [ Hz ] B f f f 1 2 3 f [ Hz ] f f f 1 2 3 Tedious – need to re-plot BA Analysis (not design) oriented Requires iterations 3
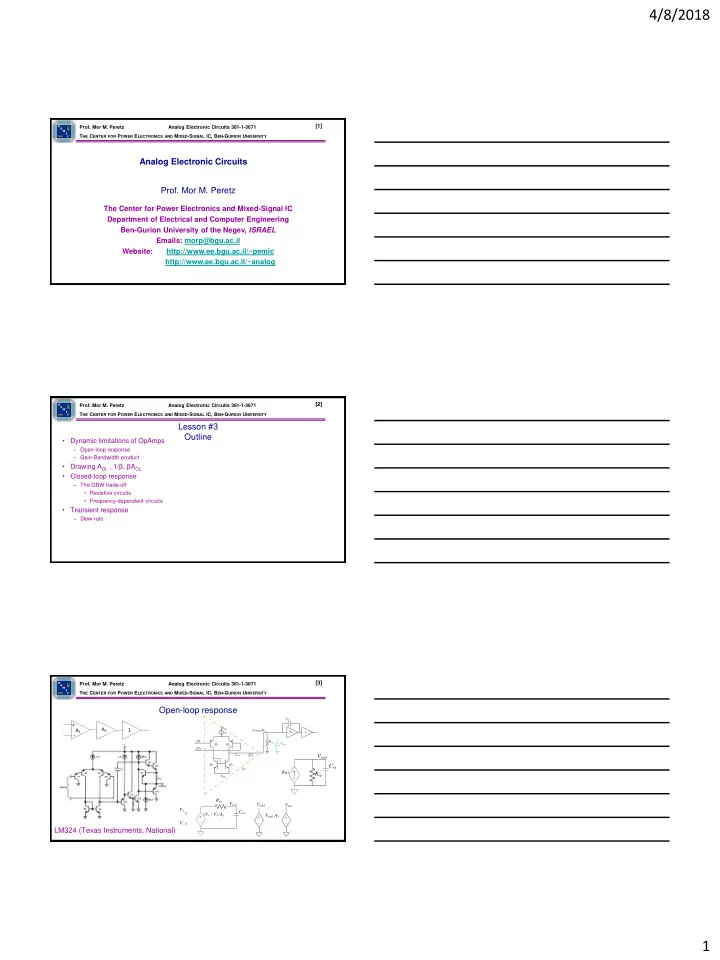
4/8/2018 [10] PE Prof. Mor M. Peretz Analog Electronic Circuits 361-1-3671 M I C T HE C ENTER FOR P OWER E LECTRONICS AND M IXED -S IGNAL IC, B EN -G URION U NIVERSITY BGU Graphical Representation of β A OL 1 20log A 20log 20log(BA) B A [ dB ] A 1 20logA 20log B A 1 B BA 1 LG ( f ) BA 1 B BA BA 1 BA 1 f o [ Hz ] [11] PE Prof. Mor M. Peretz Analog Electronic Circuits 361-1-3671 M I BGU C T HE C ENTER FOR P OWER E LECTRONICS AND M IXED -S IGNAL IC, B EN -G URION U NIVERSITY Closed-loop response Non-inverting Amp + [ dB ] V OUT V in V e A OL - 1/ β 1 R 1 A 0_1 1/ β 2 A 0_2 R 2 𝐻 = 1 1/ β 3 A 0_3 𝛾 = 𝑆 1 + 𝑆 2 1 𝑆 2 f [ Hz ] f c_1 f c_2 f c_3 𝐻𝐶𝑋 = 𝐵 0 𝑔 𝑑 = 𝑔 f unity 𝑣𝑜𝑗𝑢𝑧 [12] PE Prof. Mor M. Peretz Analog Electronic Circuits 361-1-3671 M I BGU C T HE C ENTER FOR P OWER E LECTRONICS AND M IXED -S IGNAL IC, B EN -G URION U NIVERSITY Closed-loop response Inverting Amp R 1 [ dB ] A OL R 2 V in - V OUT V e + 1/ β A 0 𝑆 1 𝐻 = 𝑆 1 + 𝑆 2 f c f unity 𝛾 = 𝑆 1 + 𝑆 2 1 G f [ Hz ] 𝑆 2 𝐻𝐶𝑋 = 𝐵 0 𝑔 𝑑 = 𝑔 𝑣𝑜𝑗𝑢𝑧 4
4/8/2018 [13] PE Prof. Mor M. Peretz Analog Electronic Circuits 361-1-3671 M I C T HE C ENTER FOR P OWER E LECTRONICS AND M IXED -S IGNAL IC, B EN -G URION U NIVERSITY BGU Closed-loop response Integrator C [ dB ] R V in - A OL V OUT V Ɛ + 1/ β 1 𝐻 = 𝑡𝐷𝑆 + 1 𝛾 = 𝑡𝐷𝑆 + 1 1 G f [ Hz ] 𝑡𝐷𝑆 𝐻𝐶𝑋 = 𝐵 0 𝑔 𝑑 = 𝑔 𝑣𝑜𝑗𝑢𝑧 [14] PE Prof. Mor M. Peretz Analog Electronic Circuits 361-1-3671 M I BGU C T HE C ENTER FOR P OWER E LECTRONICS AND M IXED -S IGNAL IC, B EN -G URION U NIVERSITY Transient response Rise-time (ideal) R eq R C eq C eq I R eq + - V OUT V e V in + V f 0.9V f 0.1V f t t r [15] PE Prof. Mor M. Peretz Analog Electronic Circuits 361-1-3671 M I BGU C T HE C ENTER FOR P OWER E LECTRONICS AND M IXED -S IGNAL IC, B EN -G URION U NIVERSITY 5
4/8/2018 [16] PE Prof. Mor M. Peretz Analog Electronic Circuits 361-1-3671 M I C T HE C ENTER FOR P OWER E LECTRONICS AND M IXED -S IGNAL IC, B EN -G URION U NIVERSITY BGU 6
Recommend
More recommend