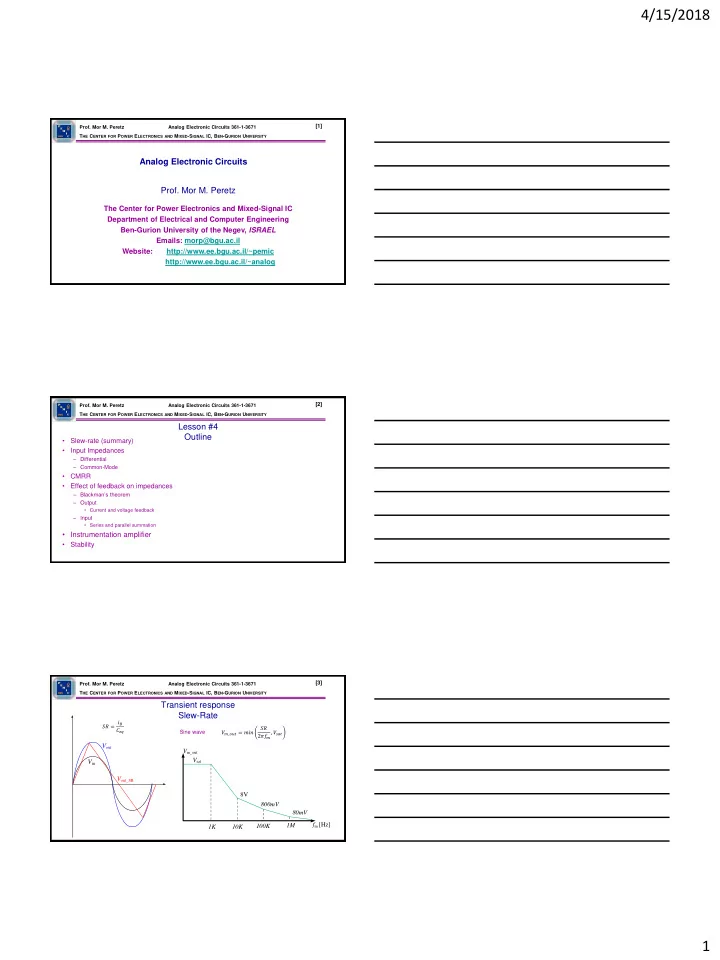

4/15/2018 [1] PE Prof. Mor M. Peretz Analog Electronic Circuits 361-1-3671 M I C T HE C ENTER FOR P OWER E LECTRONICS AND M IXED -S IGNAL IC, B EN -G URION U NIVERSITY BGU Analog Electronic Circuits Prof. Mor M. Peretz The Center for Power Electronics and Mixed-Signal IC Department of Electrical and Computer Engineering Ben-Gurion University of the Negev, ISRAEL Emails: morp@bgu.ac.il Website: http://www.ee.bgu.ac.il/~pemic http://www.ee.bgu.ac.il/~analog [2] PE Prof. Mor M. Peretz Analog Electronic Circuits 361-1-3671 M I BGU C T HE C ENTER FOR P OWER E LECTRONICS AND M IXED -S IGNAL IC, B EN -G URION U NIVERSITY Lesson #4 Outline • Slew-rate (summary) • Input Impedances – Differential – Common-Mode • CMRR • Effect of feedback on impedances – Blackman’s theorem – Output • Current and voltage feedback – Input • Series and parallel summation • Instrumentation amplifier • Stability [3] PE Prof. Mor M. Peretz Analog Electronic Circuits 361-1-3671 M I BGU C T HE C ENTER FOR P OWER E LECTRONICS AND M IXED -S IGNAL IC, B EN -G URION U NIVERSITY Transient response Slew-Rate 𝑇𝑆 = 𝑗 𝐶 𝑇𝑆 𝐷 𝑓𝑟 𝑊 𝑛_𝑝𝑣𝑢 = 𝑛𝑗𝑜 , 𝑊 Sine wave 𝑡𝑏𝑢 2𝜌𝑔 𝑛 V out V m_out V sat V in V out_SR 8V 800mV 80mV f m [Hz] 100K 1M 1K 10K 1
4/15/2018 [4] PE Prof. Mor M. Peretz Analog Electronic Circuits 361-1-3671 M I C T HE C ENTER FOR P OWER E LECTRONICS AND M IXED -S IGNAL IC, B EN -G URION U NIVERSITY BGU Input Impedances V1 - Vd/2 V OUT + R d 𝑑𝑛 = 𝑊 1 + 𝑊 + 2 𝑊 2 𝑊 𝑒 = 𝑊 1 − 𝑊 R cm 2 R cm Vcm + + Vd/2 V2 [5] PE Prof. Mor M. Peretz Analog Electronic Circuits 361-1-3671 M I BGU C T HE C ENTER FOR P OWER E LECTRONICS AND M IXED -S IGNAL IC, B EN -G URION U NIVERSITY Input Impedances Differential VCC ℎ 𝑗𝑓 = 𝑊 𝑈 ℎ 𝑔𝑓 + 1 𝐽 𝑓 𝑊 𝑈 𝑆 𝑒 = 2ℎ 𝑗𝑓 = 2 ℎ 𝑔𝑓 + 1 𝐽 𝑓 OUT IN- IN+ Q 1 Q 2 R in VEE [6] PE Prof. Mor M. Peretz Analog Electronic Circuits 361-1-3671 M I BGU C T HE C ENTER FOR P OWER E LECTRONICS AND M IXED -S IGNAL IC, B EN -G URION U NIVERSITY Input Impedances Common-mode VCC 𝑆 𝑓 = 1 ℎ 𝑝𝑓 OUT 𝑆 𝑑𝑛 = 2𝑆 𝑓 ℎ 𝑔𝑓 + 1 IN- IN+ Q 1 Q 2 V EE R in VEE 2
4/15/2018 [7] PE Prof. Mor M. Peretz Analog Electronic Circuits 361-1-3671 M I C T HE C ENTER FOR P OWER E LECTRONICS AND M IXED -S IGNAL IC, B EN -G URION U NIVERSITY BGU Common-Mode Rejection Ratio (CMRR) 𝐷𝑁𝑆𝑆 ≡ 𝐵 𝑒 = 𝐵 𝑒 𝐵 2 𝐵 3 𝑊 𝑑𝑛 = 0 R 1 𝐵 𝑑𝑛 𝐵 𝑑𝑛 𝐵 2 𝐵 3 R 2 V 2 V in - V OUT V 1 + 𝑑𝑛 = 𝑊 1 + 𝑊 2 𝑊 2 𝑊 𝑒 = 𝑊 1 − 𝑊 2 V 1 + V OUT V in V 2 - 𝑊 1 + 𝑊 2 R 1 𝑊 𝑝𝑣𝑢_𝑑𝑛 = 𝐵 𝑑𝑛 𝑊 𝑑𝑛 = 𝐵 𝑑𝑛 2 R 2 𝑊 𝑑𝑛 = 𝑊 𝑗𝑜 [8] PE Prof. Mor M. Peretz Analog Electronic Circuits 361-1-3671 M I BGU C T HE C ENTER FOR P OWER E LECTRONICS AND M IXED -S IGNAL IC, B EN -G URION U NIVERSITY Common-Mode Rejection Ratio (CMRR) 𝑊 𝑑𝑛 = 0 R 1 𝑊 𝑝𝑣𝑢 = 𝐵 𝑑𝑛 𝑊 𝑑𝑛 + 𝐵 𝑒 𝑊 𝑒 R 2 V 2 V in - V OUT 𝑊 𝑊 Reflected to input 𝑝𝑣𝑢 𝐷𝑁𝑆𝑆 + 𝑊 𝑑𝑛 𝐵 𝑒 = V 1 𝑒 + (as done to ALL non-idealities) V 1 + V OUT V in V 2 - R 1 R 2 𝑊 𝑑𝑛 = 𝑊 𝑗𝑜 [9] PE Prof. Mor M. Peretz Analog Electronic Circuits 361-1-3671 M I BGU C T HE C ENTER FOR P OWER E LECTRONICS AND M IXED -S IGNAL IC, B EN -G URION U NIVERSITY Blackman’s Theorem 1+𝛾𝐵 𝑇𝐷 𝑎 𝐺 = 𝑎 𝑂𝐺 1+𝛾𝐵 𝑃𝐷 V’ in Load V’ in Load V in V e V in V e + - A OL + - A OL G G V out I out V f V f β β Voltage feedback Current feedback 𝑎 𝑂𝐺 𝑎 𝐺 = 𝑎 𝐺 = 𝑎 𝑂𝐺 1 + 𝛾𝐵 1+𝛾𝐵 3
4/15/2018 [10] PE Prof. Mor M. Peretz Analog Electronic Circuits 361-1-3671 M I C T HE C ENTER FOR P OWER E LECTRONICS AND M IXED -S IGNAL IC, B EN -G URION U NIVERSITY BGU Effect of feedback Output impedance Voltage feedback Current feedback V 1 + V OUT I out V in V ref + V 2 - - R 1 R 2 R 1+𝛾𝐵 𝑇𝐷 1+𝛾𝐵 𝑇𝐷 𝑎 𝑝𝑣𝑢_𝐺 = 𝑆 0 𝑎 𝑝𝑣𝑢_𝐺 = 𝑆 0 1+𝛾𝐵 𝑃𝐷 1+𝛾𝐵 𝑃𝐷 [11] PE Prof. Mor M. Peretz Analog Electronic Circuits 361-1-3671 M I BGU C T HE C ENTER FOR P OWER E LECTRONICS AND M IXED -S IGNAL IC, B EN -G URION U NIVERSITY Effect of feedback Example [ dB ] V 1 + A OL V OUT V in 100 V 2 - R 1 R 0 R 2 40 1/ β 20 R1=10k R2=1k f [ Hz ] R0=100 Z out_F -40 [12] PE Prof. Mor M. Peretz Analog Electronic Circuits 361-1-3671 M I BGU C T HE C ENTER FOR P OWER E LECTRONICS AND M IXED -S IGNAL IC, B EN -G URION U NIVERSITY Linear regulator Low-Drop Out (LDO) Z out_F + Z Load Vout 4
4/15/2018 [13] PE Prof. Mor M. Peretz Analog Electronic Circuits 361-1-3671 M I C T HE C ENTER FOR P OWER E LECTRONICS AND M IXED -S IGNAL IC, B EN -G URION U NIVERSITY BGU Effect of feedback Input impedance + β R 1 V in A V e V OUT - R 2 V in - R 1 V OUT V e + R 2 V f Parallel (current) summation Series (voltage) summation 𝑎 𝑂𝐺 𝑎 𝑗𝑜_𝐺 = 1+𝛾𝐵 𝑎 𝑗𝑜_𝐺 = 𝑎 𝑂𝐺 1 + 𝛾𝐵 [14] PE Prof. Mor M. Peretz Analog Electronic Circuits 361-1-3671 M I BGU C T HE C ENTER FOR P OWER E LECTRONICS AND M IXED -S IGNAL IC, B EN -G URION U NIVERSITY Input Impedances R 1 R 1 R 2 R 2 V 2 V 1 V in - - V OUT V OUT V Ɛ R 3 V 1 V 2 𝑆 1 +𝑠 0 + + 𝑆 𝑗𝑜 = 𝑆 2 + 1+𝛾𝐵 ≈ 𝑆 2 R 4 𝑆 𝑗𝑜_1 = 𝑆 2 V 1 + 𝑆 𝑗𝑜_2 = 𝑆 3 + 𝑆 4 V OUT V in 𝑆 𝑗𝑜_1,2 = 𝑆 2 + 𝑆 3 V 2 - R 1 𝑆 𝑗𝑜 = 𝑆 𝑒 1 + 𝛾𝐵 R 2 [15] PE Prof. Mor M. Peretz Analog Electronic Circuits 361-1-3671 M I BGU C T HE C ENTER FOR P OWER E LECTRONICS AND M IXED -S IGNAL IC, B EN -G URION U NIVERSITY Difference Amplifier R 1 R 2 V 1 - R 1 V OUT V Ɛ R 3 V 2 - + R 2 - V 1 V OUT V Ɛ R 4 + R 3 + V 2 + R 4 𝑆 1 𝑊 𝑝𝑣𝑢_1 = −𝑊 1 𝑆 2 - 𝑆 4 1 + 𝑆 1 𝑊 𝑝𝑣𝑢_2 = 𝑊 2 𝑆 3 + 𝑆 4 𝑆 2 𝑆 2 𝑆 3 𝑆 1 = 𝑆 4 𝑝𝑣𝑢 = 𝑆 1 𝑊 𝑊 2 − 𝑊 1 𝑆 2 5
4/15/2018 [16] PE Prof. Mor M. Peretz Analog Electronic Circuits 361-1-3671 M I C T HE C ENTER FOR P OWER E LECTRONICS AND M IXED -S IGNAL IC, B EN -G URION U NIVERSITY BGU Instrumentation Amplifier V 1 𝑝𝑣𝑢 = 𝑆 1 + 𝑊 𝑊 𝑏𝑛𝑞_𝑐 − 𝑊 𝑏𝑛𝑞_𝑏 V amp_a 𝑆 2 - 𝐽 𝑦 = 𝑊 1 − 𝑊 2 R a 𝑆 𝑦 R 1 R 2 - 𝑊 𝑏𝑛𝑞_𝑐 − 𝑊 𝑏𝑛𝑞_𝑏 = 𝐽 𝑦 𝑆 𝑦 + 𝑆 𝑏 + 𝑆 𝑐 V OUT R x V Ɛ R 3 + 𝑝𝑣𝑢 = 𝑆 1 1 + 𝑆 𝑏 + 𝑆 𝑐 𝑊 𝑊 2 − 𝑊 1 R 4 𝑆 2 𝑆 𝑦 R b - V amp_b + V 2 [17] PE Prof. Mor M. Peretz Analog Electronic Circuits 361-1-3671 M I BGU C T HE C ENTER FOR P OWER E LECTRONICS AND M IXED -S IGNAL IC, B EN -G URION U NIVERSITY Loop-Gain Nyquist Criterion 𝐵 𝑃𝑀 𝑡 𝐵 𝐷𝑀 = 𝐻 𝑡 1 + 𝛾𝐵 𝑃𝑀 𝑡 • The system is unstable if the characteristic equation {1+ β A OL (s)} has roots in the right half of the complex plane • Nyquist criterion is a test for location of {1+ β A OL (s)} roots • Nyquist criterion can be viewed on the frequency domain (Bode) [18] PE Prof. Mor M. Peretz Analog Electronic Circuits 361-1-3671 M I BGU C T HE C ENTER FOR P OWER E LECTRONICS AND M IXED -S IGNAL IC, B EN -G URION U NIVERSITY Loop-gain on the frequency domain |LG| [dB] X’ in f A f X out X in X e + - A OL G X f f β +180 f 0 In negative feedback o o systems 180 ( 180 ) At f 0 6
4/15/2018 [19] PE Prof. Mor M. Peretz Analog Electronic Circuits 361-1-3671 M I C T HE C ENTER FOR P OWER E LECTRONICS AND M IXED -S IGNAL IC, B EN -G URION U NIVERSITY BGU Bode plot Phase margin [dB] A A 1 f f 0 m ( 180 o ) 180 o m | A | 1 | A | 1 -180 [20] PE Prof. Mor M. Peretz Analog Electronic Circuits 361-1-3671 M I BGU C T HE C ENTER FOR P OWER E LECTRONICS AND M IXED -S IGNAL IC, B EN -G URION U NIVERSITY Graphical representation of β A OL conventional method A [ dB ] AB [ dB ] A AB f [ Hz ] B [ dB ] f [ Hz ] B f f f 1 2 3 f [ Hz ] f f f 1 2 3 Tedious – need to re-plot BA Analysis (not design) oriented Requires iterations [21] PE Prof. Mor M. Peretz Analog Electronic Circuits 361-1-3671 M I BGU C T HE C ENTER FOR P OWER E LECTRONICS AND M IXED -S IGNAL IC, B EN -G URION U NIVERSITY Graphical Representation of β A OL 1 20log A 20log 20log(BA) B A [ dB ] A 1 20logA 20log B A 1 B BA LG ( f ) BA 1 1 B BA BA 1 BA 1 f o [ Hz ] 7
Recommend
More recommend