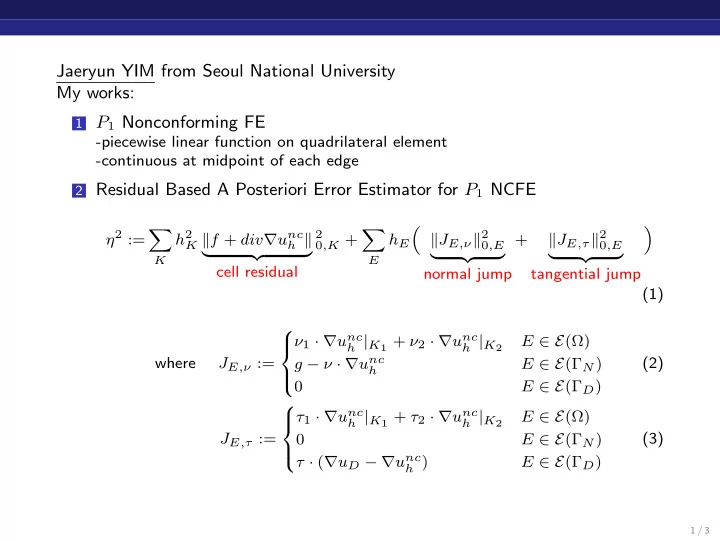
Jaeryun YIM from Seoul National University My works: 1 P 1 - PowerPoint PPT Presentation

Jaeryun YIM from Seoul National University My works: 1 P 1 Nonconforming FE -piecewise linear function on quadrilateral element -continuous at midpoint of each edge 2 Residual Based A Posteriori Error Estimator for P 1 NCFE � � � � η 2 := h 2 K � f + div ∇ u nc 2 � J E,ν � 2 � J E,τ � 2 h � 0 ,K + h E + 0 ,E 0 ,E � �� � � �� � � �� � K E cell residual normal jump tangential jump (1)  ν 1 · ∇ u nc h | K 1 + ν 2 · ∇ u nc h | K 2 E ∈ E (Ω)   g − ν · ∇ u nc where J E,ν := E ∈ E (Γ N ) (2) h   0 E ∈ E (Γ D )  τ 1 · ∇ u nc h | K 1 + τ 2 · ∇ u nc h | K 2 E ∈ E (Ω)   J E,τ := 0 E ∈ E (Γ N ) (3)   τ · ( ∇ u D − ∇ u nc h ) E ∈ E (Γ D ) 1 / 3

Consider −△ u = 0 in Ω = [ − 1 , 1] 2 \ [0 , 1] 2 Exact solution in polar coordinate p ( r, θ ) = r α sin( α ( θ − π/ 2)) ∈ H 1+ α (Ω) , α = 2 / 3 (4) "solution-1.gnuplot" "solution-7.gnuplot" 0.9 0.8 0.8 0.7 0.7 0.6 0.6 0.5 0.5 0.4 0.4 0.3 0.3 0.2 0.2 0.1 0.1 0 0 -0.1 -0.1 -0.6 -0.6 -0.4 -0.4 -0.2 -0.2 0 0 -0.6 0.2 -0.6 -0.4 0.2 -0.4 -0.2 -0.2 0 0 0.2 0.4 0.2 0.4 0.4 0.4 0.6 0.6 0.6 0.6 Figure : (Tops) P 1 NCFE Solution w/ tangential term: (L) After 1 refinement, (R) After 7 (Bottoms) Grid after 7 refinements: (L) Q 1 CFE, (C) P 1 NCFE w/o tangential term, (R) P 1 NCFE w/ tangential term 2 / 3

Further works: tangential jump term on Dirichlet boundary edges coefficient consideration for the general elliptic problem a posterior error estimator for NCFE to another problems 3 / 3