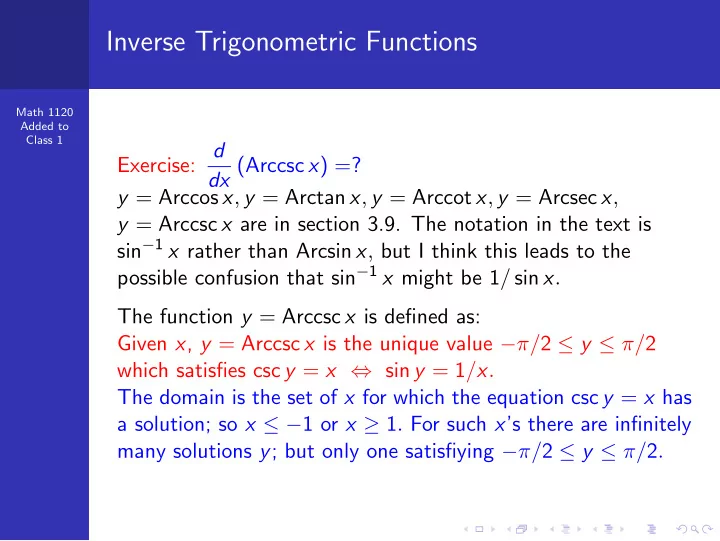
Inverse Trigonometric Functions Math 1120 Added to Class 1 - PowerPoint PPT Presentation

Inverse Trigonometric Functions Math 1120 Added to Class 1 Exercise: d dx (Arccsc x ) =? y = Arccos x , y = Arctan x , y = Arccot x , y = Arcsec x , y = Arccsc x are in section 3.9. The notation in the text is sin − 1 x rather than Arcsin x , but I think this leads to the possible confusion that sin − 1 x might be 1 / sin x . The function y = Arccsc x is defined as: Given x , y = Arccsc x is the unique value − π/ 2 ≤ y ≤ π/ 2 which satisfies csc y = x ⇔ sin y = 1 / x . The domain is the set of x for which the equation csc y = x has a solution; so x ≤ − 1 or x ≥ 1 . For such x ’s there are infinitely many solutions y ; but only one satisfiying − π/ 2 ≤ y ≤ π/ 2 .

Solution to Exercise Math 1120 Rewrite the equation as 1 / sin y = x , or sin y = 1 / x , and Added to Class 1 differentiate both sides (using the chain rule) 1 cos y · y ′ = − 1 / x 2 ⇔ y ′ = − x 2 cos y We need to express y ′ in terms of x alone. Use cos 2 y + sin 2 y = 1 , so solving for cos y we get � � 1 − sin 2 y = ± 1 − 1 / x 2 . cos y = ± However − π/ 2 ≤ y ≤ π/ 2 so cos y ≥ 0 . Since square roots are always positive quantities, the final answer is 1 y ′ = − x 2 � 1 − 1 / x 2

Solution to Exercise continued Math 1120 Added to IMPORTANT: Class 1 1 1 1 1 − 1 − 1 / x 2 = − = − = − √ √ x 2 � � x 2 − 1 x 2 − 1 x 2 − 1 x 2 x 2 √ x 2 | x | x 2 x 2 √ x 2 = | x | NOT x ! The final answer is because 1 (Arccsc x ) ′ = − √ . x 2 − 1 | x | If you use a right triangle with one angle equal to y , the hypotenuse of size x , and the opposite side equal to 1, to compute cos y , you are implicitly assuming that x > 0; this is not the case for the definition of Arccsc .