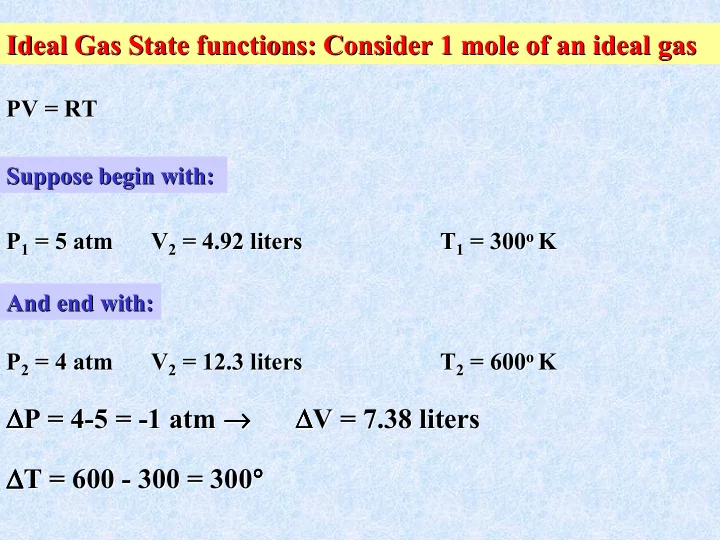
Ideal Gas State functions: Consider 1 mole of an ideal gas Ideal Gas - PowerPoint PPT Presentation

Ideal Gas State functions: Consider 1 mole of an ideal gas Ideal Gas State functions: Consider 1 mole of an ideal gas PV = RT PV = RT Suppose begin with: Suppose begin with: P 1 = 5 atm atm V 2 = 4.92 liters T 1 = 300 o o K K P 1 = 5 V 2 = 4.92 liters T 1 = 300 And end with: And end with: P 2 = 4 atm atm V 2 = 12.3 liters T 2 = 600 o o K K P 2 = 4 V 2 = 12.3 liters T 2 = 600 ∆ P = 4 ∆ → → ∆ V = 7.38 liters ∆ ∆ ∆ → → ∆ ∆ ∆ ∆ ∆ ∆ atm → → → → ∆ ∆ ∆ ∆ P = 4- -5 = 5 = - -1 1 atm V = 7.38 liters ∆ ∆ T = 600 ° ° ∆ ∆ ° ° ∆ ∆ ∆ ∆ 300 = 300 ° ° ° ° T = 600 - - 300 = 300

If we got to the condition 4 atm atm, 12.3 liters, , 12.3 liters, If we got to the condition 4 ° K by going as follows: ° ° ° and 600 ° ° ° ° K by going as follows: and 600 ° ° ° ° → → → 4 → → ° → ° ° ° → 4 → → → , 4.92 liters, 300 ° ° ° ° → → → , 6.15 liters, 300 ° ° ° ° → → → 5 atm atm, 4.92 liters, 300 4 atm atm, 6.15 liters, 300 4 atm atm, , 5 ° ° or by the path: 12.3 liters, 600 ° ° ° ° ° ° or by the path: 12.3 liters, 600 ° ° ° ° → → → 5 → → ° ° → ° ° → 4 → → → , 4.92 liters, 300 ° ° ° ° → → → , 9.84 liters, 600 ° ° ° ° → → → 5 atm atm, 4.92 liters, 300 5 atm atm, 9.84 liters, 600 4 atm atm, , 5 ° ° ° ° 12.3 liters, 600 ° ° ° ° 12.3 liters, 600 ∆ ∆ ∆ ∆ P, ∆ ∆ ∆ ∆ V, ∆ ∆ T ∆ ∆ Would get same ∆ ∆ ∆ ∆ P, ∆ ∆ ∆ ∆ V, ∆ ∆ ∆ ∆ T Would get same Changes in state functions are independent of path. Changes in state functions are independent of path. Important State Functions: Important State Functions: T, P, V, Entropy, Energy and any combination of the above. T, P, V, Entropy, Energy and any combination of the above. Important Non State Functions: Work, Heat. Important Non State Functions: Work, Heat.

Bonus * Bonus * Bonus

dw = = - - p p ext dV dw ext dV Total work done in any change is the sum of little Total work done in any change is the sum of little infinitesimal increments for an infinitesimal change dV dV. . infinitesimal increments for an infinitesimal change ∫ ∫ dw ∫ ∫ ∫ ∫ ∫ - ∫ ∫ ∫ ∫ ∫ = ∫ ∫ ∫ ∫ dw = - p p ext dV = w (work done by the system ) = w (work done by the system ) ext dV Two Examples : Two Examples : ( 1 ) pressure = constant = p p external , ( 1 ) pressure = constant = external , → → v → → i → → → → V changes v i v f V changes v f v f v f ∫ ∫ ∆ ∆ ∆ ∆ V ⇒ ⇒ ⇒ ⇒ ext ∆ ∆ ∆ ∆ ⇒ ⇒ ⇒ ⇒ w = = - - p p ext dV= = - - p p ext dV = = - - p p ext ( v v f - v v i ) = - -p p ext V w ext dV dV ext ( f - i ) = ext v i v i ≠ p ≠ ≠ ≠ ext ≠ ≠ ≠ ≠ {Irreversible Irreversible expansion if expansion if p p ext p gas { gas ≠ ≠ p ≠ ≠ /V ≠ ≠ ≠ ≠ That is if, p p gas = nRT nRT/V p external } That is if, gas = external }

Example 2 : dV ≠ 0, but p ≠ const and T = const: nRT (Called a reversible process.) p ext = p gas = V dV w = - ∫ ∫ ∫ nRT ∫ V v f ∫ v f dV dV ∫ w = - nRT = - nRT v i V V v i [Remembering that ∫ ∫ ∫ f(x) dx is the ∫ w = - nRT ln ( v f / v i ) area under f(x) in a plot of f(x) vs x, w = - ∫ ∫ pdV is the area under p in a ∫ ∫ plot of p vs V.] P, V not const but PV = nRT = const (Isothermal change) {Reversible isothermal expansion because p ext = p gas }

Graphical representation of ∫ p ext dV (If done at constant T, (If done at constant T, Expansion At gas pressure follows Expansion At gas pressure follows P P constantPres- - constantPres curved P= nRT nRT/V path /V path curved P= sure p p ext =P 2 sure ext =P while p p ext remains fixed) while ext remains fixed) P 1 P 2 1 no work no work P= P= nRT nRT/V /V Isothermal reversible P 2 P expansion 2 shaded area = - shaded area = -w w V V V i = V 1 V f = V 2 V i = V V f = V 1 2 P p ext = nRT nRT/V /V P p ext = PV=const const is is PV= a hyperbola a hyperbola Compare the shaded ≠ ≠ ≠ ≠ constant ≠ ≠ ≠ ≠ constant area in the plot above P 1 P P = nRT nRT/V /V P = 1 to the shaded area in the PV= PV= const const plot for a reversible P 2 P 2 isothermal expansion with shaded area = - shaded area = -w w p ext = p p gas = nRT nRT/V /V p ext = gas = V V V i V i = V = V 1 V V f f = V = V 2 1 2

Work done is NOT independent of path : Change the State of a gas two different ways: Consider n moles of an ideal gas Initial condition: T i = 300 K, V i = 2 liter, p i = 2 atm. Final condition: T f = 300 K, V f = 1 liter, p f = 4 atm. Path 1 consists of two steps: ∆ V ≠ ∆ ∆ ∆ ≠ 0 for ≠ ≠      → Step 1 : 2 atm, 2 l , 300K cool at 2 atm, 1 l , 150K this step const − p compress ∆ ∆ ∆ ∆ V=0 for Step 2: Warm at constant V: 2 atm, 1 liter, 150 K → → → → this step 4 atm, 1 liter, 300 K. w = - p ext ( V f - V i ) for the first step, p ext = const = 2 atm w = - 2 atm ( 1 - 2 ) l = 2 l -atm w = 0 for 2nd step since V = const w tot = 2 l -atm

Path 2 is a single step reversible isothermal compression: p ext =p gas = 2 atm, 2 l , 300K → → 4 atm, 1 l , 300K (T constant) → → nRT/V= p v f v f v f ∫ ∫ ∫ dV dV w = - p dV = - nRT = - nRT v i v i V v i V w = - nRT ln ( v f / v i ) = -nRT ln ( 1/2 ) Since nRT = const = PV = 4 l -atm → → → → w = -4 l -atm ( ln 1/2 ) = ( .693 ) 4 l -atm = 2.772 l -atm Compare to w for path 1: w = 2 l -atm w for two different paths between same initial and fianl states is NOT the same. Work is NOT a state Function!

Heat : Just as work is a form of energy, heat is also a form of Heat : Just as work is a form of energy, heat is also a form of energy. energy. Heat is energy which can flow between bodies that are in Heat is energy which can flow between bodies that are in thermal contact. thermal contact. In general heat can be converted to work and work to heat -- -- can can In general heat can be converted to work and work to heat exchange the various energy forms. exchange the various energy forms. Heat is also NOT a state function. The heat change occurring Heat is also NOT a state function. The heat change occurring when a system changes state very definitely depends on the path. when a system changes state very definitely depends on the path. Can prove by doing experiments, or (for ideal gases) can use heat t Can prove by doing experiments, or (for ideal gases) can use hea capacities to determine heat changes by different paths. capacities to determine heat changes by different paths.

The First Law of Thermodynamics The First Law of Thermodynamics I) Energy is a state function for any system : I) Energy is a state function for any system : ∆ E E a ∆ Path a a Path a State 1 State 1 State 2 State 2 ∆ E E b ∆ b Path b Path b ∆ E E a and ∆ E E b are ∆ a and ∆ b are both for going both for going → 2 → from 1 → → → → → → 2 from 1 If E not a state function then: If ∆ ∆ ∆ E ∆ ≠ ∆ ≠ ≠ ≠ ∆ ∆ ∆ E ∆ ∆ ∆ ∆ ∆ a ≠ ≠ ≠ ≠ ∆ ∆ ∆ E not a state function then: E a E b b Suppose ∆ ∆ E ∆ ∆ ∆ ∆ > ∆ ∆ E ∆ ∆ ∆ ∆ ∆ ∆ ∆ ∆ E a E b - now go from state 1 to state 2 along path a, now go from state 1 to state 2 along path a, Suppose a > b - then return to 1 along path b. then return to 1 along path b.

∆ ∆ E = ∆ E ∆ - ∆ ∆ ∆ E Energy change = ∆ ∆ ∆ ∆ E = ∆ ∆ ∆ ∆ ∆ ∆ ∆ ∆ ∆ ∆ ∆ ∆ ∆ E a E b Energy change = a - b ∆ E > 0. Have returned system to its original state ∆ ∆ ∆ ∆ ∆ ∆ ∆ E > 0. Have returned system to its original state and created energy. and created energy. Experimentally find no situation in which energy is created, Experimentally find no situation in which energy is created, ∆ E ∆ ∆ ∆ E therefore, ∆ ∆ ∆ ∆ = ∆ ∆ ∆ ∆ ∆ ∆ ∆ ∆ E a E b and energy is a state function. therefore, a = b and energy is a state function. No one has made a perpetual motion machine of 1st kind. No one has made a perpetual motion machine of 1st kind. The First Law The First Law The energy increase of a system in going between two states equals ls The energy increase of a system in going between two states equa the heat added to the system plus the work done on on the system. the system. the heat added to the system plus the work done