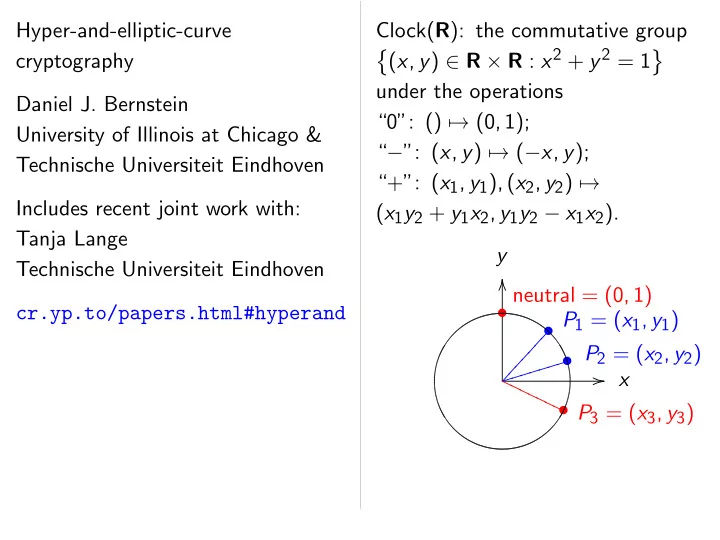
Hyper-and-elliptic-curve Clock( R ): the commutative group ( x; y - PowerPoint PPT Presentation

� � Hyper-and-elliptic-curve Clock( R ): the commutative group ( x; y ) ∈ R × R : x 2 + y 2 = 1 ˘ ¯ cryptography under the operations Daniel J. Bernstein “0”: () �→ (0 ; 1); University of Illinois at Chicago & “ − ”: ( x; y ) �→ ( − x; y ); Technische Universiteit Eindhoven “+”: ( x 1 ; y 1 ) ; ( x 2 ; y 2 ) �→ Includes recent joint work with: ( x 1 y 2 + y 1 x 2 ; y 1 y 2 − x 1 x 2 ). Tanja Lange y Technische Universiteit Eindhoven neutral = (0 ; 1) • cr.yp.to/papers.html#hyperand P 1 = ( x 1 ; y 1 ) • ✂ ✂ ✂ P 2 = ( x 2 ; y 2 ) ✂ • ✐ ✂ ✐ ✐ ✐ ✂ ✐ ✐ x P ✐ ✂ P P P P P P • P 3 = ( x 3 ; y 3 )

� � er-and-elliptic-curve Clock( R ): the commutative group More clo ( x; y ) ∈ R × R : x 2 + y 2 = 1 ˘ ¯ cryptography “A parametrize under the operations J. Bernstein t �→ (sin “0”: () �→ (0 ; 1); University of Illinois at Chicago & is a group “ − ”: ( x; y ) �→ ( − x; y ); echnische Universiteit Eindhoven inducing “+”: ( x 1 ; y 1 ) ; ( x 2 ; y 2 ) �→ Includes recent joint work with: ( x 1 y 2 + y 1 x 2 ; y 1 y 2 − x 1 x 2 ). Lange y echnische Universiteit Eindhoven neutral = (0 ; 1) • cr.yp.to/papers.html#hyperand P 1 = ( x 1 ; y 1 ) • ✂ ✂ ✂ P 2 = ( x 2 ; y 2 ) ✂ • ✐ ✂ ✐ ✐ ✐ ✂ ✐ ✐ x ✐ P ✂ P P P P P P • P 3 = ( x 3 ; y 3 )

� � er-and-elliptic-curve Clock( R ): the commutative group More clock perspectives: ( x; y ) ∈ R × R : x 2 + y 2 = 1 ˘ ¯ “A parametrized clo under the operations Bernstein t �→ (sin t; cos t ) “0”: () �→ (0 ; 1); Illinois at Chicago & is a group hom R “ − ”: ( x; y ) �→ ( − x; y ); Universiteit Eindhoven inducing R = 2 ı Z , ։ “+”: ( x 1 ; y 1 ) ; ( x 2 ; y 2 ) �→ joint work with: ( x 1 y 2 + y 1 x 2 ; y 1 y 2 − x 1 x 2 ). y Universiteit Eindhoven neutral = (0 ; 1) • cr.yp.to/papers.html#hyperand P 1 = ( x 1 ; y 1 ) • ✂ ✂ ✂ P 2 = ( x 2 ; y 2 ) ✂ • ✐ ✂ ✐ ✐ ✐ ✂ ✐ ✐ x P ✐ ✂ P P P P P P • P 3 = ( x 3 ; y 3 )

� � Clock( R ): the commutative group More clock perspectives: ( x; y ) ∈ R × R : x 2 + y 2 = 1 ˘ ¯ “A parametrized clock”: under the operations t �→ (sin t; cos t ) “0”: () �→ (0 ; 1); Chicago & is a group hom R ։ Clock( R “ − ”: ( x; y ) �→ ( − x; y ); Eindhoven inducing R = 2 ı Z , ։ Clock( R “+”: ( x 1 ; y 1 ) ; ( x 2 ; y 2 ) �→ with: ( x 1 y 2 + y 1 x 2 ; y 1 y 2 − x 1 x 2 ). y Eindhoven neutral = (0 ; 1) • cr.yp.to/papers.html#hyperand P 1 = ( x 1 ; y 1 ) • ✂ ✂ ✂ P 2 = ( x 2 ; y 2 ) ✂ • ✐ ✂ ✐ ✐ ✐ ✂ ✐ ✐ x P ✐ ✂ P P P P P P • P 3 = ( x 3 ; y 3 )

� � Clock( R ): the commutative group More clock perspectives: ( x; y ) ∈ R × R : x 2 + y 2 = 1 ˘ ¯ “A parametrized clock”: under the operations t �→ (sin t; cos t ) “0”: () �→ (0 ; 1); is a group hom R ։ Clock( R ) “ − ”: ( x; y ) �→ ( − x; y ); inducing R = 2 ı Z , ։ Clock( R ). “+”: ( x 1 ; y 1 ) ; ( x 2 ; y 2 ) �→ ( x 1 y 2 + y 1 x 2 ; y 1 y 2 − x 1 x 2 ). y neutral = (0 ; 1) • P 1 = ( x 1 ; y 1 ) • ✂ ✂ ✂ P 2 = ( x 2 ; y 2 ) ✂ • ✐ ✂ ✐ ✐ ✐ ✂ ✐ ✐ x P ✐ ✂ P P P P P P • P 3 = ( x 3 ; y 3 )

� � Clock( R ): the commutative group More clock perspectives: ( x; y ) ∈ R × R : x 2 + y 2 = 1 ˘ ¯ “A parametrized clock”: under the operations t �→ (sin t; cos t ) “0”: () �→ (0 ; 1); is a group hom R ։ Clock( R ) “ − ”: ( x; y ) �→ ( − x; y ); inducing R = 2 ı Z , ։ Clock( R ). “+”: ( x 1 ; y 1 ) ; ( x 2 ; y 2 ) �→ “Complex numbers of norm 1”: ( x 1 y 2 + y 1 x 2 ; y 1 y 2 − x 1 x 2 ). { u ∈ C : uu = 1 } is a group under y 1; u �→ u ; u 1 ; u 2 �→ u 1 u 2 . neutral = (0 ; 1) ( x; y ) �→ y + ix is a group hom • P 1 = ( x 1 ; y 1 ) • Clock( R ) , ։ { u ∈ C : uu = 1 } . ✂ ✂ ✂ P 2 = ( x 2 ; y 2 ) ✂ • ✐ ✂ ✐ ✐ ✐ ✂ ✐ ✐ x ✐ P ✂ P P P P P P • P 3 = ( x 3 ; y 3 )

� � Clock( R ): the commutative group More clock perspectives: ( x; y ) ∈ R × R : x 2 + y 2 = 1 ˘ ¯ “A parametrized clock”: under the operations t �→ (sin t; cos t ) “0”: () �→ (0 ; 1); is a group hom R ։ Clock( R ) “ − ”: ( x; y ) �→ ( − x; y ); inducing R = 2 ı Z , ։ Clock( R ). “+”: ( x 1 ; y 1 ) ; ( x 2 ; y 2 ) �→ “Complex numbers of norm 1”: ( x 1 y 2 + y 1 x 2 ; y 1 y 2 − x 1 x 2 ). { u ∈ C : uu = 1 } is a group under y 1; u �→ u ; u 1 ; u 2 �→ u 1 u 2 . neutral = (0 ; 1) ( x; y ) �→ y + ix is a group hom • P 1 = ( x 1 ; y 1 ) • Clock( R ) , ։ { u ∈ C : uu = 1 } . ✂ ✂ ✂ P 2 = ( x 2 ; y 2 ) ✂ • ✐ ✂ ✐ ✐ ✐ “2-dimensional rotations”: ✂ ✐ ✐ x P ✐ ✂ “ y P P P x ” P P ( x; y ) �→ is a P • P 3 = ( x 3 ; y 3 ) − x y group hom Clock( R ) , ։ SO 2 ( R ).

� � R ): the commutative group More clock perspectives: Clocks over ) ∈ R × R : x 2 + y 2 = 1 ¯ “A parametrized clock”: Clock( F 7 the operations ˘ t �→ (sin t; cos t ) ( x; y ) ∈ () �→ (0 ; 1); is a group hom R ։ Clock( R ) Group op ( x; y ) �→ ( − x; y ); inducing R = 2 ı Z , ։ Clock( R ). · ( x 1 ; y 1 ) ; ( x 2 ; y 2 ) �→ · “Complex numbers of norm 1”: + y 1 x 2 ; y 1 y 2 − x 1 x 2 ). { u ∈ C : uu = 1 } is a group under · y 1; u �→ u ; u 1 ; u 2 �→ u 1 u 2 . · neutral = (0 ; 1) ( x; y ) �→ y + ix is a group hom · • P 1 = ( x 1 ; y 1 ) • Clock( R ) , ։ { u ∈ C : uu = 1 } . · ✂ ✂ ✂ P 2 = ( x 2 ; y 2 ) ✂ • · ✐ ✂ ✐ ✐ ✐ “2-dimensional rotations”: ✂ ✐ ✐ x P ✐ ✂ “ y P P P Diagram x ” P P ( x; y ) �→ is a P • P 3 = ( x 3 ; y 3 ) − x y − 3 ; − 2 ; − group hom Clock( R ) , ։ SO 2 ( R ).

� commutative group More clock perspectives: Clocks over finite fields : x 2 + y 2 = 1 ¯ “A parametrized clock”: Clock( F 7 ) = erations ˘ t �→ (sin t; cos t ) ( x; y ) ∈ F 7 × F 7 1); is a group hom R ։ Clock( R ) Group operations as − x; y ); inducing R = 2 ı Z , ։ Clock( R ). · · · · 2 ; y 2 ) �→ · • · · · “Complex numbers of norm 1”: y 2 − x 1 x 2 ). { u ∈ C : uu = 1 } is a group under · · · • · 1; u �→ u ; u 1 ; u 2 �→ u 1 u 2 . · · • · · neutral = (0 ; 1) ( x; y ) �→ y + ix is a group hom · · · • · P 1 = ( x 1 ; y 1 ) • Clock( R ) , ։ { u ∈ C : uu = 1 } . · • · · · ✂ ✂ P 2 = ( x 2 ; y 2 ) • · · · · ✐ ✐ ✐ ✐ “2-dimensional rotations”: x “ y Diagram plots F 7 as x ” P P ( x; y ) �→ is a P • P 3 = ( x 3 ; y 3 ) − x y − 3 ; − 2 ; − 1 ; 0 ; 1 ; 2 ; group hom Clock( R ) , ։ SO 2 ( R ).

commutative group More clock perspectives: Clocks over finite fields ¯ = 1 “A parametrized clock”: Clock( F 7 ) = ( x; y ) ∈ F 7 × F 7 : x 2 + y 2 ˘ t �→ (sin t; cos t ) is a group hom R ։ Clock( R ) Group operations as before. inducing R = 2 ı Z , ։ Clock( R ). · · · · · · · · • · · · · • · · “Complex numbers of norm 1”: ). { u ∈ C : uu = 1 } is a group under · · · • · · · · 1; u �→ u ; u 1 ; u 2 �→ u 1 u 2 . · · • · · • · · · (0 ; 1) ( x; y ) �→ y + ix is a group hom · · · • · · · · ( x 1 ; y 1 ) Clock( R ) , ։ { u ∈ C : uu = 1 } . · • · · · · • · · = ( x 2 ; y 2 ) · · · · · · · “2-dimensional rotations”: x “ y Diagram plots F 7 as x ” ( x; y ) �→ is a ( x 3 ; y 3 ) − x y − 3 ; − 2 ; − 1 ; 0 ; 1 ; 2 ; 3. group hom Clock( R ) , ։ SO 2 ( R ).

More clock perspectives: Clocks over finite fields “A parametrized clock”: Clock( F 7 ) = ( x; y ) ∈ F 7 × F 7 : x 2 + y 2 = 1 ˘ ¯ t �→ (sin t; cos t ) . is a group hom R ։ Clock( R ) Group operations as before. inducing R = 2 ı Z , ։ Clock( R ). · · · · · · · · • · · · · • · · “Complex numbers of norm 1”: { u ∈ C : uu = 1 } is a group under · · · • · · · · 1; u �→ u ; u 1 ; u 2 �→ u 1 u 2 . · · • · · • · · · ( x; y ) �→ y + ix is a group hom · · · • · · · · Clock( R ) , ։ { u ∈ C : uu = 1 } . · • · · · · • · · · · · · · · · “2-dimensional rotations”: “ y Diagram plots F 7 as x ” ( x; y ) �→ is a − x y − 3 ; − 2 ; − 1 ; 0 ; 1 ; 2 ; 3. group hom Clock( R ) , ։ SO 2 ( R ).