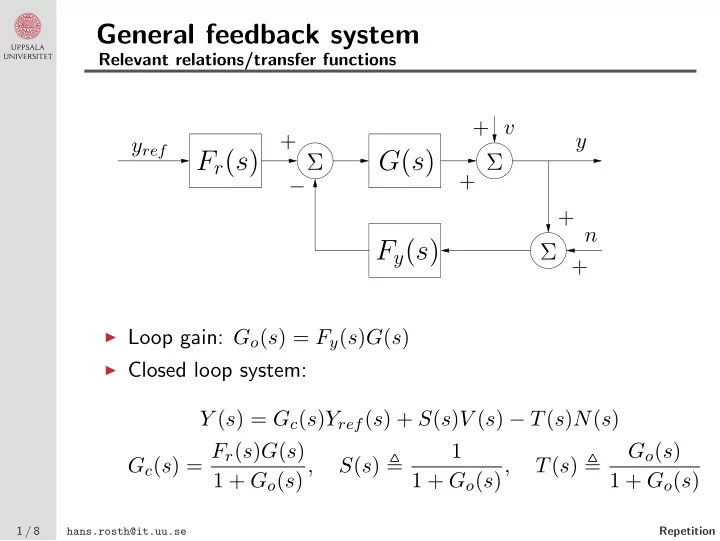
General feedback system Relevant relations/transfer functions + v - PowerPoint PPT Presentation

General feedback system Relevant relations/transfer functions + v + y y ref F r ( s ) G ( s ) Σ Σ + − + n F y ( s ) Σ + ◮ Loop gain: G o ( s ) = F y ( s ) G ( s ) ◮ Closed loop system: Y ( s ) = G c ( s ) Y ref ( s ) + S ( s ) V ( s ) − T ( s ) N ( s ) G c ( s ) = F r ( s ) G ( s ) 1 G o ( s ) S ( s ) � T ( s ) � 1 + G o ( s ) , 1 + G o ( s ) , 1 + G o ( s ) 1 / 8 hans.rosth@it.uu.se Repetition

Bode plots Frequency domain models Example: Second order system ω 2 � � 0 � 1 − ζ 2 G ( s ) = poles in − ω 0 ζ ± i , s 2 + 2 ζω 0 s + ω 2 0 ω 0 = distance from the origin, ζ = damping ratio. 1 0 10 ζ =0.05 ζ =0.05 −30 ζ =0.1 ζ =0.1 ζ =0.3 ζ =1 ζ =0.3 0 −60 10 ζ =0.5 Phase ( o ) ζ =1 ζ =0.5 Gain −90 −1 −120 10 −150 −2 −180 10 −1 0 1 −1 0 1 10 10 10 10 10 10 ω / ω 0 ω / ω 0 ◮ Small damping ratio, ζ ≪ 1 ⇒ resonance peak M p ≥ | G ( iω 0 ) | = 1 2 ζ 2 / 8 hans.rosth@it.uu.se Repetition

The Nyquist criterion Stability for feedback systems Let γ be a semicircle with radius R → ∞ . G o ( s ) maps γ onto γ ′ . G o ( s ) Im Im γ R Re Re γ ′ The Nyquist criterion: ◮ If γ ′ encircles -1 k times counter clockwise, the closed loop system has k more poles in the RHP than G o ( s ) has. ◮ If γ ′ encircles -1 k times clockwise, the closed loop system has k fewer poles in the RHP than G o ( s ) has. 3 / 8 hans.rosth@it.uu.se Repetition

The step response Typical time domain specifications My f r y f 0 . 9 y f e ∞ = r − y f T r = risetime ( 10% – 90% of y f ) T s = settling time (within p % ) M = overshoot (often in % of y f ) e ∞ = steady state error 0 . 1 y f T r time T s 4 / 8 hans.rosth@it.uu.se Repetition

Specifications on the Nyquist curve G o ( iω ) Stability margins 1 ω = ω p Im A m | G o | -1 Re 1 ϕ m 1 A m ω arg G o ω = ω c − i ϕ m − 180 ◦ ω c ω p ω 5 / 8 hans.rosth@it.uu.se Repetition

State space representations Controller canonical form The system with the transfer function b 1 s n − 1 + · · · + b n − 1 s + b n G ( s ) = s n + a 1 s n − 1 + · · · + a n − 1 s + a n can be represented by the controller canonical form :      − a 1 − a 2 · · · − a n − 1 − a n 1        1 0 · · · 0 0 0              0 1 · · · 0 0 0  x = ˙ x + u           . . . . . ...  . . . .   .  . . . . .            0 0 · · · 1 0 0      � �  y =  b 1 b 2 · · · b n x  6 / 8 hans.rosth@it.uu.se Repetition

State space representations Observer canonical form The system with the transfer function b 1 s n − 1 + · · · + b n − 1 s + b n G ( s ) = s n + a 1 s n − 1 + · · · + a n − 1 s + a n can be represented by the observer canonical form :      − a 1 1 0 · · · 0 b 1        − a 2 0 1 · · · 0 b 2         . . . . .  ...     . . . . .  x = ˙ x + u  . . . .   .            − a n − 1 0 0 · · · 1 b n − 1            − a n 0 0 · · · 0 b n      � �  y =  1 0 0 · · · 0 x  7 / 8 hans.rosth@it.uu.se Repetition

State space representations Solution of the state equation The initial value problem x ( t ) = Ax ( t ) + Bu ( t ) , ˙ x ( t 0 ) = x 0 , has the solution � t x ( t ) = e A ( t − t 0 ) x 0 + e A ( t − τ ) Bu ( τ ) dτ. t 0 The matrix exponential function: ∞ 1 e At � � k !( At ) k , L [ e At ] = ( sI − A ) − 1 k =0 8 / 8 hans.rosth@it.uu.se Repetition