Fractions, Decimals, Ratios and Percentages Fraction Line - PowerPoint PPT Presentation

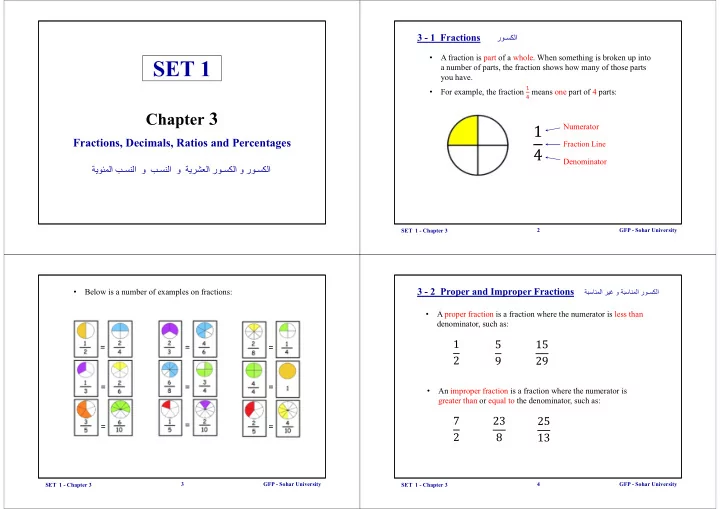
3 -1 Fractions روـسكلا • A fraction is part of a whole. When something is broken up into a number of parts, the fraction shows how many of those parts you have. • For example, the fraction means one part of 4 parts: Numerator Fractions, Decimals, Ratios and Percentages Fraction Line Denominator ةيوئملا بـسنلا و بـسنلا و ةيرشعلا روـسكلا و روـسكلا SET 1 - Chapter 3 2 GFP - Sohar University 3 - 2 Proper and Improper Fractions ةبسانملا ريغ و ةبسانملا روـسكلا • Below is a number of examples on fractions: • A proper fraction is a fraction where the numerator is less than denominator, such as: = = = = = = • An improper fraction is a fraction where the numerator is greater than or equal to the denominator, such as: = = = 3 GFP - Sohar University 4 GFP - Sohar University SET 1 - Chapter 3 SET 1 - Chapter 3

3 - 3 Mixed Numbers ةطلتخملا دادعلؤا Example 1: • An improper fraction can also be written as a mixed number. Solution: • A mixed number is a number that contains both a whole number and a proper fraction. = = = = SET 1 - Chapter 3 5 GFP - Sohar University SET 1 - Chapter 3 6 GFP - Sohar University 3 - 4 Decimals Example 2: ةيرـشعلا روـسكلا • A decimal is a fraction that has a denominator of a power of ten. Solution: • A point or dot is used to separate the whole number part from the fractional part of a number. • For example, in the number 17.591, the decimal point separate 17 (the whole number part) from 591 (the fractional part). Example 3: Solution: 7 GFP - Sohar University 8 GFP - Sohar University SET 1 - Chapter 3 SET 1 - Chapter 3

Example 4: Example 5: Solution: Solution: 32.65 18.431 rounded to 4 decimal places 24.176 + 75.257 So, 32.65 + 18.431 + 24.176 = 75.257 rounded to 4 decimal places 372.18 318.54 ‒ 53.64 ‒ 117.25 318.54 201.29 Thus, 372.18 ‒ 53.64 ‒ 117.25 = 201.29 SET 1 - Chapter 3 9 GFP - Sohar University SET 1 - Chapter 3 10 GFP - Sohar University Example 6: Example 7: Evaluate (a) 2.134 × 5.16 (b) 18.142 × 10 (c) 18.142 × 100 (d) 18.142 × 1000 Solution: Solution: (b) 18.142 × 10 = 181.42 rounded to 3 significant places (c) 18.142 × 100 = 1814.2 (c) 18.142 × 1000 = 18142 rounded to 3 significant places rounded to 3 significant places 11 GFP - Sohar University 12 GFP - Sohar University SET 1 - Chapter 3 SET 1 - Chapter 3

3 - 5 Ratios Example 8: Evaluate: (a) 5284.32 ÷ 10 (b) 5284.32 ÷ 100 بـسـنلا (c) 5284.32 ÷ 1000 • A ratio is a comparison of two or more numbers which represent different objects. Solution: • It can be written with a colon (1:5), or using the word "to" (1 to 5), or as a fraction ( ). (a) 5284.32 ÷ 10 = 528.432 • For example, in the following figure there are 5 circles and 6 squares. (b) 5284.32 ÷ 100 = 52.8432 • So, the ratio of circles to squares (c) 5284.32 ÷ 1000 = 5.28432 is (5 : 6) or (5 to 6) or ( ) • And, the ratio of squares to circles is (6 : 5) or (6 to 5) or ( ) SET 1 - Chapter 3 13 GFP - Sohar University SET 1 - Chapter 3 14 GFP - Sohar University Example 9: A classroom contains 32 tables and 28 chairs. Find the Example 11: Flavours, sugar and milk are used in an ice-cream recipe in ratio of tables to chairs and write it in the simplest form. the ratio of 2 to 3 to 10 respectively. How much flavours, sugar and milk should be used to produce 60 kg of this type Solution: of ice-cream? Ratio Solution: Thus, tables to chairs ratio or 8 : 7 or 8 to 7 Total number of parts = 2 + 3 + 10 = 15 1 part of the 60 kg Example 10: The number of students in a Mathematic class is 45. What is 2 parts = 2 × 4 = 8 kg the boys to girls ratio if there are 15 boys in that class, 3 parts = 3 × 4 = 12 kg expressed in the simplest form? 10 parts = 10 × 4 = 40 kg Solution: So, 8 kg of flavours, 12 kg of sugar, and 40 kg of milk should be Number of girls in the class = 45 ‒ 15 = 30 used to produce 60 kg of this type of ice-cream. Boys to girls ratio or 1 to 2 (check: 8 + 12 + 40 = 60) 15 GFP - Sohar University 16 GFP - Sohar University SET 1 - Chapter 3 SET 1 - Chapter 3

3 - 6 Percentages Example 12: Express the following as percentages: (a) 0.32 (b) 2.51 ةيوئملا بـسـنلا (c) 9.0582 (d) (e) (f) • Percentage is a ratio with a base of 100. • Below are two examples on percentages: Solution: 25% of the squares are green 50% of the squares are green 75% of the squares are white 50% of the squares are white SET 1 - Chapter 3 17 GFP - Sohar University SET 1 - Chapter 3 18 GFP - Sohar University Example 13: Express the following as decimal fractions: Example 14: Express the following as proper fractions: (a) 25% (b) 40% (c) 81.3% (d) 3.75% (a) 30% (b) 42% (c) 21.5% Solution: Solution: 19 GFP - Sohar University 20 GFP - Sohar University SET 1 - Chapter 3 SET 1 - Chapter 3

Example 17: A notebook has 250 pages out of which 172 pages were used. Example 15: Express the following as mixed numbers: (a) 340% (b) 225% What is the percentage of unused pages? Solution: Solution: Example 18: In the first semester, 350 students registered an English course Example 16: Evaluate 12.7% of 257 and 40% of them were boys. Calculate the number of girls who registered this course. Solution: Solution: SET 1 - Chapter 3 21 GFP - Sohar University SET 1 - Chapter 3 22 GFP - Sohar University