Figure 1: Sc hematic of the exp erimen tal set up. The - PDF document

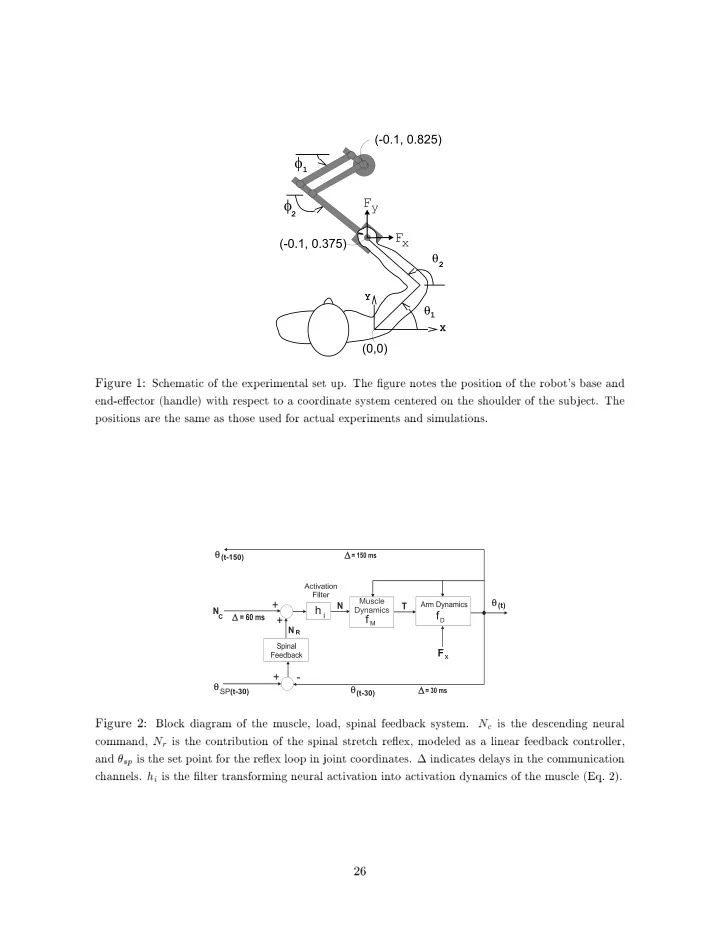
θ θ Figure 1: Sc hematic of the exp erimen tal set up. The �gure notes the p osition of the rob ot's base and end-e�ector (handle) with resp ect to a co ordinate system cen tered on the shoulder of the sub ject. The p ositions are the same as those used for actual exp erimen ts and sim ulations. Figure 2: Blo c k diagram of the m uscle, load, spinal feedbac k system. N is the descending neural c command, N is the con tribution of the spinal stretc h re�ex, mo deled as a linear feedbac k con troller, r and � is the set p oin t for the re�ex lo op in join t co ordinates. � indicates dela ys in the comm unication sp c hannels. h is the �lter transforming neural activ ation in to activ ation dynamics of the m uscle (Eq. 2). i 26

Inverse Muscle Model External Forces (A1) (B1) (A2) (B2) 0.4 0.4 Hand Velocity (m/s) 0.3 0.3 0.2 0.2 0.1 0.1 0 0 −0.1 0.5 1 1.5 2 0.5 1 1.5 2 Time (sec) Time (sec) Figure 3: Blo c k diagram illustrating a feedforw ard con troller that utilizes an in v erse m uscle mo del for assignmen t of neural activ ations to the m uscles, without accoun ting for dynamics of the lim b. The �gures in the middle ro w sho w sim ulation results of hand tra jectory in a n ull �eld (A1) and in force �eld B (B1). Figures in the b ottom ro w are for the same t w o conditions, except that they sho w v elo cit y of 1 the hand for a mo v emen t to the b ottom-most target (at -90 ). The gra y line is v elo cit y in the direction � parallel to the direction of target (i.e., along the y-axis of Fig. 1), and the blac k line is the v elo cit y in a direction p erp endicular to that of target (i.e., along the x-axis of Fig. 1). The resp onse of the system to the unmo deled inertial dynamics of the lim b and to the force �eld is en tirely due to spring-lik e nature of the m uscles and the feedbac k pro vided b y the spinal system. 27

(A1) (B1) (A2) (B2) 0.4 0.4 0.3 Hand Velocity (m/s) Hand Velocity (m/s) 0.3 0.2 0.2 0.1 0.1 0 −0.1 0 0.5 1 1.5 2 0.5 1 1.5 2 Time (s) Time (s) Figure 4: Blo c k diagram illustrating a feedforw ard con troller that utilizes an in v erse dynamics mo del of the inertial prop erties of the lim b, as w ell as an in v erse m uscle mo del, for assignmen t of neural activ ations to the m uscles. Figures in the middle ro w (A1 and A2) sho w hand tra jectory of the system in a n ull �eld and in �eld B . Figures in the b ottom ro w (B1 and B2) sho w v elo cit y of the hand for a mo v emen t 1 to the b ottom-most target. The gra y line is v elo cit y along the y-axis, and the blac k line is the v elo cit y along the x-axis. The resp onse of the system to force �eld is en tirely due to spring-lik e nature of the m uscles and the feedbac k pro vided b y the spinal system. 28

Figure 5: T ra jectories are in �eld B after sub ject and con trollers had adapted to �eld B . (A) Hand 2 1 tra jectories for the con troller of Fig. 4, where only an in v erse mo del is used. (B) T ra jectories for a t ypical sub ject. (C) T ra jectories for a con troller corresp onding to Fig. 11 (switc h set to 1) whic h used a forw ard mo del in conjunction with an in v erse mo del. First ro w: hand paths for 8 mo v emen t directions. Second ro w: v elo cit y along the y-axis (gra y line, parallel to the direction of target) and x-axis (blac k line, p erp endicular to the direction of target) for a mo v emen t to w ard a target at -90 � . Third ro w: hand sp eed and segmen tation p oin ts S for a mo v emen t to w ard -90 . F ourth ro w: deriv ativ e of v elo cit y direction � i and corresp onding segmen tation p oin ts for a mo v emen t to w ard -90 . Fifth ro w: segmen tation of the � hand's tra jectory . 29

Figure 6: T ra jectory c haracteristics during a reac hing mo v emen t to w ard the b ottom most target (-90 ) � for 16 sub jects in force �eld B after adaptation to �eld B (middle bar, dark gra y). W e ha v e also plotted 2 1 the results of 29 sim ulations of in v erse mo del con troller (ligh t gra y , corresp onding to the con troller in Fig. 4) and 35 sim ulations of the forw ard-in v erse mo del feedbac k con troller (blac k, corresp onding to the con troller in Fig. 11, switc h set to 1) for the same mo v emen t. The tra jectory parameters refer to the segmen tation sho wn in Fig. 5. � is angle ab out a segmen tation p oin t, t is the time to reac h the i i i -th segmen tation p oin t, d is the distance to the i -th segmen tation p oin t, j v j is the hand sp eed at the i i segmen tation p oin t, and N is the n um b er of segmen tation p oin ts in the tra jectory . The v alue prin ted s at the top of eac h bar triplet is the v alue at the mean for the highest bar in the triplet. Note that for � , 1 d , and t , i.e., the initial part of the mo v emen t, p erformance of b oth con trollers v ery closely matc hes 1 1 that of the exp erimen tal data. Ho w ev er, in later stages of the mo v emen t only the forw ard mo del based con troller of Fig. 11 con tin ues to accurately predict the exp erimen tal data. ^ Figure 7: Blo c k diagram sho wing ho w a forw ard mo del f of a non-linear system f can b e used to p p construct an observ er for a time-dela y ed nonlinear system where the state at time t is estimated from the measured state at time t � t and the orderly cascade of descending commands N from time t � t 0 c 0 to t . 30

Figure 8: A con trol metho d that uses a forw ard mo del in m uscle co ordinates. The switc h is in p osition 2 for feedbac k con trol using only the forw ard mo del, and in p osition 1 when a forw ard mo del is used in conjunction with an in v erse mo del. y x G + _ G ^ Figure 9: A simple linear system that uses a forw ard mo del G of system dynamics G . Note that ^ � 1 y =x = 1 = (1 + G ) � G , i.e., the feedbac k lo op e�ectiv ely appro ximate an in v erse of the plan t dynamics. 31

(A1) (B1) (C1) (A2) (B2) (C2) 0.4 0.3 0.3 Hand Velocity (m/s) 0.3 0.2 0.2 0.2 0.1 0.1 0.1 0 0 0 −0.1 −0.1 0.5 1 1.5 2 0.5 1 1.5 2 0.5 1 1.5 2 Time (s) Time (s) Time (s) Figure 10: A con trol sc heme that uses a forw ard mo del in join t co ordinates. The switc h is in p osition 2 for con trol via only the forw ard mo del (FM), and in p osition 1 for con trol via b oth the forw ard and in v erse mo dels (IM). Sim ulated tra jectories for switc h in p osition 2. (A1 and A2) FM exp ects n ull �eld, arm mo v es in the n ull �eld, (B1 and B2) FM exp ects n ull �eld, arm mo v es in force �eld B , (C1 and C2) 1 FM exp ects B , arm mo v es in B . Hand paths are represen ted as dots (big gra y dots- actual; small blac k 1 1 dots- desired) at 20 ms in terv als. Lo w er panel: hand v elo cit y along y-axis (gra y line) and x-axis (blac k line) for a mo v emen t to w ard -90 � . Gains on the linear feedbac k error con troller, K = 30 N.m/rad and p K = 3 N.m/rad/sec, w ere set at 1/2 of the v alue for whic h the system w as marginally stable. Ev en v with a p erfect forw ard mo del, the system is not able to follo w the desired tra jectory . 32