f i
play

F I t P P ( 1 r ) nt G J r t H K A P 1 n - PowerPoint PPT Presentation

Mar 18, 2023 •159 likes •224 views

Lesson 5.1: Part 3 Applications Continuous Compound Interest More frequent compounding Annual Interest F I t P P ( 1 r ) nt G J r t H K A P 1 n where r rate , n # of compoundings and

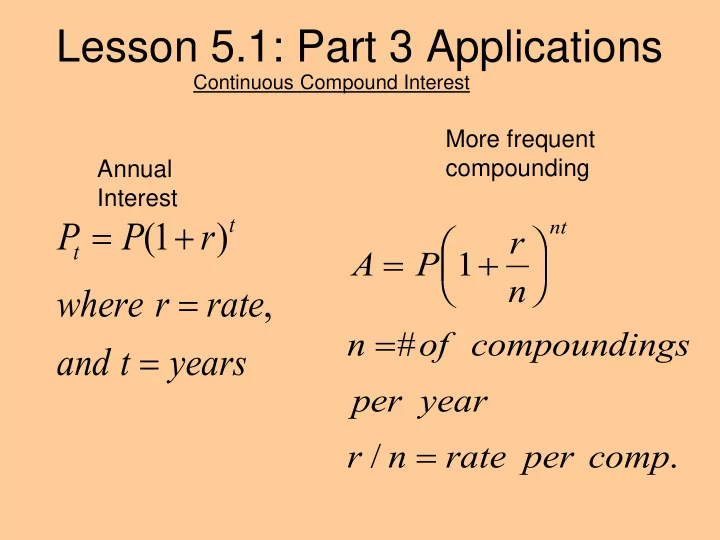
  1. Lesson 5.1: Part 3 Applications Continuous Compound Interest More frequent compounding Annual Interest F I   t P P ( 1 r ) nt G J r t  H  K A P 1 n  where r rate ,  n # of compoundings  and t years per year  r n / rate per comp .

  2. For Continuous Compounding  Pe rt A Ex 8: A total of $12,000 is invested for 5 years at an annual interest rate of 9%. Find the balance if it is compounded a.Quarterly b.Monthly c.Continuously

  3. P = $12,000 r = 9% t = 5 years Quarterly Monthly F I nt r F I H K nt   r A P 1 H K   A P 1 n n F I F I 12 5 ( ) . 09 4 5 ( ) H K . 09   P 12000 1 H K   P 12000 1 5 12 5 4 a f a f   . 60 P 12000 1 0075   . 20 P 12000 1 0225 5 5 a f a f  20  60 P 12000 10225 . P 12000 10075 . 5 5  $18726.  $18788. P 11 P 17 5 5

  4. P = $12,000 r = 9% t = 5 years Continuously  Pe rt A  12000 . 09 5 ( ) A e  12000 . 45 A e A  $18819.75

  5. Radioactive Decay Ex 9: The half-life of the radioactive decay rate of the plutonium that spread across Chernobyl, in 1986, in the former Soviet Union, can be modeled using the function  F G I t / 24 360 , J 10 1 H K P 2 where P is the amount of an original 10 lbs of plutonium remaining after t years. If t = 0 represents 1986, how much plutonium is left in 2005? How much plutonium would be left after 24,360 year? 100,000 years?

  6. After 24,360 years (extra writing space) P  F H I 24360 10 1 24360 K 2005 = 19 years 2 P  F H I 19 10 1 P  F H I 24360 K 1 10 1 K  5 lbs 2 2  9 99 P . lbs P  F H I 100000 24360  0 58 10 1 K After 100,000 years . lbs 2 Homework: p373 #51-57 odd

Recommend

More recommend