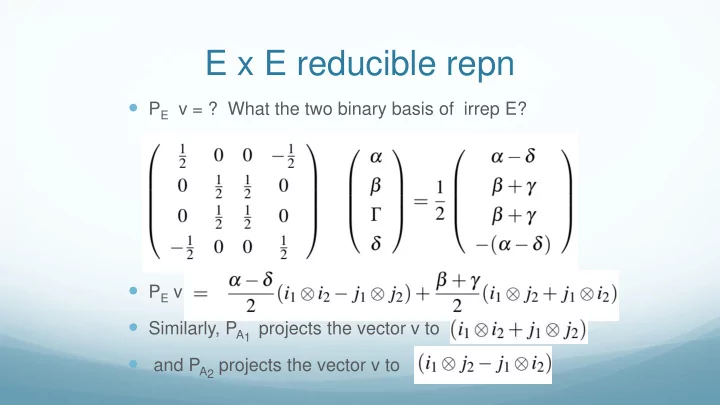
E x E reducible repn P E v = ? What the two binary basis of irrep - PowerPoint PPT Presentation

E x E reducible repn  P E v = ? What the two binary basis of irrep E?  P E v  Similarly, P A1 projects the vector v to  and P A2 projects the vector v to

Recall Character table Binary basis emerging from tensor product (x,y) component of electric dipole moment p vector belongs to irrep E z-component of p belongs to A 1 All observables can be associated with irreps

Recall Character table Binary basis emerging from tensor product What about quadrupole moment tensor- binary basis

SELECTION RULES For operator f, whether this is non-zero/zero. Gives allowed/forbidden transitions

Selection rules 

Selection rules contd  For a system with group symmetry G, the transition from an initial state to final state due to interaction is  Is this zero or non-zero?  Note that initial state belongs to one irrep αof G  Final state also to an irrep β of G  The interaction operator belongs to an irrep γ  The integrand belongs to  The transition is allowed if the tensor product allows A 1

Examples  Let us look at electric dipole moment transitions for systems with group symmetry C 4v  A 1 A 2  Is this allowed or forbidden?

Electric dipole moment belongs to irrep F 1 of O F 1 × A 1 =F 1, F 1 × A 2 =F 2, F 1 × E=F 1 + F 2 F 1 × F 1 =A 1 +E+ F 1 +F 2 , F 1 × F 2 =A 2 +E+ Belongs to F 2 of O F 1 +F 2 , A 1 to A 2 electric dipole moment transition is forbidden.

Polar vector Vs Axial vector  Polar vectors are same as axial vectors for molecules with no inversion symmetry/mirror symmetry.  For group O, the selection rules is same for electric dipole moment and magnetic dipole moment.  For group T d , the selection rule for electric dipole moment transitions is different from the selection rule for magnetic moment transitions.  Write the selection rules for electric dipole and magnetic dipole transitions for group D 3d

Molecular vibrations  Classical problem of two masses connected by spring  Frequency of oscillation/vibration is  Where ϰ is stiffness constant and μ is reduced mass of the system  As the number of masses in the system increase, the number of degrees of freedom ( dof ) increase and oscillatory motion becomes complicated  The number of vibrational degree of freedom is 3N-6- Why? What will be the number of vibrational dof for linear molecule

Molecular vibrations  For a complex system with s dof  Let (x 1 , x 2, … x s ) denote small excursions of mass points whose Lagrangian is  We can diagonalise this so that where  η ɭ are the normal coordinates

Vibrations of the non-linear molecule  For non-linear triatomic molecule, there will be 3 vibrational modes  Bond length changes or bond angle changes

Vibrational modes of nonlinear triatomic molecule