Diffusion Demo Glass tube filled with water. At time t = 0, add - PowerPoint PPT Presentation

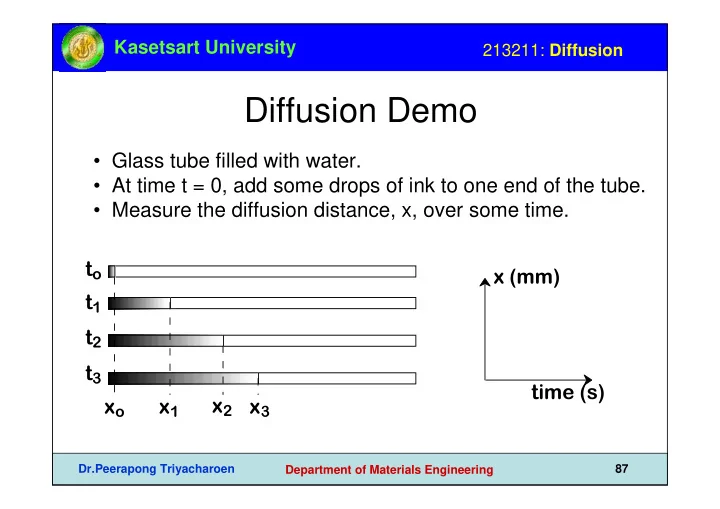
Kasetsart University 213211: Diffusion Diffusion Demo • Glass tube filled with water. • At time t = 0, add some drops of ink to one end of the tube. • Measure the diffusion distance, x, over some time. t o x (mm) t 1 t 2 t 3 time (s) x 2 x o x 1 x 3 Dr.Peerapong Triyacharoen 87 Department of Materials Engineering

Kasetsart University 213211: Diffusion Diffusion: The Phenomena • Interdiffusion: In an alloy, atoms tend to migrate from regions of large concentration. Cu-Ni Diffusion Couple Initially After some time Cu Ni 100% 100% 0 0 Concentration Profiles Concentration Profiles Dr.Peerapong Triyacharoen 88 Department of Materials Engineering

Kasetsart University 213211: Diffusion Diffusion: The Phenomena (con.) • Self-diffusion: In an elemental solid, atoms also migrate. Label some atoms After some time C C D A A D B B Dr.Peerapong Triyacharoen 89 Department of Materials Engineering

Kasetsart University 213211: Diffusion Diffusion Mechanisms (1) Vacancy or Substitutional Diffusion: • applies to substitutional impurities • atoms exchange with vacancies • rate depends on: --number of vacancies --activation energy to exchange. increasing elapsed time Dr.Peerapong Triyacharoen 90 Department of Materials Engineering

Kasetsart University 213211: Diffusion Activation Energy Dr.Peerapong Triyacharoen 91 Department of Materials Engineering

Kasetsart University 213211: Diffusion Diffusion Mechanisms (2) Interstitial Diffusion: • atoms migrate from an interstitial position to a neighboring one that is empty, ex. H, O, C, N atoms Dr.Peerapong Triyacharoen 92 Department of Materials Engineering

Kasetsart University 213211: Diffusion Processing using Diffusion • Case Hardening: --Diffuse carbon atoms into the host iron atoms at the surface. --Example of interstitial diffusion is a case hardened gear. • Result: The "Case" is --hard to deform: C atoms "lock" planes from shearing. --hard to crack: C atoms put the surface in compression. Dr.Peerapong Triyacharoen 93 Department of Materials Engineering

Kasetsart University 213211: Diffusion Modeling Diffusion: Flux • Flux: rate of mass transfer ⎡ ⎤ ⎡ ⎤ J = 1 dM kg ⎥ or atoms dt ⇒ ⎢ ⎥ ⎢ A m 2 s m 2 s ⎣ ⎦ ⎣ ⎦ x-direction • Flux can be measured for : --vacancies --host (A) atoms Unit area A y Jy --impurity (B) atoms through which Jx • Directional Quantity Jz x atoms z move. Dr.Peerapong Triyacharoen 94 Department of Materials Engineering

Kasetsart University 213211: Diffusion Concentration Profiles & Flux • Concentration Profile, C(x): [kg/m 3 ] Cu flux Ni flux Concentration Concentration of Cu [kg/m3] of Ni [kg/m3] Position, x Fick's First Law: Diffusion coefficient [m2/s] flux in x-dir. J x = − D dC [kg/m2-s] concentration dx gradient [kg/m4] The steeper the concentration profile, the greater the flux! Dr.Peerapong Triyacharoen 95 Department of Materials Engineering

Kasetsart University 213211: Diffusion Steady-State Diffusion • Steady State: the concentration profile doesn't change with time. Steady State: Jx(left) Jx(right) Jx(left) = Jx(right) x Concentration, C, in the box doesn’t change w/time. J x = − DdC Apply Fick's First Law: dx ⎛ ⎞ ⎛ ⎞ dC = dC ⎜ ⎟ ⎜ ⎟ If J x ) left = J x ) right , then ⎝ ⎠ ⎝ ⎠ dx dx left right Result: the slope, dC/dx, must be constant! Dr.Peerapong Triyacharoen 96 Department of Materials Engineering

Kasetsart University 213211: Diffusion Steady-State Diffusion Dr.Peerapong Triyacharoen 97 Department of Materials Engineering

Kasetsart University 213211: Diffusion Ex. Steady State Diffusion A plate of iron is exposed to a carburizing atmosphere on one side and a decarburizing atmosphere on the other side at 700°C. 3 m / g k 2 Fick's First Law: 3 . 1 m = / − g k C 1 C C 8 . = − 0 A B = J D C 2 − Carbon x x Steady State = A B straight line! rich gas − Carbon 1 . 2 0 . 8 − = − × 11 J ( 3 10 ) deficient − − × − 3 2 5 10 10 gas D=3x10-11m2/s x1 x2 0 2 ⋅ − 9 = × 10mm kg/m s 5 J 2 . 4 10 m m Dr.Peerapong Triyacharoen 98 Department of Materials Engineering

Kasetsart University 213211: Diffusion Non Steady-State Diffusion dx • Concentration profile, C(x), J(left) J(right) changes w/ time. Concentration, C, in the box • To conserve matter: • Fick's First Law: J = − D dC J(right) − J(left) = − dC or dx dx dt = − D d2 C (if D does = − dC dJ dJ not vary dx 2 dt dx with x) dx equate dt = Dd 2 C dC Fick's Second Law • Governing Eqn.: dx 2 Dr.Peerapong Triyacharoen 99 Department of Materials Engineering

Kasetsart University 213211: Diffusion Case Study: Non Steady-State Diffusion • Copper diffuses into a bar of aluminum. Surface conc., bar Cs of Cu atoms pre-existing conc., Co of copper atoms C(x,t) Cs t3 to t1 t2 Co position, x ⎛ ⎞ C(x,t) − C o x = 1 − erf ⎜ ⎟ • General solution: ⎝ ⎠ C s − C o 2 Dt " error function " Values calibrated in Table 5.1, Callister 6e . Dr.Peerapong Triyacharoen 100 Department of Materials Engineering

Kasetsart University 213211: Diffusion Error Function Values z x 2 ∫ − 2 y dy = = where z erf ( z ) e π 2 Dt 0 Dr.Peerapong Triyacharoen 101 Department of Materials Engineering

Kasetsart University 213211: Diffusion Ex. Carburizing Process Steel: C o = 0.25 wt% C T = 950°C C s = 1.20 wt% C How long would it take to achieve a carbon content of 0.80 wt% C at a position 0.5 mm below the surface? ⎛ ⎞ − − − × 4 ⎜ ⎟ C C 0 . 80 0 . 25 5 10 = = − x o 1 erf ⎜ ⎟ − − ⎜ ⎟ − C C 1 . 20 0 . 25 × 11 x o ⎝ 2 ( 1 . 6 10 ) t ⎠ ⎛ ⎞ 62 . 5 ⎜ ⎟ = erf 0 . 4210 ⎜ ⎟ ⎝ ⎠ t − − z 0 . 35 0 . 4210 0 . 3794 z = 0.392 = − − 0 . 40 0 . 35 0 . 4282 0 . 3794 t = 25,400 s = 7.1 hr Dr.Peerapong Triyacharoen 102 Department of Materials Engineering

Kasetsart University 213211: Diffusion Ex. Non Steady-State Diffusion Copper diffuses into a bar of aluminum for 10 hours at 600°C and gives desired C(x). How many hours would it take to get the same C(x) if we processed at 500 ° C? Key point 1: C(x,t 500C ) = C(x,t 600C ). Key point 2: Both cases have the same C o and C s . ⎛ ⎞ C(x,t) − Co x (Dt) 500 ° C = (Dt) 600 ° C = 1 − erf ⎜ ⎟ ⎝ ⎠ C − Co 2Dt s 5.3x10- 13 m 2 /s 10hrs t 500 = (Dt) 600 = 110hr D 500 4.8x10- 14 m 2 /s Dr.Peerapong Triyacharoen 103 Department of Materials Engineering

Kasetsart University 213211: Diffusion Diffusion and Temperature • Diffusivity increases with T. pre-exponential [m2/s] (see Table 5.2, Callister 6e ) activation energy ⎛ ⎞ [J/mol],[eV/mol] Qd ⎜ ⎟ D = Doexp − diffusivity (see Table 5.2, Callister 6e ) ⎝ ⎠ RT gas constant [8.31J/mol-K] • Experimental Data: 1500 1000 600 300 T(C) D has exp. dependence on T 10 -8 C Recall: Vacancy does also! i n γ D (m2/s) - C F i e n Dinterstitial >> Dsubstitutional α - F e Cu in Cu C in α -Fe 10- 14 Z n Al in Al F i C in γ -Fe A n e l C Fe in α -Fe F i n i Cu in Cu u n e A Fe in γ -Fe i γ n l - F α e Zn in Cu - F 10 -20 e 1000K/T 0.5 1.0 1.5 2.0 Dr.Peerapong Triyacharoen 104 Department of Materials Engineering

Kasetsart University 213211: Diffusion Diffusion Data Dr.Peerapong Triyacharoen 105 Department of Materials Engineering

Kasetsart University 213211: Diffusion Ex. Carburizing Process 2 C o = 0.20 wt% C s = 1.00 wt% Need C x = 0.60 wt% at 0.75 mm below surface Specify an appropriate heat treatment in terms of temperature and time for temperature between 900 ° C and 1050 ° C. ⎛ ⎞ − − C C 0 . 60 0 . 20 x x ⎜ ⎟ = = − = x o Table 5.1 = 0 1 erf 0 . 5 ⎜ ⎟ 0 . 4747 − − 1 . 0 0 . 25 ⎝ ⎠ C C 2 Dt 2 Dt x o x = 7.5x10 -4 m Dt = 6.24x10 -7 m 2 Time T (°C) ⎡− ⎤ 148 , 000 − − s hr × 5 = × 7 ( 2 . 3 10 ) exp 6 . 24 10 ⎢ ⎥ ⎣ ⎦ 8 . 31 T 900 106,400 29.6 0 . 0271 950 57,200 15.9 = ( in t s ) ⎛− ⎞ 17 , 810 1000 32,300 9.0 ⎜ ⎟ exp ⎝ ⎠ 1050 19,000 5.3 T Dr.Peerapong Triyacharoen 106 Department of Materials Engineering

Kasetsart University 213211: Diffusion Summary: Structure & Diffusion Diffusion FASTER for... Diffusion SLOWER for... • open crystal structures • close-packed structures • lower melting T materials • higher melting T materials • materials w/secondary • materials w/covalent bonding bonding • smaller diffusing atoms • larger diffusing atoms • cations • anions • lower density materials • higher density materials Dr.Peerapong Triyacharoen 107 Department of Materials Engineering