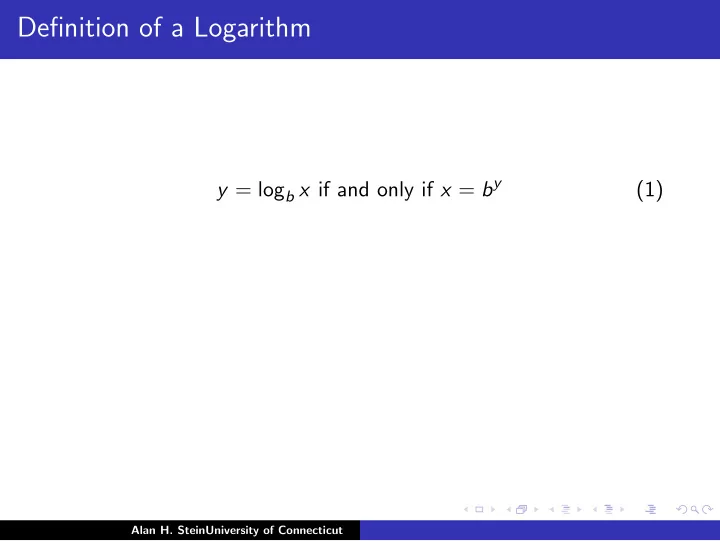
Definition of a Logarithm y = log b x if and only if x = b y (1) - PowerPoint PPT Presentation

Jan 25, 2024 •47 likes •147 views

Definition of a Logarithm y = log b x if and only if x = b y (1) Alan H. SteinUniversity of Connecticut Definition of a Logarithm y = log b x if and only if x = b y (1) Essentially, the logarithm to the base b of a number x is the power which

Definition of a Logarithm y = log b x if and only if x = b y (1) Alan H. SteinUniversity of Connecticut
Definition of a Logarithm y = log b x if and only if x = b y (1) Essentially, the logarithm to the base b of a number x is the power which b must be raised to in order to obtain x. Alan H. SteinUniversity of Connecticut
Definition of a Logarithm y = log b x if and only if x = b y (1) Essentially, the logarithm to the base b of a number x is the power which b must be raised to in order to obtain x. This immediately leads to the two very useful formulas b log b x = x Alan H. SteinUniversity of Connecticut
Definition of a Logarithm y = log b x if and only if x = b y (1) Essentially, the logarithm to the base b of a number x is the power which b must be raised to in order to obtain x. This immediately leads to the two very useful formulas b log b x = x and log b b x = x . (2) Alan H. SteinUniversity of Connecticut
Properties of Logarithms Each of the properties of exponential functions has an analog for logarithmic functions. Alan H. SteinUniversity of Connecticut
Properties of Logarithms Each of the properties of exponential functions has an analog for logarithmic functions. ◮ log b ( xy ) = log b x + log b y Alan H. SteinUniversity of Connecticut
Properties of Logarithms Each of the properties of exponential functions has an analog for logarithmic functions. ◮ log b ( xy ) = log b x + log b y ◮ log b ( x / y ) = log b x − log b y Alan H. SteinUniversity of Connecticut
Properties of Logarithms Each of the properties of exponential functions has an analog for logarithmic functions. ◮ log b ( xy ) = log b x + log b y ◮ log b ( x / y ) = log b x − log b y ◮ log b ( x r ) = r log b x Alan H. SteinUniversity of Connecticut
Properties of Logarithms
Properties of Logarithms Each of the properties of exponential functions has an analog for logarithmic functions. ◮ log b ( xy ) = log b x + log b y ◮ log b ( x / y ) = log b x − log b y ◮ log b ( x r ) = r log b x ◮ log b 1 = 0. In other words, The logarithm of a product or quotient is the sum or difference of logarithms and the logarithm of a number to a power is the power times the logarithm of that number. Alan H. SteinUniversity of Connecticut

Recommend

Definition of Natural Logarithm Function Recall x n dx = x n +1 n + 1 + C n = 1 .

Definition of Natural Logarithm Function Recall x n dx = x n +1 n + 1 + C n = 1 . What happens if n = 1? the above formula does not make sense if n = 1. However, since the function f ( x ) = x 1 is continuous on the

733 views • 5 slides

The Natural Logarithm Function and The Exponential Function One specific logarithm function is

The Natural Logarithm Function and The Exponential Function One specific logarithm function is singled out and one particular exponential function is singled out. Definition e = lim x 0 (1 + x ) 1 / x Alan H. SteinUniversity of Connecticut

627 views • 29 slides

The logarithm as an inverse function In this section we concentrate on understanding the logarithm

The logarithm as an inverse function In this section we concentrate on understanding the logarithm function. If the logarithm is understood as the inverse of the exponential function, then the properties of logarithms will naturally follow from

238 views • 8 slides

Logarithms 2-1 Definition of Logarithms

Logarithms 2-1 Definition of Logarithms If x = b y then y = log b x where x and b are positive numbers and b 1 log b x is read as logarithm of x to the base of b . 2 - 2

318 views • 9 slides

Law of the iterated logarithm for pure jump L evy processes Elena Shmileva, St.Petersburg

Outline Two types of the Law of the Iterated Logarithm Generalization of the LILs. Functional LIL My results on the Functional LIL for S S processes Conclusion Law of the iterated logarithm for pure jump L evy processes Elena Shmileva,

467 views • 21 slides

Solving the Discrete Logarithm of a 113-bit Koblitz Curve with an FPGA Cluster Erich Wenger and

Solving the Discrete Logarithm of a 113-bit Koblitz Curve with an FPGA Cluster Erich Wenger and Paul Wolfger Graz University of Technology WECC 2014, Chennai, India We solved the discrete logarithm of a 113-bit Koblitz Curve.

572 views • 34 slides

Discrete logarithm problem for matrices over finite group rings Alex Myasnikov Stevens Institute

Discrete logarithm problem for matrices over finite group rings Alex Myasnikov Stevens Institute of Technology Discrete logarithm problem. The discrete logarithm problem (DLP) in a finite cyclic group G : for any pair g , h G find a

673 views • 18 slides

On the discrete logarithm problem in elliptic curves Claus Diem University of Leipzig On the

On the discrete logarithm problem in elliptic curves Claus Diem University of Leipzig On the discrete logarithm problem in elliptic curves p.1/37 Some history At ECC 2004 in Bochum, Pierrick Gaudry presented an index calculus algorithm

897 views • 67 slides

A kilobit hidden SNFS discrete logarithm computation ia.cr/2016/961 (Eurocrypt 2017) J. Fried 1 ,

A kilobit hidden SNFS discrete logarithm computation ia.cr/2016/961 (Eurocrypt 2017) J. Fried 1 , P. Gaudry 2 , N. Heninger 1 , E. Thom e 2 1 U. Penn ; 2 Caramba/Inria/Loria Nov 13rd, 2017 A kilobit hidden SNFS discrete logarithm computation

441 views • 42 slides

MATH 12002 - CALCULUS I 5.2: The Natural Logarithm Professor Donald L. White Department of

MATH 12002 - CALCULUS I 5.2: The Natural Logarithm Professor Donald L. White Department of Mathematical Sciences Kent State University D.L. White (Kent State University) 1 / 5 The Natural Logarithm Function Recall that for n = 1,

541 views • 5 slides

How logarithm laws may fail in a mixing system: an example with a reparametrization of a

How logarithm laws may fail in a mixing system: an example with a reparametrization of a translation on the torus Pietro Peterlongo (joint work with Stefano Galatolo) PhD student at Scuola Normale, Pisa and Ecole Normale, Paris (Laboratoire de

380 views • 18 slides

Base-2 Logarithms If n = 2 k then k is called the logarithm (base 2) of n n=2 k k log 10 (n)

Base-2 Logarithms If n = 2 k then k is called the logarithm (base 2) of n n=2 k k log 10 (n) binary rep. of n 0 1 0 00000000000000001 1 2 1 00000000000000010 2 4 2 00000000000000100 3 8 3 00000000000001000 4 16 4

212 views • 3 slides

The Natural Logarithm Function x n dx = x n +1 Problem: The formula n + 1 + c has one problem

The Natural Logarithm Function x n dx = x n +1 Problem: The formula n + 1 + c has one problem it doesnt hold for n = 1. 1 On the other hand, we know from the Fundamental Theorem of Calculus that x dx exists everywhere except at

262 views • 10 slides

Confidence Bands for Distribution Functions: The Law of the Iterated Logarithm and Shape

Confidence Bands for Distribution Functions: The Law of the Iterated Logarithm and Shape Constraints Lutz Duembgen (Bern) Jon A. Wellner (Seattle) Petro Kolesnyk (Bern) Ralf Wilke (Copenhagen) November 2014 I. The LIL for Brownian Motion and

521 views • 49 slides

Comparing the Difficulty of Factorization and Discrete Logarithm: a 240-digit Experiment Solving

Comparing the Difficulty of Factorization and Discrete Logarithm: a 240-digit Experiment Solving RSA-240, DLP-240, RSA-250 Fabrice Boudot, Pierrick Gaudry, Aurore Guillevic, Nadia Heninger, Emmanuel Thom e, Paul Zimmermann ere de l FB:

466 views • 31 slides

Discrete Logarithm in GF(2 809 ) with FFS Razvan Barbulescu Cyril Bouvier J er emie Detrey

Discrete Logarithm in GF(2 809 ) with FFS Razvan Barbulescu Cyril Bouvier J er emie Detrey Pierrick Gaudry Hamza Jeljeli Emmanuel Thom e Marion Videau Paul Zimmermann CARAMEL project-team, LORIA, INRIA / CNRS / Universit e de

419 views • 22 slides

Motivation Given an elliptic curve E over a finite field F q . Is the Discrete Logarithm Problem

Counting points on elliptic curves over F q Christiane Peters DIAMANT-Summer School on Elliptic and Hyperelliptic Curve Cryptography September 17, 2008 Motivation Given an elliptic curve E over a finite field F q . Is the Discrete Logarithm

587 views • 30 slides

The characteristic function for infinite Jacobi matrices, its logarithm, the spectral zeta

The characteristic function for infinite Jacobi matrices, its logarithm, the spectral zeta function, and solvable examples Frantiek tampach 1 , Pavel tov cek 2 1 Department of Applied Mathematics, Faculty of Information Technology

524 views • 30 slides

A game-theoretic proof of the Erdos-Feller-Kolmogorov-Petrowsky law of the iterated logarithm for

A game-theoretic proof of the Erdos-Feller-Kolmogorov-Petrowsky law of the iterated logarithm for fair-coin tossing Akimichi Takemura (U.Tokyo) (joint with Takeyuki Sasai and Kenshi Miyabe) Nov.13, 2014, CIMAT Mexico Manuscript: A

418 views • 25 slides

On the discrete logarithm problem in finite fields Pierrick Gaudry CNRS, Universit de

On the discrete logarithm problem in finite fields Pierrick Gaudry CNRS, Universit de Lorraine, Inria Nancy, France joint work with Razvan Barbulescu, Antoine Joux, Emmanuel Thom RICAM Linz, Austria 1/42 Plan Background Recent

598 views • 49 slides

A subfield-logarithm attack against ideal lattices, part 1: the number-field sieve D. J.

A subfield-logarithm attack against ideal lattices, part 1: the number-field sieve D. J. Bernstein University of Illinois at Chicago & Technische Universiteit Eindhoven Sieving small integers 0 using primes 2 3 5 7: 1

646 views • 42 slides

Fundamentalism Definition? Definition? Definition? Definition? Origins Conflict with

Fundamentalism Definition? Definition? Definition? Definition? Origins Conflict with Modernity Traditionalism Maximalism Inerrancy Inerrancy Sexual Politics non-free image :( Nationalism Is it a threat? Politics Violence Media

454 views • 20 slides

Generic attacks The discrete-logarithm problem and index calculus Define = 1000003. D. J.

Generic attacks The discrete-logarithm problem and index calculus Define = 1000003. D. J. Bernstein Easy to prove: is prime. University of Illinois at Chicago Can we find an integer 1 2 3

1.24k views • 97 slides

Discrete Logarithm with Auxiliary Inputs (Special Semester Workshop 4) Jung Hee Cheon (partly

Discrete Logarithm with Auxiliary Inputs (Special Semester Workshop 4) Jung Hee Cheon (partly joint work with Taechan Kim and Yongsu Song) Department of Mathematical Sciences and ISaC-RIM Seoul National University December 13, 2013 1 / 41

557 views • 41 slides