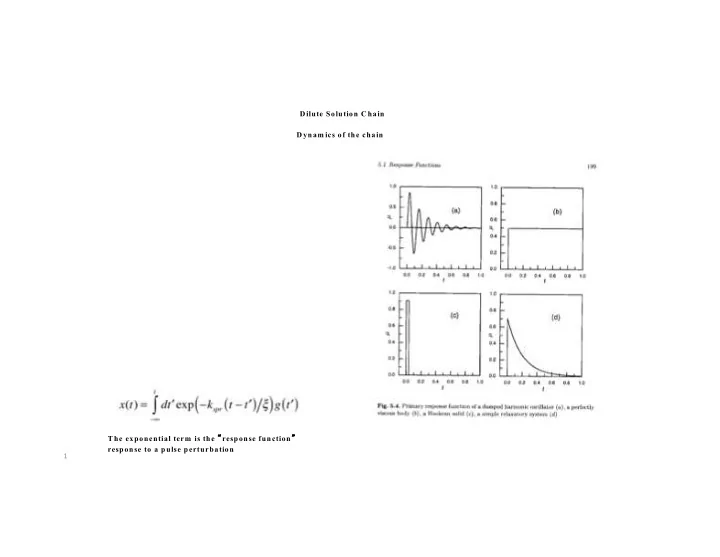
D ilute Solution C hain D ynam ics of the chain T he exponential - PowerPoint PPT Presentation

D ilute Solution C hain D ynam ics of the chain T he exponential term is the � response function � response to a pulse perturbation 1

D ilute Solution C hain D ynam ics of the chain D am ped H arm onic O scillator For Brow nian m otion of a harm onic bead in a solvent this response function can be used to calculate the tim e correlation function <x(t)x(0)> for DLS for instance τ is a relaxation tim e. 2

Draining vs Non Draining Rouse vs Zimm Consider Diffusion of a Chain D = kT/ z For Non Draining

Dilute Solution Chain Dynamics of the chain Rouse Motion Beads 0 and N are special For Beads 1 to N-1 For Bead 0 use R -1 = R 0 and for bead N R N+1 = R N This is called a closure relationship 5

Dilute Solution Chain Dynamics of the chain Rouse Motion The Rouse unit size is arbitrary so we can make it very small and: With dR/dt = 0 at i = 0 and N Reflects the curvature of R in i, it describes modes of vibration like on a guitar string 6

Dilute Solution Chain Dynamics of the chain Rouse Motion Describes modes of vibration like on a guitar string For the � p � th � mode (0 � th mode is the whole chain (string)) 7

x, y, z decouple (are equivalent) so you can just deal with z dz l ς R dt = b R ( z l + 1 − z l ) + b R ( z l − 1 − z l ) For a chain of infinite molecular weight there are wave solutions to this series of differential equations ⎛ ⎞ z l ~ exp − t ( ) ⎟ exp il δ ⎜ ⎝ ⎠ τ sin 2 δ τ − 1 = b R ) = 4 b R ( 2 − 2cos δ ζ R ζ R 2 8

sin 2 δ τ − 1 = b R ) = 4 b R ( 2 − 2cos δ For N R = 10 ζ R ζ R 2 z l = z l + N R Cyclic Boundary Conditions: N R δ = m 2 π N R values of phase shift δ m = 2 π ⎛ ⎞ m ; m = − N R ⎟ ,..., N R 2 − 1 ⎜ ⎝ ⎠ N R 2 9

sin 2 δ τ − 1 = b R ) = 4 b R ( 2 − 2cos δ For N R = 10 ζ R ζ R 2 z l − z 0 = z N R − 1 − z N R − 2 = 0 Free End Boundary Conditions: dz ) = dz ( ( ) = 0 dl l = 0 dl l = N R − 1 ( ) δ = m π N R − 1 N R values of phase shift π ( ) δ m = m ; m = 0,1,2,..., N R − 1 ( ) N R Rouse Modes of order “m” N R − 1 1 0

Lowest order relaxation time dominates the response ⎛ ⎞ ζ R ⎜ ⎟ ⎝ ⎠ 2 a R 1 τ R = 4 R 0 3 π 2 kT ⎛ ζ R ⎞ This assumes that ⎜ ⎟ ⎝ ⎠ 2 a R is constant, friction coefficient is proportional to number of monomer units in a Rouse segment This is the basic assumption of the Rouse model, 2 ~ N ζ R ~ a R = n R N R 1 1

Lowest order relaxation time dominates the response ⎛ ⎞ ζ R ⎜ ⎟ ⎝ ⎠ 2 a R 1 τ R = 4 R 0 3 π 2 kT 2 = a 0 2 N Since R 0 τ R ~ N 2 kT 1 2

The amplitude of the Rouse modes is given by: 2 2 = 2 R 0 Z m 3 π 2 m 2 The amplitude is independent of temperature because the free energy of a mode is proportional to kT and the modes are distributed by Boltzmann statistics ⎛ ⎞ ) = exp − F ( p Z m ⎜ ⎟ ⎝ ⎠ kT 90% of the total mean-square end to end distance of the chain originates from the lowest order Rouse-modes so the chain can be often represented as an elastic dumbbell 1 3

Rouse dynamics (like a dumbell response) Dumbbell Rouse ⎛ ⎞ dU ⎜ ⎟ ζ R ⎝ ⎠ + g ( t ) = − k spr x τ R = dx dx dt = − + g ( t ) 4 b R sin 2 δ ζ ζ 2 π dt 'exp − t − t ' t ⎛ ⎞ ( ) = ( ) ∫ δ = N R − 1 m , m=0,1,2,...,N R -1 ⎜ ⎟ x t g t ⎝ ⎠ τ −∞ τ = ζ k spr 1 4

Rouse dynamics (like a dumbell response) ( ) g t 2 ( ) = 2 D δ t ( ) where t = t 1 − t 2 and δ ( ) is the delta function whose integral is 1 g t 1 D = kT Also, ζ ⎛ ⎞ kT exp − t ⎜ ⎟ τ = ζ x 2 = kT ⎝ ⎠ τ ( ) x 0 ( ) = For t => 0, x t k spr k spr k spr 1 5

Predictions of Rouse Model − 1 ( ) ~ t G t 2 1 ( ) ( ) ~ ωη 0 G ' ω 2 π 2 η 0 = kT ρ p τ R 12 ~ N 1 6

Dilute Solution Chain Dynamics of the chain Rouse Motion Predicts that the viscosity will follow N which is true for low molecular weights in the melt and for fully draining polymers in solution Rouse model predicts Relaxation time follows N 2 (actually follows N 3 /df) Diffusion constant follows 1/N (zeroth order mode is translation of the molecule) (actually follows N -1/df ) Both failings are due to hydrodynamic interactions (incomplete draining of coil) 1 7

Dilute Solution Chain Dynamics of the chain Rouse Motion Predicts that the viscosity will follow N which is true for low molecular weights in the melt and for fully draining polymers in solution Rouse model predicts Relaxation time follows N 2 (actually follows N 3 /df) 1 8