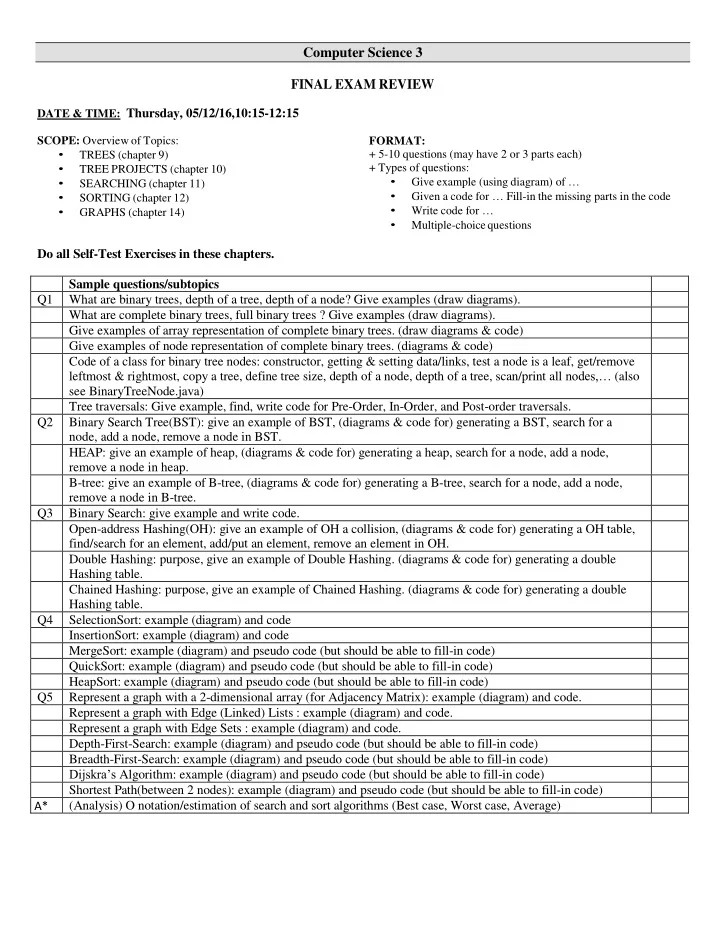

Computer Science 3 FINAL EXAM REVIEW DATE & TIME: Thursday, 05/12/16,10:15-12:15 SCOPE: Overview of Topics: FORMAT: • TREES (chapter 9) + 5-10 questions (may have 2 or 3 parts each) • + Types of questions: TREE PROJECTS (chapter 10) • Give example (using diagram) of … • SEARCHING (chapter 11) • Given a code for … Fill-in the missing parts in the code • SORTING (chapter 12) • Write code for … • GRAPHS (chapter 14) • Multiple-choice questions Do all Self-Test Exercises in these chapters. Sample questions/subtopics Q1 What are binary trees, depth of a tree, depth of a node? Give examples (draw diagrams). What are complete binary trees, full binary trees ? Give examples (draw diagrams). Give examples of array representation of complete binary trees. (draw diagrams & code) Give examples of node representation of complete binary trees. (diagrams & code) Code of a class for binary tree nodes: constructor, getting & setting data/links, test a node is a leaf, get/remove leftmost & rightmost, copy a tree, define tree size, depth of a node, depth of a tree, scan/print all nodes,… (also see BinaryTreeNode.java) Tree traversals: Give example, find, write code for Pre-Order, In-Order, and Post-order traversals. Q2 Binary Search Tree(BST): give an example of BST, (diagrams & code for) generating a BST, search for a node, add a node, remove a node in BST. HEAP: give an example of heap, (diagrams & code for) generating a heap, search for a node, add a node, remove a node in heap. B-tree: give an example of B-tree, (diagrams & code for) generating a B-tree, search for a node, add a node, remove a node in B-tree. Q3 Binary Search: give example and write code. Open-address Hashing(OH): give an example of OH a collision, (diagrams & code for) generating a OH table, find/search for an element, add/put an element, remove an element in OH. Double Hashing: purpose, give an example of Double Hashing. (diagrams & code for) generating a double Hashing table. Chained Hashing: purpose, give an example of Chained Hashing. (diagrams & code for) generating a double Hashing table. Q4 SelectionSort: example (diagram) and code InsertionSort: example (diagram) and code MergeSort: example (diagram) and pseudo code (but should be able to fill-in code) QuickSort: example (diagram) and pseudo code (but should be able to fill-in code) HeapSort: example (diagram) and pseudo code (but should be able to fill-in code) Q5 Represent a graph with a 2-dimensional array (for Adjacency Matrix): example (diagram) and code. Represent a graph with Edge (Linked) Lists : example (diagram) and code. Represent a graph with Edge Sets : example (diagram) and code. Depth-First-Search: example (diagram) and pseudo code (but should be able to fill-in code) Breadth-First-Search: example (diagram) and pseudo code (but should be able to fill-in code) Dijskra’s Algorithm: example (diagram) and pseudo code (but should be able to fill-in code) Shortest Path(between 2 nodes): example (diagram) and pseudo code (but should be able to fill-in code) A* (Analysis) O notation/estimation of search and sort algorithms (Best case, Worst case, Average)
Sample Final Exam: 1) Fill-in the code for function treeCopy to copy a binary tree. public class BinaryTreeNode{ public int data; public BinaryTreeNode left, right; public BinaryTreeNode(int initialData, BinaryTreeNode initialLeft, BinaryTreeNode initialRight) { data = initialData; left = initialLeft; right = initialRight; } public static BinaryTreeNode treeCopy(BinaryTreeNode source) { BinaryTreeNode leftCopy, rightCopy; if (source == null) return null; else { leftCopy = treeCopy(source.left); //FILL-IN HERE ... return // FILL_IN HERE ... } } } 2) Draw a diagram to give an example of a Heap of 9 nodes(show node values) and its array representation. 3) Draw a diagram to give an example of a Hash function for a Hash table of size 5, a list of 5 data, and its Chained Hashing table. 4) Write code for function SelectSort(int[] A, int N) which sorts array A using SelectionSort method and returns the sorted array. 5) Given a Graph(p. 722), show order of visited nodes using Breadth-First-Search
• EXAMPLE of QUESTIONS: 1) Given a heap: ADD a node with value 111, draw a new heap: REMOVE the root , draw a new heap: IF we use ARRAY IMPLEMENTATION for heaps(complete binary trees): For the given above heap: index 0 1 2 3 4 5 6 7 8 9 10 11 12 value 100 90 91 17 26 45 1 2 7 3 19 25 36 EXAMPLE of (advanced) QUESTIONS: 2) GIVEN a heap with array implementation: index 0 1 2 3 4 5 6 7 8 9 10 11 12
value 100 90 91 17 26 45 1 2 7 3 19 25 36 ADD a node with value 95, find the NEW array contents: (THEN, we’ll need to draw) (ADD using the graph, we’ll have a NEW GRAPH) index 0 1 2 3 4 5 6 7 8 9 10 11 12 13 value 100 90 95 17 26 45 91 2 7 3 19 25 36 1 3)GIVEN a heap with array implementation: index 0 1 2 3 4 5 6 7 8 9 10 11 12 13 value 100 90 95 17 26 45 91 2 7 3 19 25 36 1 REMOVE the ROOT, find the NEW array contents: (THEN, we’ll need to draw) REVOME using the graph, we’ll have a NEW GRAPH)
index 0 1 2 3 4 5 6 7 8 9 10 11 12 13 value 95 90 91 17 26 45 1 2 7 3 19 25 36 4) Given a set of data {2,7,26,25,19,17} build/draw the HEAP/graph using array implementation: Initially: index 0 1 2 3 4 5 value 2 7 26 125 19 17 THEN, we’ll have to do DOWNWARD HEAPIFICATION: AFTER that we’ll have our HEAP: index 0 1 2 3 4 5 value 26 25 17 7 19 2 5) WHICH of the following trees are HEAP or BST ? a)
b) c) d) e)
List Complete Binary Trees: a,b,d List NON-Complete BT: c,e List HEAP: b,d List BST:c 6) BINARY SEARCH TREE: ADD a node: (55) BEFORE: AFTER: REMOVE a node:
Add 71 back: EXAMPLES of QUESTIONS: 7)Given a BST (below). This is was created by adding a node at a time from a list. Define that list {X,X,X,X,X}. a) ANSWER: the list = {49,9,93,13,52} b)
ANSWER: the list = {13,9,49,52,93} ANOTHER answer: = 13,49,52,9,93 ANOTHER answer: = 13,49,9,52,93 ANOTHER answer: = 13,49,52,93,9 Hashing Method: 8) EXAMPLE: Assume that we need to store the 10 dates of the month (from 1 to 31), for example {4,12,10,5,24,15,30,22,20,14}. Build a Hash table for these data in the following cases: a) Use MIN memory b) With MIN # of collisions (fast) c) Using Double Hash d) Using Linked List (open hashing(=close addressing)), chaining hashing ) a)Use MIN memory Hash function is MOD-10(x) => hash values [0-9] [0] [1] [2] [3] [4] [5] [6] [7] [8] [9] 10 30 12 22 4 5 24 15 20 14 24 => collision => WHICH methods (of probing) to use ? Linear Double hashing Linked
24,15 gives 2 collisions 15,30, gives 1 20 give 8 14 give 5 TOTAL = 19 collisions b) With MIN # of collisions (fast) {4,12,10,5,24,15,30,22,20,14}. DATA range is [1,31] => how many different inputs/data ? 31 => Hash function is MOD-31(x) => hash values [0-30] => Hash TABLE ? size =31 [0] [1] [2] [3] [4] [5] [6] [7] [8] [9] 4 5 [10] [11] [12] [13] [14] [15] [16] [17] [18] [19] 10 12 14 15 [20] [21] [22] [23] [24] [25] [26] [27] [28] [29] 20 22 24 [30] 30 TOTAL # of collisions ? = 0 WHAT IF we have to ADD 31 => where it will be ? MOD 31 (31)=0
Recommend
More recommend