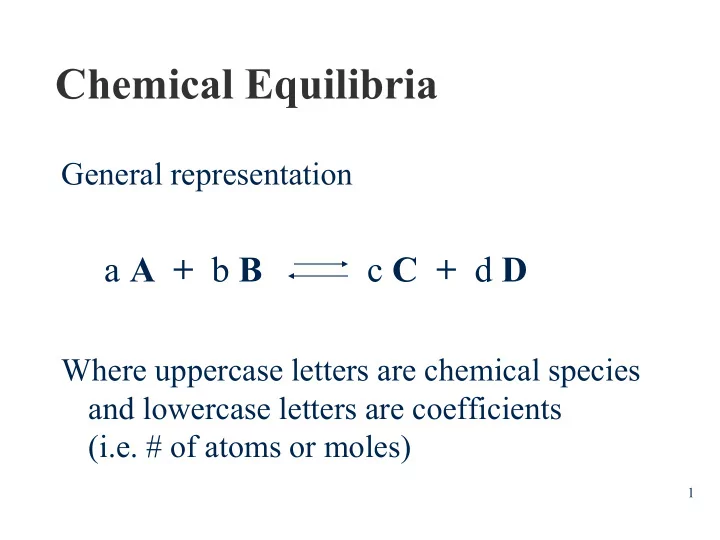

Chemical Equilibria General representation a A + b B c C + d D Where uppercase letters are chemical species and lowercase letters are coefficients (i.e. # of atoms or moles) 1
True Thermodynamic Equilibrium Constant o (a C ) c (a D ) d K = ---------------- (a A ) a (a B ) b For a A + b B c C + d D K o Defined for standard conditions of 25 o C, 1 atm pressure and I = 0 (infinite dilution) 2
Equilibrium Constant [C] c [D] d K = --------------- [A] a [B] b where [ ] = concentration, usually molar 3
Many types of K’s (equilibrium constants) K a for acid dissociation K b for base hydrolysis K w for water auto ionization K sp for solubility product K f for a formation constant K 1 , K 2 , K 3 , etc. for stepwise formation constants β 1 , β 2 , β 3 , etc. for overall formation constants 4
Solubility Equilibria Ba 2+ 2- (aq) + SO 4 BaSO 4(s) (aq) or by convention BaSO 4(s) Ba 2+ (aq) + SO 4 2- (aq) We can write an equilibrium constant for rxn 5
Solubility Product (equilibrium constant) [Ba 2+ ] [SO 42- ] K sp = ------------------ = [Ba 2+ ] [SO 42- ] 1 a Ba a SO4 sp = ------------------ = a Ba a K SO4 1 activity of solid is defined as = 1 6
Solubility Calculated Solubility (S) is the concentration of individual ions generated from an insoluble compound Ba 2+ 2- BaSO 4(s) (aq) + SO 4 (aq) S = [Ba 2+ ] = [SO 4 2- ] 7
Solubility Calculation (continued) K SP = [Ba 2+ ][SO 4 2- ] = 2.0 x 10 -10 Given Then S = √ K SP = √ 2.0 x 10 -10 = 1.4 x 10 -5 M S = [Ba 2+ ] = [SO 4 2- ] = 1.4 x 10 -5 M So 8
Activity Correction a Ba a SO4 K SP = --------------- = a Ba a SO4 1 Since a Ba = γ Ba [Ba 2+ ] & a SO4 = γ SO4 [SO 4 2- ] Substituting K SP = a Ba a SO4 = γ Ba [Ba 2+ ] γ SO4 [SO 4 2- ] 9
Solubility Calculation (completed) Since K SP = γ Ba [Ba 2+ ] γ SO4 [SO 4 2- ] & γ Ba = γ SO4 K SP Then S = ------- √ γ 2 To determine solubility of BaSO 4 in a solution containing other ions (as in SW), you must calculate the activity coefficient ( γ ) 10
Two ways to correct for activity 1) Correct each ion as discussed K SP = a Ba a SO4 = γ Ba [Ba 2+ ] γ SO4 [SO 4 2- ] 2) Correct the equilibrium constant K K SP K ´ = --------- = [Ba 2+ ] [SO 4 2- ] γ 2 11
Common Ion Effect In seawater the total concentration of sulfate is 2.86 x 10 -2 moles/kg must use here ↓ K SP = a Ba a SO4 = γ Ba [Ba 2+ ] γ SO4 [SO 4 2- ] K SP K ´ = --------- = [Ba 2+ ] [SO 4 2- ] γ 2 12
Water Hydrolysis (very important) H 2 O H + + OH - Applying same rules for K expressions a H+ a OH- K w = -------------- = a H+ a OH- 1 Where H 2 O (the solvent) is assigned activity = 1 13
Remember pH pH is defined as the negative logarithm of the hydrogen ion activity pH = -log a H+ Given the numerical value K w = 1 x 10 -14 & K w = a H+ a OH- then we can always calculate OH - from the pH 14
pH Examples At neutral pH a H+ = a OH- and a H+ = √ K w = 1 x 10 -7 = pH 7.00 At seawater pH (e.g., 8.2) a H+ = 1 x 10 -8.2 = 6.31 x 10 -9 M K w 1 x 10 -14 a OH- = -------- = ------------ = 1.58 x 10 -6 M a H+ 6.31 x 10 -9 15
Hydronium Ion Water actually hydrolyses to form a hydronium ion (H 3 O + ) rather than the lone proton (H + ) (Once again an ion-water interaction akin to those discussed previously) For the sake of simplicity, we will refer to this species as H + which is common practice 16
A Note on Strong & Weak Electrolytes Salts, Acids & Bases are all ionic compounds that dissociate (i.e., form ions) in water either partially or completely Complete dissociation = a strong electrolyte NaCl H 2 O Na + + Cl - no equilibrium Partial dissociation = a weak electrolyte H + + HCO 3 H 2 CO 3 - K a1 H + + CO 3 HCO 3 - 2- K a2 Two step equilibrium = forward & back reactions 17
Acid-Base Equilibria Fictitious Weak Acid (HA) H + + A - HA [H + ] [A-] a H+ a A- K a = -------------- or -------------- [HA] a HA The smaller the K a the weaker the acid Strong acids have no K a it approaches infinity 18
Acid-Base Equilibria Fictitious Weak Base (B) Capable of accepting a proton (H + ) BH + + OH - B + H 2 O [BH + ] [OH - ] a BH+ a OH- K b = ----------------- or ------------------ [B] a B The smaller the K b the weaker the base Strong bases have no K b it approaches infinity 19
Ion Pair or Complex Formation Equilibria Dozens of Ion Pairs form in SW & even more complexes – deal with them the same way Mg 2+ (aq) + SO 4 2- MgSO 4(aq) (aq) a MgSO 4 K f = ---------------- a Mg a SO4 Larger K f = stronger formation – reaction 20
Typical Problem in SW Find Various Forms or Species Given total concentration data for certain constituents in SW, find % of species Example:If total Mg is C Mg = 5.28 x 10 -2 mol/kg and total SO 4 is C SO4 = 2.82 x 10 -2 mol/kg knowing that Mg 2+ (aq) + SO 4 2- MgSO 4(aq) (aq) and the value of the K f or K MgSO4 = 2.29 x 10 2 21
Steps in the Manual Solution of Simple Equilibrium Problems 1) Start with a recipe: C Mg = 5.28 x 10 -2 mol/kg C SO4 = 2.82 x 10 -2 mol/kg 2) List the species: Mg 2+ , SO 4 2- , MgSO 4 3) List reaction(s): Mg 2+ + SO 4 2- MgSO 4 4) Write Mass Balance equations: C Mg = [Mg 2+ ] + [MgSO 4 ] = 5.28 x 10 -2 mol/kg 2- ] + [MgSO 4 ] = 2.82 x 10 -2 mol/kg C SO4 = [SO 4 22
Steps in the Manual Solution of Simple Equilibrium Problems 5) Write a Charge Balance equation: Σ Z i+ [i + ] = Σ Z i- [i - ] 6) Write equilibrium constant expression(s): a MgSO 4 [MgSO 4 ] K f = ----------------- or ------------------- a Mg a SO 4 [Mg 2+ ] [SO 4 2- ] There are 3 species or 3 unknown concentrations There are also 3 equations (actually 4) to solve 23
We can solve the 3 equations simultaneously to get an answer Solve for free Mg concentration first = [Mg 2+ ] Rearrange the mass balance equations: C Mg = [Mg 2+ ] + [MgSO 4 ] rearranges to give [MgSO 4 ] = C Mg - [Mg 2+ ] C SO4 = [SO 4 2- ] + [MgSO 4 ] rearranges giving [SO 4 2- ] = C SO4 - [MgSO 4 ] We must also substitute the 1st into the 2nd 24
25
C Mg = [Mg 2+ ] + [MgSO 4 ] rearranges to give [MgSO 4 ] = C Mg - [Mg 2+ ] C SO4 = [SO 4 2- ] + [MgSO 4 ] rearranges giving [SO 4 2- ] = C SO4 - [MgSO 4 ] Substituting the 1 st into the 2 nd for [MgSO 4 ] Gives [SO 4 2- ] = C SO4 - (C Mg - [Mg 2+ ]) Now we can [MgSO 4 ] K f = ------------------- 2- ] Substitute into K [Mg 2+ ] [SO 4 26
Our resulting equation looks like C Mg - [Mg 2+ ] K MgSO4 = ---------------------------------------- [Mg 2+ ] (C SO4 - (C Mg - [Mg 2+ ])) Be careful of signs in denomenator C Mg - [Mg 2+ ] K MgSO4 = ---------------------------------------- [Mg 2+ ] (C SO4 - C Mg + [Mg 2+ ]) Cast in the form of a quadratic K[Mg 2+ ]C SO4 - K[Mg 2+ ]C Mg + K[Mg 2+ ] 2 = C Mg - [Mg 2+ ] Set equal to zero and solve with the quadratic formula 27
Equation from previous slide K[Mg 2+ ]C SO4 - K[Mg 2+ ]C Mg + K[Mg 2+ ] 2 = C Mg - [Mg 2+ ] Set equal to 0 & rearrange in form for quadratic formula K[Mg 2+ ] 2 + K[Mg 2+ ]C SO4 - K[Mg 2+ ]C Mg + [Mg 2+ ] - C Mg = 0 Gather terms K[Mg 2+ ] 2 + (KC SO4 - KC Mg + 1)[Mg 2+ ] - C Mg = 0 Remember the quadratic formula ? 28
Equation from previous slide K[Mg 2+ ] 2 + (KC SO4 - KC Mg + 1)[Mg 2+ ] - C Mg = 0 Quadratic formula - b + √ b 2 - 4 a c x = ------------------------ 2 a Solve for x which for us is [Mg 2+ ] where a = K b = (KC SO4 - KC Mg + 1) c = - C Mg 29
Solving this problem with the quadratic formula And substituting in the known values for: K f ´ which equals K f γ 2 Where K f = K MgSO4 = 2.29 x 10 2 and γ = 0.23 C Mg = 5.28 x 10 -2 mol/kg C SO4 = 2.82 x 10 -2 mol/kg The answer is: x = [Mg 2+ ] = 4.35 x 10 -2 mol/kg Since C Mg = 5.28 x 10 -2 mol/kg then [Mg 2+ ] = 82 % 30
Activity Coefficient At typical ionic strengths for SW I = 0.5 to 0.7 From Davies Equation Mg 2+ activity coefficient ln γ = - A Z 2 [I 0.5 /(1 + I 0.5 ) – 0.2 I] If Z = 2 & A = 1.17 then ln γ = - 1.47 & γ = 0.23 31
Calculate All Species Given C Mg = 5.28 x 10 -2 mol/kg and C SO4 = 2.82 x 10 -2 mol/kg We calculated [Mg 2+ ] = 4.35 x 10 -2 mol/kg or 82 % By difference [MgSO 4 ] = 9.30 x 10 -3 mol/kg or 18 % 2- ] concentration & % We can likewise calculate [SO 4 2- ] = 1.89 x 10 -2 mol/kg C SO4 - [MgSO 4 ] = [SO 4 32
Problems Went through a moderately difficult calculation & only calculated species for 1 reaction in SW If considered more complicated equilibria where several reactions were going on, the math would quickly get out of hand Didn’t consider any other reactions involving Mg or SO 4 that might influence our results 33
Other Problems Equilibrium constants can vary as much as 5% depending on the source Concentration data vary as well Activity corrections can also vary depending on the method used We only considered activity corrections for charged species, while neutral species may also have γ ’s that are non unity (e.g., MgSO 4 ) 34
Problems Mentioned Only 1 reaction, 1 set of species, simple equilibrium Didn’t consider any other reactions involving Mg or SO 4 that might influence our results CO 3 2- Ca 2+ Na + Mg 2+ (aq) + SO 4 2- MgSO 4(aq) (aq) F - K + Zn 2+ Other reactions influence amount of MgSO 4 produced 35
Recommend
More recommend