CEE 370 Environmental Engineering Principles Lecture #32 - PDF document

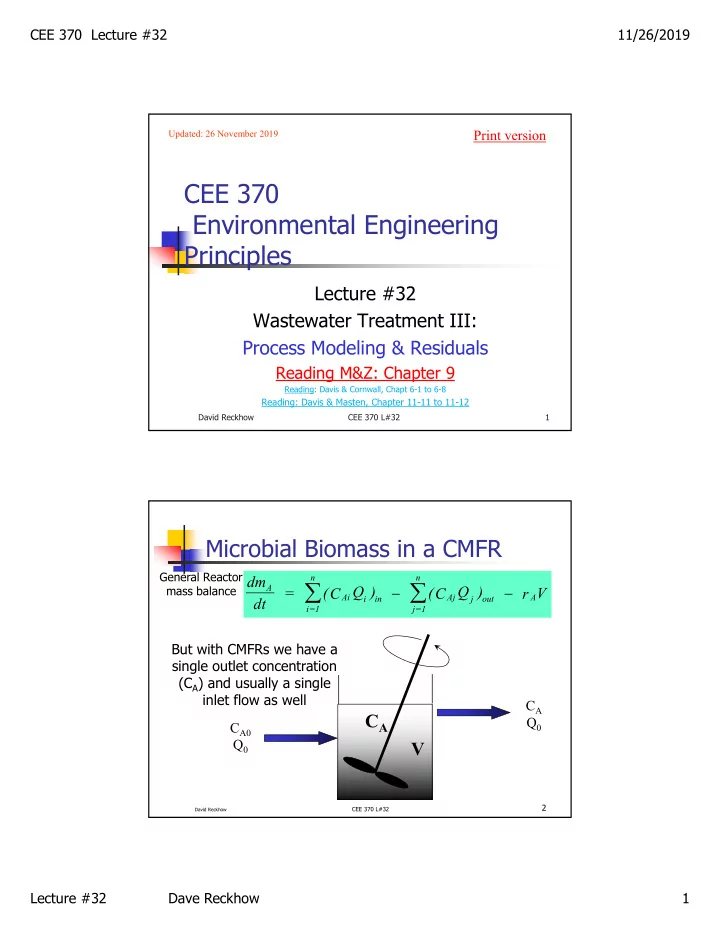
CEE 370 Lecture #32 11/26/2019 Print version Updated: 26 November 2019 CEE 370 Environmental Engineering Principles Lecture #32 Wastewater Treatment III: Process Modeling & Residuals Reading M&Z: Chapter 9 Reading: Davis & Cornwall, Chapt 6-1 to 6-8 Reading: Davis & Masten, Chapter 11-11 to 11-12 David Reckhow CEE 370 L#32 1 Microbial Biomass in a CMFR General Reactor n n dm   A (C Q )  (C Q )  mass balance dt = r V Ai Aj A i in j out i=1 j=1 But with CMFRs we have a single outlet concentration (C A ) and usually a single inlet flow as well C A C A Q 0 C A0 Q 0 V 2 CEE 370 L#32 David Reckhow Lecture #32 Dave Reckhow 1

CEE 370 Lecture #32 11/26/2019 Batch Microbial Growth 0 0 General Reactor n n dM   A  (C Q ) (C Q ) mass balance = - r V Ai Aj A i in j out dt i=1 j=1 Batch reactors are usually filled, allowed to react, then emptied for the next batch Because there isn’t any flow in a batch reactor: 1 C A dM A = - r A V dt For 1 st order V X kX And: biomass growth dC A dt = - r A 3 CEE 370 L#32 David Reckhow Batch Microbial Growth  Observed behavior Stationary Death Covered in lecture #17 Lag Exponential Growth Time 4 CEE 370 L#32 David Reckhow Lecture #32 Dave Reckhow 2

CEE 370 Lecture #32 11/26/2019 Exponential Growth model Covered in lecture #17  dX      X  dt  gr D&M Text where, N X = concentration of microorganisms at time t t t = time  = proportionality constant or specific r growth rate, [time ─ 1 ] dN/dt dX/dt = microbial growth rate, [mass per volume-time] 5 CEE 370 L#32 David Reckhow Exp. Growth (cont.) Covered in lecture #17  dX   dX      X     dt or  dt   X  gr gr   X    ln = t   X o  t X = X e o 6 CEE 370 L#32 David Reckhow Lecture #32 Dave Reckhow 3

CEE 370 Lecture #32 11/26/2019 Substrate-limited Growth  Also known as resource-limited growth  THE MONOD MODEL S   dX SX        and   X max S  max K S   dt  K S gr S where,  max = maximum specific growth rate, [day -1 ] S = concentration of limiting substrate, [mg/L] K s = Monod or half-velocity constant, or half saturation coefficient, [mg/L] 7 CEE 370 L#32 David Reckhow Monod Kinetics Covered in lecture #17 0.5*µ m K S 8 CEE 370 L#32 David Reckhow Lecture #32 Dave Reckhow 4

CEE 370 Lecture #32 11/26/2019 Substrate Utilization & Yield  Related to growth by Y, the yield coefficient  Mass of cells produced H&H, Fig 11-38, pp.406 per mass of substrate utilized dX  X dt   Y  dS S dt  Just pertains to cell growth  dX  dS    Y dt dt   gr 9 CEE 370 L#32 David Reckhow Microbial Growth  dX  dS    Y  dt  dt gr  Monod kinetics in a chemostat (batch reactor) Substitute for dS   dX  SX dS XS      max   X max  S   dt  K S dt Y K S gr S & Divide by Y XS  Where  r k e su  K S  dS/dt = r su = actual substrate utilization rate S e  k = maximum substrate utilization rate = μ max /Y  S = concentration of substrate (S e in H&H)  K S = half-saturation constant  Y = cell yield = dX/dS 10 CEE 370 L#32 David Reckhow Lecture #32 Dave Reckhow 5

CEE 370 Lecture #32 11/26/2019 Death  Bacterial cells also die at a characteristic first order rate with a rate constant, k  dX      k X d  dt  d  This occurs at all times, and is independent of the substrate concentration 11 CEE 370 L#32 David Reckhow Overall model: chemostat  Combining growth and death, we have:  dX   dX   dX           dt   dt   dt  net gr d SX    k X See: M&Z equ 9.3 max d  K S S  And in terms of substrate utilization  dX  dS   Y    dt  dt gr  dX   dS      Y   k X d dt dt     net 12 CEE 370 L#32 David Reckhow Lecture #32 Dave Reckhow 6

CEE 370 Lecture #32 11/26/2019 Activated Sludge Flow Schematic  Conventional X o Q+ Q r Q X X e Effluent S o Aeration S S Basin Settling Influent Tank V,X Q r X r Return activated sludge Q w X r S 13 Waste activated sludge CEE 370 L#32 David Reckhow Efficiency & HRT  Efficiency of BOD removal    S S 100 %  E o S o  Hydraulic Retention Time, HRT (Aeration Time)  Same as retention time in DWT (t R ) V    Actual HRT is a bit different Q  Isn’t used as much in design V   act  Q Q R 14 CEE 370 L#32 David Reckhow Lecture #32 Dave Reckhow 7

CEE 370 Lecture #32 11/26/2019 SRT – solids retention time & R  SRT: Primary operation and design parameter  How long does biomass stay in system XV XV      See: M&Z equ 9.10 c   Q Q X Q X Q X w e w r w r  Typically equals 5-15 days Q  Recycle Ratio  R r Q  Values of 0.25-1.0 are typical 15 CEE 370 L#32 David Reckhow F:M Ratio and volumetric loading  Food-to-Microorganism Ratio (F/M)  F Q BOD   M V X F QS  o M&Z equ 9.16 M XV  Typical values are 0.2-0.6 in complete mixed AS  BOD volumetric Loading QS  Loading o V  Typically 50-120 lb BOD/day/1000ft 3 tank volume 16 CEE 370 L#32 David Reckhow Lecture #32 Dave Reckhow 8

CEE 370 Lecture #32 11/26/2019 Act. Sludge: Biomass Model  dX   dX   dX     Steady State mass balance on biomass        dt   dt   dt  net gr d   dX dX SX  0     V QX Q X Q X V      k X max  d o e e w r K S dt  dt  S batch From chemostat model  Incorporating the chemostat model gets:   dX SX          V 0 QX Q X Q X V k X   o e e w r max d  dt K S   S  And simplifying   SX         QX Q X Q X V k X   o e e w r max  d K S   S  Finally, we recognize that the amount of solids entering with the WW (i.e., X o ) and leaving in the treated effluent (i.e., X e ) is quite small and can be neglected 17 CEE 370 L#32 David Reckhow Biomass Model II  So it becomes   SX      Q X V k X   w r max  d K S   S  And rearranging Q X S 1     w r k  max d  VX K S c S Earlier equation for SRT XV XV      c   Q Q X Q X Q X w e w r w r 18 CEE 370 L#32 David Reckhow Lecture #32 Dave Reckhow 9

CEE 370 Lecture #32 11/26/2019 Act. Sludge: Substrate Model  Steady state mass balance on substrate  dS XS  max S  dt Y K S dS  dS   0     V QS Q S Q S V   o e w From chemostat model dt  dt  batch  Substituting and noting that Q e =Q-Q w    XS       max QS QS Q S Q S V   o w w  Y K S   S  And further simplifying    XS       Q S S V max   o  Y K S   S 19 CEE 370 L#32 David Reckhow Merging the biomass & substrate models If we divide the previous equation by V and X         Q S S S XS        Q S S V max o max   o  Y K S    VX Y K S S S Multiply both sides by Y     YQ S S S   o M&Z equ 9.8 max  VX K S S Now insert the LH term into the 1  Q X S     w r k earlier equation based on biomass  max  d VX K S c S    1 Q X YQ S S    w r o k M&Z equ 9.9  d VX VX c 20 CEE 370 L#32 David Reckhow Lecture #32 Dave Reckhow 10

CEE 370 Lecture #32 11/26/2019 Combined model II  Now recognize that Q/V is the reciprocal of the HRT    1 1 Y S S    o k d  X c 21 CEE 370 L#32 David Reckhow Question  All else being equal, as SRT goes up: Settleability goes down 1. F/M goes down 2. Waste sludge return ratio must go down 3. Endogenous respiration becomes less 4. important Sludge yield increases 5. 22 CEE 370 L#32 David Reckhow Lecture #32 Dave Reckhow 11