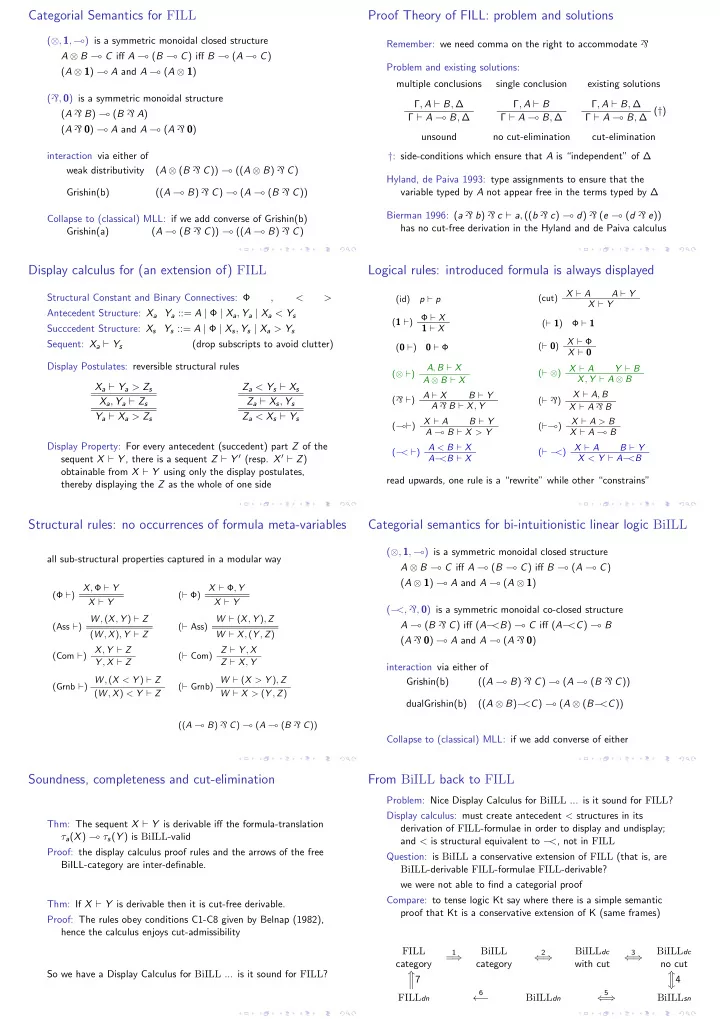
Categorial Semantics for FILL Proof Theory of FILL: problem and - PDF document

Categorial Semantics for FILL Proof Theory of FILL: problem and solutions ( ⊗ , 1 , ⊸ ) is a symmetric monoidal closed structure Remember: we need comma on the right to accommodate ` A ⊗ B ⊸ C iff A ⊸ ( B ⊸ C ) iff B ⊸ ( A ⊸ C ) Problem and existing solutions: ( A ⊗ 1 ) ⊸ A and A ⊸ ( A ⊗ 1 ) multiple conclusions single conclusion existing solutions ( ` , 0 ) is a symmetric monoidal structure Γ , A ⊢ B , ∆ Γ , A ⊢ B Γ , A ⊢ B , ∆ ( † ) ( A ` B ) ⊸ ( B ` A ) Γ ⊢ A ⊸ B , ∆ Γ ⊢ A ⊸ B , ∆ Γ ⊢ A ⊸ B , ∆ ( A ` 0 ) ⊸ A and A ⊸ ( A ` 0 ) unsound no cut-elimination cut-elimination interaction via either of † : side-conditions which ensure that A is “independent” of ∆ weak distributivity ( A ⊗ ( B ` C )) ⊸ (( A ⊗ B ) ` C ) Hyland, de Paiva 1993: type assignments to ensure that the Grishin(b) (( A ⊸ B ) ` C ) ⊸ ( A ⊸ ( B ` C )) variable typed by A not appear free in the terms typed by ∆ Bierman 1996: ( a ` b ) ` c ⊢ a , (( b ` c ) ⊸ d ) ` ( e ⊸ ( d ` e )) Collapse to (classical) MLL: if we add converse of Grishin(b) has no cut-free derivation in the Hyland and de Paiva calculus Grishin(a) ( A ⊸ ( B ` C )) ⊸ (( A ⊸ B ) ` C ) Display calculus for (an extension of) FILL Logical rules: introduced formula is always displayed X ⊢ A A ⊢ Y Structural Constant and Binary Connectives: Φ (id) p ⊢ p (cut) , < > X ⊢ Y Antecedent Structure: X a Y a ::= A | Φ | X a , Y a | X a < Y s Φ ⊢ X ( 1 ⊢ ) ( ⊢ 1 ) Φ ⊢ 1 Succcedent Structure: X s Y s ::= A | Φ | X s , Y s | X a > Y s 1 ⊢ X X ⊢ Φ Sequent: X a ⊢ Y s (drop subscripts to avoid clutter) ( ⊢ 0 ) ( 0 ⊢ ) 0 ⊢ Φ X ⊢ 0 Display Postulates: reversible structural rules A , B ⊢ X X ⊢ A Y ⊢ B ( ⊢ ⊗ ) ( ⊗ ⊢ ) A ⊗ B ⊢ X X , Y ⊢ A ⊗ B X a ⊢ Y a > Z s Z a < Y s ⊢ X s X ⊢ A , B A ⊢ X B ⊢ Y ( ` ⊢ ) ( ⊢ ` ) X a , Y a ⊢ Z s Z a ⊢ X s , Y s A ` B ⊢ X , Y X ⊢ A ` B Y a ⊢ X a > Z s Z a < X s ⊢ Y s X ⊢ A > B X ⊢ A B ⊢ Y ( ⊸ ⊢ ) ( ⊢ ⊸ ) A ⊸ B ⊢ X > Y X ⊢ A ⊸ B Display Property: For every antecedent (succedent) part Z of the A < B ⊢ X X ⊢ A B ⊢ Y ( − < ⊢ ) ( ⊢ − < ) sequent X ⊢ Y , there is a sequent Z ⊢ Y ′ (resp. X ′ ⊢ Z ) A − < B ⊢ X X < Y ⊢ A − < B obtainable from X ⊢ Y using only the display postulates, read upwards, one rule is a “rewrite” while other “constrains” thereby displaying the Z as the whole of one side Structural rules: no occurrences of formula meta-variables Categorial semantics for bi-intuitionistic linear logic BiILL ( ⊗ , 1 , ⊸ ) is a symmetric monoidal closed structure all sub-structural properties captured in a modular way A ⊗ B ⊸ C iff A ⊸ ( B ⊸ C ) iff B ⊸ ( A ⊸ C ) ( A ⊗ 1 ) ⊸ A and A ⊸ ( A ⊗ 1 ) X , Φ ⊢ Y X ⊢ Φ , Y (Φ ⊢ ) ( ⊢ Φ) X ⊢ Y X ⊢ Y ( − <, ` , 0 ) is a symmetric monoidal co-closed structure W , ( X , Y ) ⊢ Z W ⊢ ( X , Y ) , Z A ⊸ ( B ` C ) iff ( A − < B ) ⊸ C iff ( A − < C ) ⊸ B (Ass ⊢ ) ( ⊢ Ass) ( W , X ) , Y ⊢ Z W ⊢ X , ( Y , Z ) ( A ` 0 ) ⊸ A and A ⊸ ( A ` 0 ) X , Y ⊢ Z Z ⊢ Y , X (Com ⊢ ) ( ⊢ Com) Y , X ⊢ Z Z ⊢ X , Y interaction via either of W , ( X < Y ) ⊢ Z W ⊢ ( X > Y ) , Z Grishin(b) (( A ⊸ B ) ` C ) ⊸ ( A ⊸ ( B ` C )) (Grnb ⊢ ) ( W , X ) < Y ⊢ Z ( ⊢ Grnb) W ⊢ X > ( Y , Z ) dualGrishin(b) (( A ⊗ B ) − < C ) ⊸ ( A ⊗ ( B − < C )) (( A ⊸ B ) ` C ) ⊸ ( A ⊸ ( B ` C )) Collapse to (classical) MLL: if we add converse of either Soundness, completeness and cut-elimination From BiILL back to FILL Problem: Nice Display Calculus for BiILL ... is it sound for FILL ? Display calculus: must create antecedent < structures in its Thm: The sequent X ⊢ Y is derivable iff the formula-translation derivation of FILL -formulae in order to display and undisplay; τ a ( X ) ⊸ τ s ( Y ) is BiILL -valid and < is structural equivalent to − < , not in FILL Proof: the display calculus proof rules and the arrows of the free Question: is BiILL a conservative extension of FILL (that is, are BiILL-category are inter-definable. BiILL -derivable FILL -formulae FILL -derivable? we were not able to find a categorial proof Compare: to tense logic Kt say where there is a simple semantic Thm: If X ⊢ Y is derivable then it is cut-free derivable. proof that Kt is a conservative extension of K (same frames) Proof: The rules obey conditions C1-C8 given by Belnap (1982), hence the calculus enjoys cut-admissibility FILL BiILL BiILL dc BiILL dc 1 2 3 = ⇒ ⇐ ⇒ ⇐ ⇒ category category with cut no cut So we have a Display Calculus for BiILL ... is it sound for FILL ? � � � 7 � 4 � � 6 5 FILL dn ← − BiILL dn ⇐ ⇒ BiILL sn