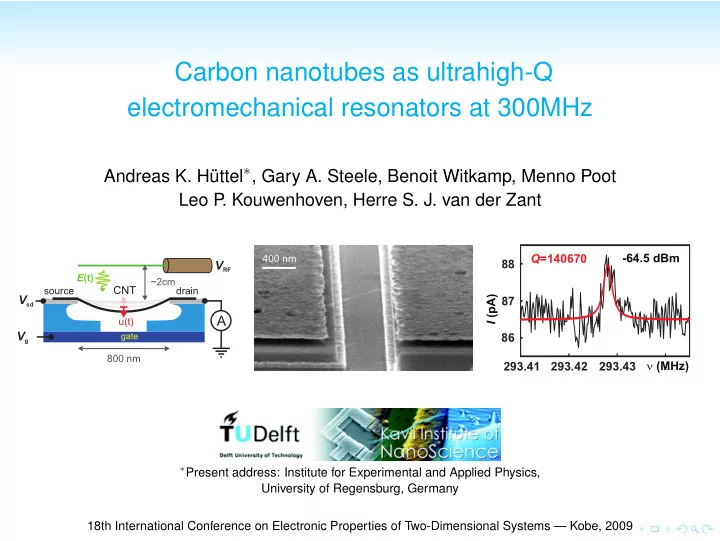
Carbon nanotubes as ultrahigh-Q electromechanical resonators at - PowerPoint PPT Presentation

Carbon nanotubes as ultrahigh-Q electromechanical resonators at 300MHz uttel ∗ , Gary A. Steele, Benoit Witkamp, Menno Poot Andreas K. H¨ Leo P . Kouwenhoven, Herre S. J. van der Zant -64.5 dBm 400 nm Q =140670 88 V RF E (t) ~2cm source CNT drain V sd I (pA) 87 A u(t) V g gate 86 800 nm 293.41 293.42 293.43 � (MHz) ∗ Present address: Institute for Experimental and Applied Physics, University of Regensburg, Germany 18th International Conference on Electronic Properties of Two-Dimensional Systems — Kobe, 2009

Nanotubes as beam resonators — up to now complicated setup — even at 1K, maximally Q ≃ 2000 • Nanotube as nonlinear circuit element • RF downmixing at mech. resonance • Q � 2000 — why? • HF cables to sample: heating, noise • Contamination during lithography • Clamping points? Ultrasensitive Mass Sensing with a Nanotube Electromech. Resonator B. Lassagne, D. Garcia-Sanchez, A. Aguasca and A. Bachtold Nano Lett., 2008, 8 (11), pp 3735–373

Chip fabrication and measurement setup 400 nm V RF E (t) ~2cm source drain CNT V sd A V g gate 800 nm • Nanotube CVD-grown above Pt electrodes, across pre-defined trench • Back gate connected to a gate voltage source V g • RF antenna suspended ∼ 2cm above chip • Dilution refrigerator ( T ≃ 20mK) • Only dc measurement G. A. Steele et al. , Nature Nanotech. 4 , 363 (2009); A. K. H¨ uttel et al. , Nano Lett. 9 , 2547 (2009)

dc Coulomb blockade measurement — beautiful diamonds I (nA) |I| (pA) 2 V sd (mV) 1 10000 0 1000 0.5 100 10 -2 0 1 -4.4 -4.2 V g (V) -4.0 -0.88 -0.86 -0.84 -0.82 V g (V) highly regular quantum dot within the nanotube source dot drain � V g � S N el. � S � D � S � D � D V SD I � Dot V g gate CB SET A. K. H¨ uttel et al. , Nano Lett. 9 , 2547 (2009)

Fixed V g and V SD , sweep of RF signal frequency 2 Q =140670 -17.8 dBm -64.5 dBm 88 I (pA) I (nA) 87 1 86 0 100 300 500 293.41 293.42 293.43 293.44 � (MHz) � (MHz) • Sharp resonant structure in I dc ( ν ) • Very low driving power required • From FWHM, Q ≃ 140000 A. K. H¨ uttel et al. , Nano Lett. 9 , 2547 (2009)

V g dependence — this is really a mechanical resonance! � (MHz) 350 red: continuum beam model 300 � (MHz) 250 300 200 150 d I 250 d � -6 -5 -4 -3 -2 -1 0 V g (V) (pA/MHz) 1000 200 100 150 10 -6 -4 -2 V g (V) 0 larger | V g | − → increased tension − → higher frequency ν A. K. H¨ uttel et al. , Nano Lett. 9 , 2547 (2009)

Detection mechanism — mechanically induced averaging 2 • at resonant driving the nanotube I (nA) � I ac,eff V g position oscillates 1 • oscillating C g 0 − → fast averaging over I ( V g ) 0.1 � I (nA) 0 -0.1 • black line: dc measurement I ( V g ) • red line: this numerically averaged 0.1 � I (nA) 0 • blue: difference, effect of averaging -0.1 • red points: measured peak amplitude in I ( ν ) , for different values of V g -5.22 -5.21 -5.2 -5.19 V g (V) A. K. H¨ uttel et al. , Nano Lett. 9 , 2547 (2009)

Driving into nonlinear response 80 mK, -70 dBm I (pA) • same temperature • same working point V g , V SD -62 dBm I (pA) I (pA) • low driving power: symmetric, “linear” response -56 dBm I (pA) • high driving power: asymmetric response, hysteresis -52.5 dBm Duffing-like oscillator I (pA) � (MHz) A. K. H¨ uttel et al. , Nano Lett. 9 , 2547 (2009)

Temperature dependence of Q (a) -66 dBm, 40mK I (pA) 5 Q=123578 10 -0.36 ~ T Q-factor (b) -50.5 dBm, 320mK I (pA) Q=59283 (c) -45 dBm, 1K I (pA) Q=23210 4 10 0.01 0.1 1 � (MHz) Temperature (K) Q ( T ) fits power law prediction for intrinsic dissipation in nanotube − → H. Jiang et al. , Phys. Rev. Lett. 93 , 185501 (2004) A. K. H¨ uttel et al. , Nano Lett. 9 , 2547 (2009)

Detailed ν ( V g ) : in SET, frequency decreases I (nA) 8 dc current 0 140.0 � (MHz) N holes RF response N-1 holes 139.2 -0.90 -0.84 V g (V) “Coulomb blockade oscillations of mechanical resonance frequency” electrostatic contribution to spring constant

Also Q and nonlinearity dominated by backaction ΔI sd (nA) 0.1 ΔI sd (nA) -0.1 -0.5 1.0 258 -60 dB -45 dB • Dissipation whenever charge can fluctuate � (MHz) • Q decreases on SET peaks 256 • Nonlinearity dominated -4.345 -4.335 -4.345 -4.335 V g (V) V g (V) by tunneling 1 nA Up Down f 0 Fit Q ~ 57000 • Switches between 20000 α < 0 ΔI sd weakening and 2900 α > 0 softening spring 90000 α < 0 -200 200 256 258 - 0 (kHz) � (MHz) � �

Summary, conclusion & outlook! • 120MHz � ν � 360MHz, Q � 150000 • Self-detection of motion via dc current • Easy driving into nonlinear oscillator regime • Q ( T ) is consistent with intrinsic dissipation model • Single-electron steps of the resonance frequency • Backaction of single electron tunneling on ν , Q , nonlinearity • Self-excitation of motion! • Estimated motion amplitude at resonant driving ∼ 250pm compare thermal motion 6 . 5pm, zero-point motion 1 . 9pm u • Application as mass sensor: sensitivity 4 . 2 √ Hz • Without driving: mechanical thermal occupation n ≃ 1 . 2 • Stay tuned for more interesting results!! A. K. H¨ uttel et al. , Nano Lett. 9 , 2547 (2009); G. A. Steele, A. K. H¨ uttel et al.

Self-excitation of the resonator 050 dI/dV ( S) dI/dV ( S) -10 5 A B D I sd 2 5 10 I sd (nA) sd (mV) sd (mV) V -4.93 g 5 V V -10 -2 0 -5.19 -5.17 -1.05 -0.95 V g (V) V g (V) E 284.5 C 10 nA f (MHz) I sd 283.5 0.5 sd (nA) V I V sd g -0.75 -4.935 -4.93 V g (V) Usmani et al. , PRB 75 , 195312 (2007)

Model for ν ( V g ) • Electrostatic force between tube and backgate: F dot = 1 d C g � 2 � V g − V dot (1) 2 d z • Quantum dot voltage: V dot = C g V g + q dot q dot ( q c ) = −| e |� N � ( q c ) , q c = C g V g (2) , C dot • Electrostatic contribution to spring constant: � 2 � � d C g � k dot = V g ( V g − V dot ) 1 −| e | d � N � (3) c dot d z d q c g /C DOT -q DOT = |e| N(q c ) g V DOT C V e/C DOT 1e q c = C g V g q c = C g V g

Model for α ( V g ) Frequency α < 0 α > 0 α < 0 Gate Voltage � 2 d 2 k dot α dot = − d 3 F d z 3 = d 2 � d C g d z 2 k dot ( q c ) = V 2 (4) g d q 2 d z c The sign of α dot follows the sign of the curvature of k dot .