Bounds on Deviation by Markov: Chebyshev Bound E[(R -) 2 ] x 2 - PowerPoint PPT Presentation

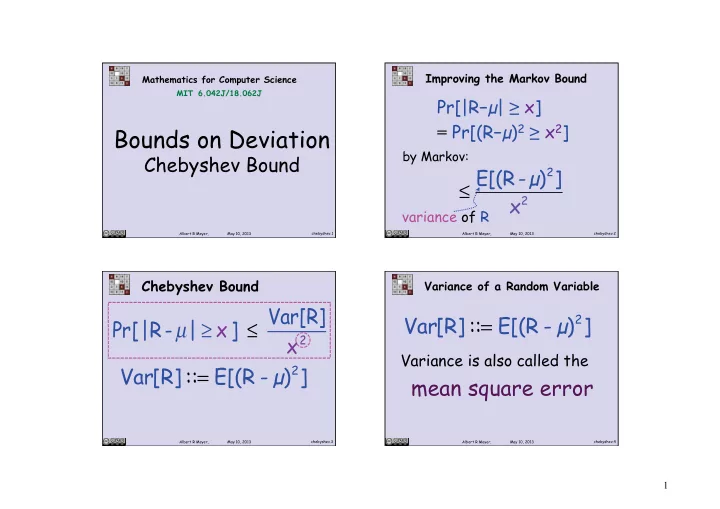
Improving the Markov Bound Mathematics for Computer Science MIT 6.042J/18.062J Pr[|R−µ| ≥ x] = Pr[(R−µ) 2 ≥ x 2 ] Bounds on Deviation by Markov: Chebyshev Bound ≤ E[(R -µ) 2 ] x 2 variance of R chebyshev.1 chebyshev.2 Albert R Meyer, May 10, 2013 Albert R Meyer, May 10, 2013 Chebyshev Bound Variance of a Random Variable Pr[|R - μ | ≥ x ] ≤ Var[R] Var[R] :: = E[(R - µ) 2 ] 2 x Variance is also called the Var[R] :: = E[(R - µ) 2 ] mean square error Albert R Meyer, May 10, 2013 chebyshev.3 Albert R Meyer, May 10, 2013 chebyshev.4 1

Chebyshev Bound Standard Deviation of an RV Standard deviation is also Pr[|R - μ | ≥ x ] ≤ Var[R] called the 2 x root mean square error σ R ::= Var[R] σ R ::= Var[R] standard deviation standard deviation chebyshev.5 chebyshev.6 Albert R Meyer, May 10, 2013 Albert R Meyer, May 10, 2013 Chebyshev Bound Standard Deviation of an RV 2 Pr[|R - μ | ≥ x ] ≤ σ R σ R PDF R 2 x � μ� σ R ::= Var[R] σ R ::= Var[R] Albert R Meyer, May 10, 2013 chebyshev.7 Albert R Meyer, May 10, 2013 chebyshev.8 2

Chebyshev Bound Chebyshev Bound (Restated) 2 Pr[|R - μ | ≥ c σ R ] ≤ 1 Pr[|R - μ | ≥ x ] ≤ σ R 2 2 x c σ R ::= Var[R] σ R ::= Var[R] chebyshev.9 chebyshev.10 Albert R Meyer, May 10, 2013 Albert R Meyer, May 10, 2013 Standard Deviation Pr[|R - μ | ≥ c σ R ] ≤ 1 c 2 R probably not many σ’s from μ: � Pr ≤ 1 further than σ Pr ≤ 1/4 2σ Pr ≤ 1/9 3σ Pr ≤ 1/16 4σ Albert R Meyer, May 10, 2013 chebyshev.11 3

MIT OpenCourseWare http://ocw.mit.edu 6.042J / 18.062J Mathematics for Computer Science Spring 20 15 For information about citing these materials or our Terms of Use, visit: http://ocw.mit.edu/terms.