b v
play

B v B-fields are created by moving charges (currents). B-fields - PDF document

Feb 16, 2023 •305 likes •545 views

Magnetic field and force So far we have studied two forces: gravity and electricity Magnetism is a new force, but also related to electric charges. Gravity is created by mass and gravity acts on masses. kQ = E r Electric fields are

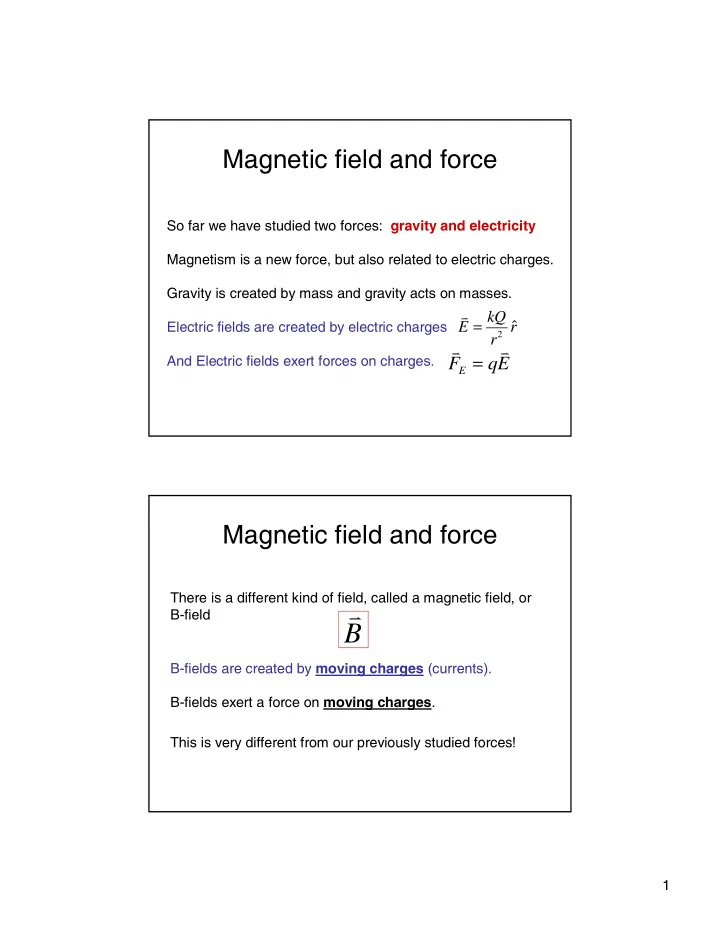
  1. Magnetic field and force So far we have studied two forces: gravity and electricity Magnetism is a new force, but also related to electric charges. Gravity is created by mass and gravity acts on masses. kQ = ˆ E r Electric fields are created by electric charges v 2 r v = And Electric fields exert forces on charges. F E q E v Magnetic field and force There is a different kind of field, called a magnetic field, or B-field B v B-fields are created by moving charges (currents). B-fields exert a force on moving charges . This is very different from our previously studied forces! 1

  2. Natural magnetism Natural magnetism 2

  3. What is the origin of this force? Magnetic charge? Monopoles? Scientists have postulated that there might be some other kind of object that has a “magnetic charge” = Magnetic Monopole. No magnetic monopoles have ever been found. Thus, we do not consider them in classes. 3

  4. Magnetism and electric charge Oersted’s experiment: A compass is placed directly over a wire (here viewed from above). A passing current deflects the needle. Force on a moving charge We will see later exactly how B-fields are made by moving charges. Now we will study how B-fields exert forces on moving charges. = × F v q v B v v The magnetic force exerted on a charge q moving with velocity v in a magnetic field B. This equation actually defines the magnetic B-field. 4

  5. Visualizing a M. Field: Field lines http://www.youtube.com/watch?v=wuA-dkKvrd0 Force on a moving charge = × F q v B v v v Vector cross product. = θ | F | q | v || B | sin v v v B What about the resulting force direction from the cross product? θ v 5

  6. Vector cross product – Right Hand Rule v × u v v • Fingers in direction of first vector. • Bend them into direction of second vector. •Thumb points in cross product direction. •If your hand does not bend that way, flip it around! – never use the left hand ;-) New vector direction is always perpendicular to the original vectors! Force on a moving charge 6

  7. Consequences: = × F q v B v v v = θ | F | q | v || B | sin v v v • If particle is not moving (v=0) then no force. • If velocity and B-field are parallel, then no force. • If velocity and B-field are perpendicular, then maximum force. • If q is negative, then force is in opposite direction. Drawing conventions Drawing vector directions on a 2 dimensional piece of paper? Magnetic Field Vector to the right. Magnetic Field Vector out of the page. Arrow head pointing at you. X Magnetic Field Vector into the page. Arrow tail pointing at you. 7

  8. Clicker Question A negative particle and a positive particle are moving with certain velocities in a constant, uniform magnetic field, as shown. The direction of the B-field is to the right. The (+) particle is moving directly left; the (–) particle is moving directly up. The force on the positive particle due B to the B-field is (in = into page, out = out of page). A: in B: out C: zero D: right E: left Answer: The (+) particle is moving anti-parallel to the B-field. The angle θ is 180 and the force is FB=qvB sin θ = 0. Clicker Question A negative particle and a positive particle are moving with certain velocities in a constant, uniform magnetic field, as shown. The direction of the B-field is to the right. The (+) particle is moving directly left; the (–) particle is moving directly up. The force on the negative particle B due to the B-field is A: in B: out C: zero D: right E: left Answer: The (–) particle is moving at right angles to the field. By the right-hand rule, the direction "v cross B" is into the page, but the particle has a negative charge q, so the force is out of the page. 8

  9. Clicker Question A positive particle is released from rest in a region of space where there is constant, uniform, electric field and a constant, uniform magnetic field. The C A B E electric field points up and the . magnetic field points out of the B (out) page in the diagram below. Which path will the positive particle follow? (All paths shown are in plane of the page.) D: it will not move Answer: The (+) particle will feel a force F E = qE due to the E- field along the direction of the E-field. As it starts moving along the E-field direction, it will acquire a velocity, and it will start to feel a force F B =qvB, due to the B-field. The direction of the force is to the right, by the right-hand-rule. Clicker Question A negative particle and a positive particle are moving with certain velocities in a constant, uniform magnetic field, as shown. The direction of the B-field is to the right. The (+) particle is moving directly left; the (–) particle is moving directly up. The force on the negative particle B due to the B-field is A: in B: out C: zero D: right E: left Answer: The (–) particle is moving at right angles to the field. By the right-hand rule, the direction "v cross B" is into the page, but the particle has a negative charge q, so the force is out of the page. 9

  10. Magnetic field units = × F q v B v v Units for Magnetic Field v B-field = [B] = [Newtons] / [Coulomb x meters/second] = [ Tesla ] How big is a 1 Tesla Magnetic Field? 10 -10 Tesla Interstellar Space 10 -10 Tesla Human Being 5x10 -5 Tesla Earth’s Surface 10 -2 Tesla Sun’s Surface 10 -2 Tesla Small Bar Magnet Experiment Magnet 1 Tesla Maximum Steady Magnet 30 Tesla Maximum in Explosive Magnet 1000 Tesla 10 8 Tesla Surface of Neutron Star 10

  11. Another Unit System 1 Gauss = 10 -4 Tesla Thus, the Earth’s magnetic field is ~ 0.5 Gauss. This unit system is often used when talking about small magnetic fields, but it is not the SI unit system ! Earth’s magnetic field 11

  12. Clicker Question Here is an event display from a high energy experiment. There is a 1 Tesla uniform magnetic field coming out of the page. What is the sign of the electric charge? = × A)Positive F q v B v v v B)Negative Videos Feymann's "why" and "Fields" 12

  13. Motion of charged particles in a M. Field = × F q v B Because the force is always v v v perpendicular to the velocity (direction of motion), the Magnetic force can do no work on q. ⊥ ∆ = ⋅ ∆ = F B v r W F r 0 v since v v B-field cannot change the Kinetic Energy of a moving particle, but can change its direction of motion. = ∆ = W net KE 0 Charged particle in a perpendicular field Particle moving in a plane with a B- v field uniformly out of the plane. B Results in circular motion! v No change in KE, but constant F change in velocity direction. B F 13

  14. Charged particle in a perpendicular field = = × | F v | m | a | | q v B v | v v = | F | q | v || B | v v Since velocity and B are always v perpendicular. 2 mv = = | F | q | v || B | v v Since circular motion. v R v mv Radius of circular motion R R = depends on m, v, q, B. qB B Charged particle in a perpendicular field One can then solve for the frequency of v revolution (“cyclotron frequency”). R = f # revolution s/second B distance = π qRB 2 R = = v m time T Period 1 = qB = The cyclotron frequency is f π T 2 m independent of R. 14

  15. Helical motion v What if in addition to the mv R R = circular motion in this plane, qB there is a non-zero velocity out of the page? B This extra velocity contributes no additional force since it is parallel to B. Aurora borealis (“Northern lights”) 15

  16. Clicker Question A (+) charged particle with an initial speed v o is moving in a plane perpendicular to a uniform magnetic field (B into the page). There is a tenuous gas throughout the region which causes viscous drag and slows the particle over time. The path of the particle is B A: a spiral inward B: a spiral outward C: something else Applications: Mass spectrometer 16

  17. Applications: velocity selector F E = qE Electric force: F B = qvB Magnetic force: = − F qvB qE Total force: ∑ For the particle to pass and not be deflected: E − = → = qvB qE 0 v B Thomson’s e/m experiment 1 2 eV 2 = → = From energy conservation: mv eV v 2 m E v = We have seen that to pass B through we need: e = 2 E Combining the two we obtain: 2 m 2 VB 17

  18. Force on current carrying wires Since B-fields exert forces on moving = × F q v B v v v charges, it is natural that B-fields exert forces on current carrying wires. How do we quantify this force in terms of current I , instead of q and v ? Force on current carrying wires Consider the wire shown below. = × F v ( one charge) q v B v v Now add a Magnetic Field. # charges in this Force segment of wire B X N = n x A x L Velocity v + Area A Volume #/Volume = × F v ( total ) ( nALq ) v B v Length vector L v 18

  19. Force on current carrying wires = × F v ( total ) ( nALq ) v B v v How to relate this to current? I = = I = nAqv J nqv d d A = × * Notice that L points along v, which F ( total ) I L B v v v is the direction of the current. Clicker Question A current-carrying wire is in a B-field. The wire is oriented to the B-field as shown. What is the direction of the magnetic force on the wire? A) Right B B) Down C) Out of the Page i D) Into the Page E) None of these. 19

Recommend

More recommend