= B q ( ) p x dx ( ) . 0 = For a monopolist the private - PDF document

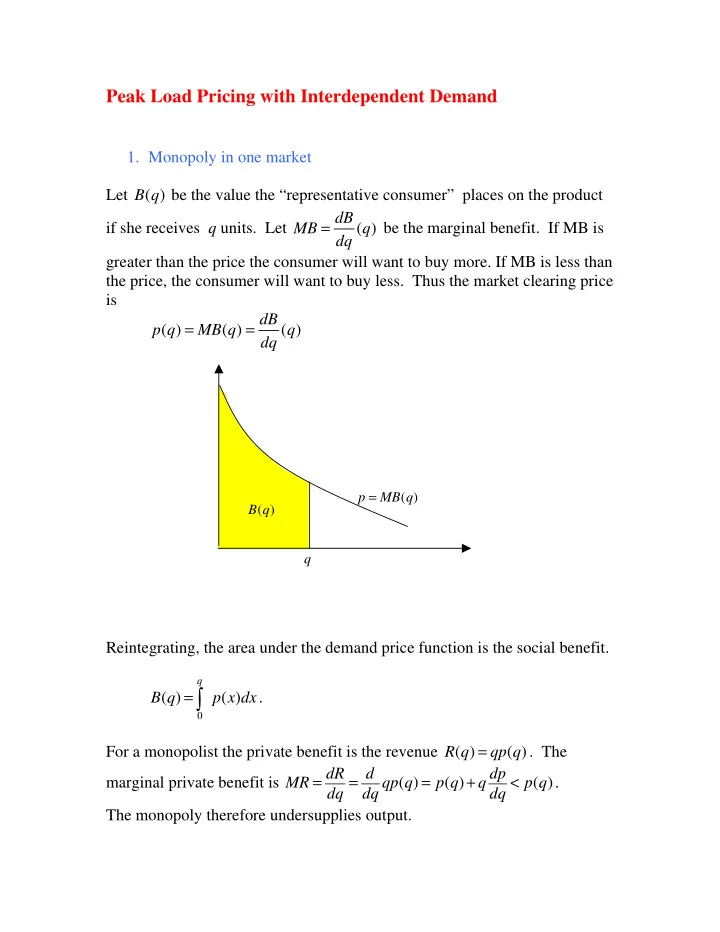
Peak Load Pricing with Interdependent Demand 1. Monopoly in one market Let ( ) B q be the value the “representative consumer” places on the product dB = if she receives q units. Let MB ( ) q be the marginal benefit. If MB is dq greater than the price the consumer will want to buy more. If MB is less than the price, the consumer will want to buy less. Thus the market clearing price is dB = = p q ( ) MB q ( ) ( ) q dq = p MB q ( ) B q ( ) q Reintegrating, the area under the demand price function is the social benefit. q = ∫ B q ( ) p x dx ( ) . 0 = For a monopolist the private benefit is the revenue R q ( ) qp q ( ) . The dR d dp = = = + < marginal private benefit is MR qp q ( ) p q ( ) q p q ( ) . dq dq dq The monopoly therefore undersupplies output.

Quadratic benefit function = − B q ( ) q a ( 1 bq ) . 2 For such a benefit function the demand price function is the familiar linear demand price function. Demand price function dB = = − − = − 1 1 p q ( ) ( a bq ) bq a bq 2 2 dq Marginal revenue dR d = = − = − MR q a ( bq ) a 2 bq dq dq = M M M The monopolist chooses output q such that MR q ( ) MC q ( ) . − * Social Surplus = B q ( ) C q ( ) . Thus social surplus is maximized at q such that dB dC − = − = − = * * . MB MC p q ( ) MC q ( ) 0 dq dq 2. Multiple products and separable quadratic benefit function = + = − + − 1 1 B q q ( , ) B q ( ) B q ( ) q a ( b q ) q a ( b q ) 1 2 1 1 2 2 1 1 2 1 1 2 2 2 2 2 ∂ B = = = − p q ( ) MB a bq . ∂ i i i i i q 1

3. Multiple products and symmetric quadratic benefit function = − ⋅ + − ⋅ 1 1 B q ( ) q a ( b q ) q a ( b q ) 1 1 2 1 2 2 2 2 = b ( b , b ) = where 1 11 12 and 12 b b = 21 b ( b , b ) 2 21 22 and ⋅ = b q sumproduct( , ) b q i i Multiplying out terms, = − − + − − 1 1 1 1 B q a ( b q b q ) q a ( b q b q ) 1 1 2 11 1 2 12 2 2 2 2 12 1 2 22 2 We can now differentiate to obtain the demand price functions. ∂ B = = − − − 1 1 p q ( ) a b q b q b q . ∂ 1 1 11 1 12 2 21 2 2 2 q 1 Given symmetry, this can be rewritten as follows. = − − = − ⋅ . p q ( ) a b q b q a b q 1 1 11 1 12 2 1 1 Similarly = − ⋅ . p q ( ) a b q 2 2 2 Finally we can compute the marginal revenue of a monopolist. = − ⋅ + − ⋅ R q ( ) q a ( b q q a ( b q ) 1 1 1 2 2 2 Multiplying out terms, = − − + − − R q a ( b q b q ) q a ( b q b q ) 1 1 11 1 12 2 2 2 12 1 22 2 Hence, ∂ R = = − − − MR a 2 b q b q b q . ∂ 1 1 11 1 12 2 21 2 q 1

Given symmetry, this can be rewritten as follows. = − − = − ⋅ . p q ( ) a 2 b q 2 b q a 2 b q 1 1 11 1 12 2 1 1 Thus there is a close parallel with the separable case. 4. Peak Load pricing Example = − − p 200 4 q q 1 1 2 = − − p 300 1 q 3 q 2 1 2 marginal operating cost = 20 marginal capacity cost = 30 qi ai bij b.q p(qi) MR(qi) R(qi) Bi c capacity 37.273 30 period 1 13.182 200 4 1 90 110 20 1450 2043.18 20 period 2 37.273 300 1 3 125 175 50 6522.73 8852.27 20 q 13.18 37.27 Revenue Benefit Cost 7972.73 10895.52127.27 pi=ai-SUMPRODUCT(bi,q) MRi=ai-2*SUMPRODUCT(bi,q) Profit Surplus Bi=qi*(ai-0.5*SUMPRODUCT(bi,q)) 5845.45 8768.18 Exercise: For this example, solve for the outputs and prices that maximize social surplus.