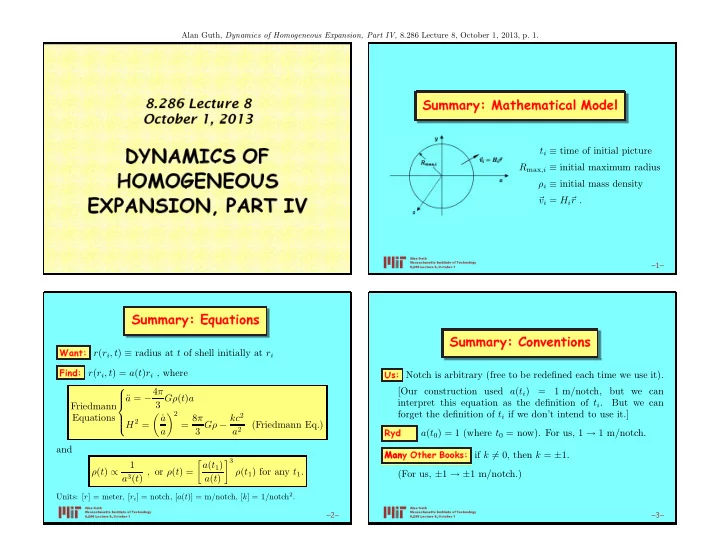
Alan Guth, Dynamics of Homogeneous Expansion, Part IV, 8.286 Lecture - PowerPoint PPT Presentation

� Alan Guth, Dynamics of Homogeneous Expansion, Part IV, 8.286 Lecture 8, October 1, 2013, p. 1. 8.286 Le ture 8 Summary: Mathemati al Model O tober 1, 2013 t i ≡ time of initial picture DYNAMICS OF R max ,i ≡ initial maximum radius HOMOGENEOUS ρ i ≡ initial mass density � v i = H i � r . EXPANSION, PART IV Alan Guth Massa husetts Institute of T e hnology –1– 8.286 Le ture 8, O tober 1 Summary: Equations Summary: Conventions Want: r ( r i , t ) ≡ radius at t of shell initially at r i Find: r ( r i , t ) = a ( t ) r i , where Us: Notch is arbitrary (free to be redefined each time we use it).  4 π [ Our construction used a ( t i ) = 1 m/notch, but we can ¨ a = − Gρ ( t ) a   i nterpret this equation as the definition of t i . But we can  3 Friedmann � ˙ f orget the definition of t i if we don’t intend to use it.] � 2 Equations 8 π kc 2 a H 2 =  = Gρ − (Friedmann Eq.)   3 a 2 a Ryd en: a ( t 0 ) = 1 (where t 0 = now). For us, 1 → 1 m/notch. and Books: if k = 0, then k = ± 1. Many Man y Other Other Books: � � 3 1 a ( t ) 1 ρ ( t ) ∝ , or ρ ( t ) = ρ ( t 1 ) for any t 1 . ( For us, ± 1 → ± 1 m/notch.) a 3 ( t ) a ( t ) Units: [ r ] = meter, [ r i ] = notch, [ a ( t )] = m/notch, [ k ] = 1/notch 2 . Alan Guth Alan Guth Massa husetts Institute of T e hnology Massa husetts Institute of T e hnology –2– –3– 8.286 Le ture 8, O tober 1 8.286 Le ture 8, O tober 1

Alan Guth, Dynamics of Homogeneous Expansion, Part IV, 8.286 Lecture 8, October 1, 2013, p. 2. Summary: Types of Solutions 3) k = 0 ( E = 0): critical mass de nsity. kc 2 3 H 2 8 πG 2 H = = ρ ≡ ρ c = 8 πG . ρ − ⇒ 1 ) a 3 ( t 1 ) 8 πG ρ ( t − kc 2 . a 2 3 a 2 ˙ = 3 a ( t ) ���� =0 For intuition, remember that k ∝ − E , where E is a measure of the energy of Flat Universe. the system. Summary: ρ > ρ c ⇐ ⇒ closed, ρ < ρ c ⇐ ⇒ open, ρ = ρ c ⇐ ⇒ flat. Types of Solutions: Numerical value: For H = 67 . 3 km-s − 1 -Mpc − 1 (Planck 2013 plus a 2 > ( − kc 2 ) > 0, so the universe expands 1) k < 0 ( E > 0): unbound system. ˙ forever. Open Universe. other experiments), a 2 2) k > 0 ( E < 0): bound system. ˙ ≥ 0 = ⇒ ρ c = 8 . 4 × 10 − 30 g/cm 3 ≈ 5 proton masses per m 3 . 1 ) a 3 ( t 1 ) . 8 πG ρ ( t a max = 3 kc 2 ρ Definition: Ω ≡ . ρ c Universe reaches maximum size and then contracts to a Big Crunch. Closed Universe. Alan Guth Massa husetts Institute of T e hnology –4– –5– 8.286 Le ture 8, O tober 1 Summary: Evolution of a Flat Universe If k = 0, then � ˙ a � 2 8 πG const const da = ρ = = ⇒ = a 3 a 3 dt a 1 / 2 2 a 3 / 2 = (const) t + c ′ . a 1 / 2 a da = const dt = ⇒ = ⇒ 3 Choose the zero of time to make c ′ = 0, and then t 2 / 3 . a ( t ) ∝ Alan Guth Massa husetts Institute of T e hnology –6– 8.286 Le ture 8, O tober 1

MIT OpenCourseWare http://ocw.mit.edu 8.286 The Early Universe Fall 2013 For information about citing these materials or our Terms of Use, visit: http://ocw.mit.edu/terms.