Addition Identity For Sine MHF4U: Advanced Functions Consider the - PDF document

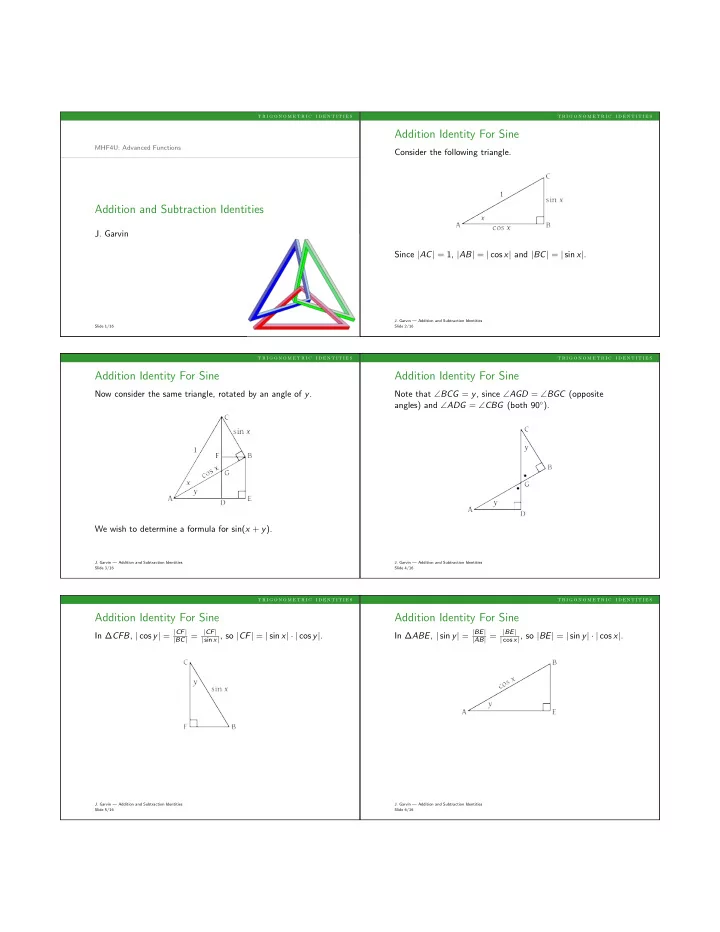
t r i g o n o m e t r i c i d e n t i t i e s t r i g o n o m e t r i c i d e n t i t i e s Addition Identity For Sine MHF4U: Advanced Functions Consider the following triangle. Addition and Subtraction Identities J. Garvin Since | AC | = 1, | AB | = | cos x | and | BC | = | sin x | . J. Garvin — Addition and Subtraction Identities Slide 1/16 Slide 2/16 t r i g o n o m e t r i c i d e n t i t i e s t r i g o n o m e t r i c i d e n t i t i e s Addition Identity For Sine Addition Identity For Sine Now consider the same triangle, rotated by an angle of y . Note that ∠ BCG = y , since ∠ AGD = ∠ BGC (opposite angles) and ∠ ADG = ∠ CBG (both 90 ◦ ). We wish to determine a formula for sin( x + y ). J. Garvin — Addition and Subtraction Identities J. Garvin — Addition and Subtraction Identities Slide 3/16 Slide 4/16 t r i g o n o m e t r i c i d e n t i t i e s t r i g o n o m e t r i c i d e n t i t i e s Addition Identity For Sine Addition Identity For Sine In ∆ CFB , | cos y | = | CF | | CF | In ∆ ABE , | sin y | = | BE | | BE | | BC | = | sin x | , so | CF | = | sin x | · | cos y | . | AB | = | cos x | , so | BE | = | sin y | · | cos x | . J. Garvin — Addition and Subtraction Identities J. Garvin — Addition and Subtraction Identities Slide 5/16 Slide 6/16

t r i g o n o m e t r i c i d e n t i t i e s t r i g o n o m e t r i c i d e n t i t i e s Addition Identity For Sine Addition Identity For Sine In ∆ ACD , | AC | = 1, so | sin( x + y ) | = | CD | . Putting things together, | sin( x + y ) | = | sin x | · | cos y | + | sin y | · | cos x | . This gives us the desired formula for sin( x + y ). Addition Identity For Sine For any angles x and y , sin( x + y ) = sin x cos y + sin y cos x . | CD | = | CF + FD | = | CF + BE | , so | sin( x + y ) | = | CF + BE | . J. Garvin — Addition and Subtraction Identities J. Garvin — Addition and Subtraction Identities Slide 7/16 Slide 8/16 t r i g o n o m e t r i c i d e n t i t i e s t r i g o n o m e t r i c i d e n t i t i e s Subtraction Identity For Sine Subtraction Identity For Sine We can use the previous expression to derive an identity for For sin( x − y ), substitute − y for y . sin( x − y ). sin( x + ( − y )) = sin x cos( − y ) + sin( − y ) cos x First, recall that sin( − x ) = − sin x , and cos( − x ) = cos x . sin( x − y ) = sin x cos y − sin y cos x Subtraction Identity For Sine For any angles x and y , sin( x − y ) = sin x cos y − sin y cos x . J. Garvin — Addition and Subtraction Identities J. Garvin — Addition and Subtraction Identities Slide 9/16 Slide 10/16 t r i g o n o m e t r i c i d e n t i t i e s t r i g o n o m e t r i c i d e n t i t i e s Verifying the Sine Identities Addition Identity For Cosine Example To develop an addition formula for cosine, we can make use � 5 π of the cofunction identities developed earlier. 6 − 2 π = sin 5 π 6 cos 2 π 3 − sin 2 π 3 cos 5 π � Verify that sin 6 . 3 � π � Since cos( a ) = sin 2 − a , let a = x + y . � π � cos( x + y ) = sin 2 − [ x + y ] � 5 π � 5 π �� π 6 − 2 π 6 − 4 π � � � � sin = sin = sin 2 − x − y 3 6 = sin π Use the subtraction formula for sine next. 6 = 1 � π � π 2 � � cos( x + y ) = sin 2 − x cos y − sin y cos 2 − x = cos x cos y − sin y sin x √ √ � � sin 5 π 6 cos 2 π 3 − sin 2 π 3 cos 5 π 6 = 1 − 1 3 3 � � − − 2 2 2 2 = − 1 4 + 3 4 Addition Identity For Cosine = 1 2 For any angles x and y , cos( x + y ) = cos x cos y − sin x sin y . J. Garvin — Addition and Subtraction Identities J. Garvin — Addition and Subtraction Identities Slide 11/16 Slide 12/16

t r i g o n o m e t r i c i d e n t i t i e s t r i g o n o m e t r i c i d e n t i t i e s Subtraction Identity For Cosine Verifying the Cosine Identities A similar formula can be developed for subtraction using Example cosine. � π � Verify that cos 3 + π = cos π 3 cos π − sin π 3 sin π . cos( x − y ) = cos( x + ( − y )) = cos x cos( − y ) − sin x sin( − y ) � π � π 3 + 3 π = cos x cos y + sin x sin y cos 3 + π � = cos � 3 = cos 4 π 3 = − 1 2 Subtraction Identity For Cosine For any angles x and y , cos( x − y ) = cos x cos y + sin x sin y . √ 3 sin π = 1 3 cos π 3 cos π − sin π 2 ( − 1) − 2 (0) = − 1 2 J. Garvin — Addition and Subtraction Identities J. Garvin — Addition and Subtraction Identities Slide 13/16 Slide 14/16 t r i g o n o m e t r i c i d e n t i t i e s t r i g o n o m e t r i c i d e n t i t i e s Exact Value of a Trigonometric Ratio Questions? Example Determine an exact value for cos π 12 . Begin by expressing 12 as the sum or difference of two angles π for which we know their exact values. 12 = 4 π 12 − 3 π π 12 = π 3 − π 4 Now, use the subtraction formula for cosine. � π cos π 12 = cos 3 − π � 4 = cos π 3 cos π 4 + sin π 3 sin π 4 √ √ √ = 1 2 3 2 2 · 2 + 2 · 2 √ √ 2+ 6 = 4 J. Garvin — Addition and Subtraction Identities J. Garvin — Addition and Subtraction Identities Slide 15/16 Slide 16/16