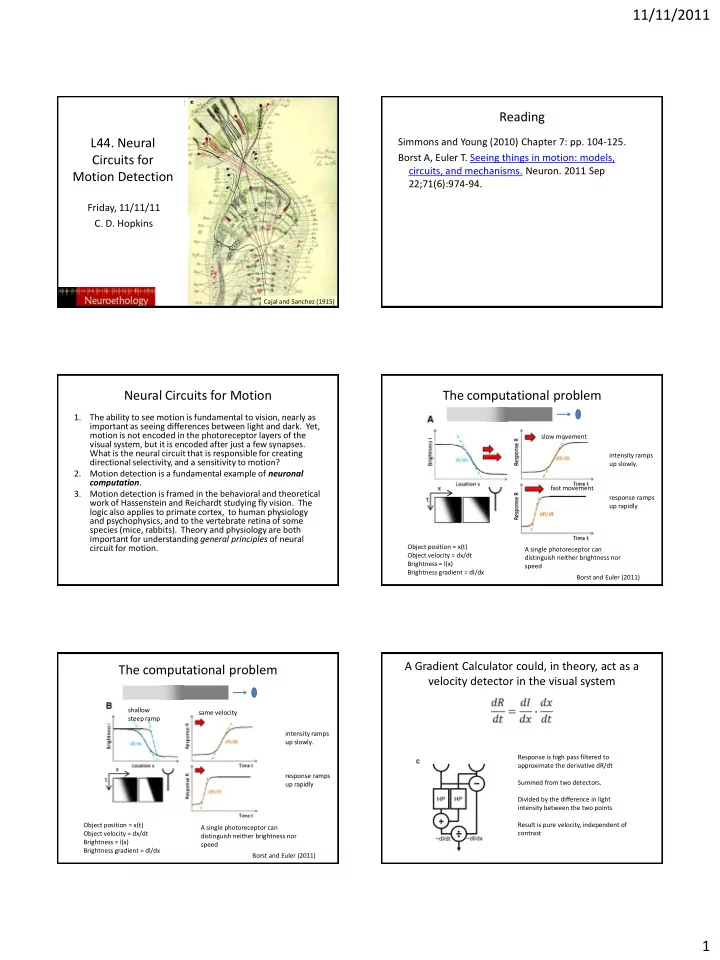
11/11/2011 Reading L44. Neural Simmons and Young (2010) Chapter 7: - PDF document

11/11/2011 Reading L44. Neural Simmons and Young (2010) Chapter 7: pp. 104-125. Borst A, Euler T. Seeing things in motion: models, Circuits for circuits, and mechanisms. Neuron. 2011 Sep Motion Detection 22;71(6):974-94. Friday, 11/11/11 C. D. Hopkins Cajal and Sanchez (1915) Neural Circuits for Motion The computational problem 1. The ability to see motion is fundamental to vision, nearly as important as seeing differences between light and dark. Yet, motion is not encoded in the photoreceptor layers of the slow movement visual system, but it is encoded after just a few synapses. What is the neural circuit that is responsible for creating intensity ramps directional selectivity, and a sensitivity to motion? up slowly. 2. Motion detection is a fundamental example of neuronal computation . moving bar.swf fast movement 3. Motion detection is framed in the behavioral and theoretical response ramps work of Hassenstein and Reichardt studying fly vision. The up rapidly logic also applies to primate cortex, to human physiology and psychophysics, and to the vertebrate retina of some species (mice, rabbits). Theory and physiology are both important for understanding general principles of neural circuit for motion. Object position = x(t) A single photoreceptor can Object velocity = dx/dt distinguish neither brightness nor Brightness = l(x) speed Brightness gradient = dl/dx Borst and Euler (2011) A Gradient Calculator could, in theory, act as a The computational problem velocity detector in the visual system shallow same velocity steep ramp intensity ramps up slowly. Response is high pass filtered to moving bar.swf approximate the derivative dR/dt response ramps Summed from two detectors. up rapidly Divided by the difference in light intensity between the two points Object position = x(t) Result is pure velocity, independent of A single photoreceptor can Object velocity = dx/dt contrast distinguish neither brightness nor Brightness = l(x) speed Brightness gradient = dl/dx Borst and Euler (2011) 1

11/11/2011 Gradient Calculator Correlation Calculator • REICHARDT DETECTOR • Gradient calculators Correlation Calculator are used in machine vision. • Computes the • Relatively simple. correlation between • Insensitive to the two detectors after pattern of stimulation. one has been low pass • sensitive to noise. filtered with a time constant Barlow Levick Detector The Reichardt (Correlation) Motion Detector 2 1 Photoreceptors Low-pass filters F F 2 create delay 1 A A 1 • Barlow and Levick 2 Multiplier (1965) delay • Identical to Reichardt Subtractor but with one half of basic Reichardt model • And not (veto) from R(t) A (T)F (T - t) - A (T)F (T - t) inhibition 2 1 1 2 Predicted responses of Reichardt versus All motion detector models share common features. Models differ in response characteristics Gradient Detectors SIMILARITIES 1. All have spatially separated inputs that record brightness 2. All have asymmetry in temporal filtering – delay line in one channel – time derivative in one channel – low pass filter in one channel 3. Non linear computation (division, multiplication, veto) DIFFERENCES Reichardt: sensitive to image contrast; output is maximum at one particular image velocity (which depends on spatial pattern wavelength) Reichardt detector shows preferred Gradient detector is Gradient: insensitive to image contrast velocity linear Borst, Al Phil. Trans. R. Soc. B 2007 362, 369-374 2

11/11/2011 Theory: Gradient detector is susceptible to noise, while Reichardt detector is insensitive to noise. Velocity Response of the Fly H1 Neuron Signal to Noise Ratios for Reichardt vs. Gradient Detectors Stimulus: sine wave grating moving with noisy velocity distribution in low luminance • Experiments show H1 neurons have peak response at a fixed temporal frequency (directly related to velocity), as in the Reichardt detector models Borst, Al Phil. Trans. R. Soc. B 2007 362, 369-374 DS CELLS Directionally Selective: respond more to motion in one direction than in the opposite direction. DS in Flies • lobula plate Lobula plate: third layer of neurons in the optic pathway (3 rd neuropil) • (cells in the medulla are too small to study) HS: • Lobula Plate Tangential cells (LPTC) horizontal respond to time ordered signals. • Number about 50. Varies by sensitive in red species. HS horizontal sensitive VS vertical sensitive • HS: front to back motion sensitive. Tangential Cell Borst and Euler (2011) Response of HS cell to moving grating DS response from HS cell to moving grating Response to moving grating as a function of the contrast. Note response to black moving bar above that the response increases with response to white moving bar below contrast PD = preferred direction 3

11/11/2011 Tuned to velocity The HS cell is responsive to moving gratings with a preferred velocity. The preferred velocity is dependent upon the spatial frequency of the pattern. When the temporal frequency is plotted, the two patterns are identical. Temporal William Levick and Horace Barlow (photo John Lisman) Levick: Australian National University (Psychology) – student with Barlow frequency is the grating velocity divided by spatial wavelength. Barlow: Univ. Cambridge (student of Adrian and Rushton, post doc Kuffler) DS cell in rabbit retina Barlow and Levick – two models for DS Barlow and Levick, 1965 Barlow and Levick, 1965 The Barlow and Levick DS cells are ON/OFF ganglion cells. They have dendrites that terminate in both the inner part of the IPL and the outer part of the IPL (accounting for the Off response and the On response). Broad tuning to both temporal frequency and spatial frequency, but with a preference for temporal tuning (i.e. Reichardt detector) 4 sub types defined by direction (D  V; V  D; A  P; P  A) ON DS ganglion cells. Dendrites only in On sublamina of IPL OFF DS ganglion cells. Dendrites only in the Off sublamina of IPL. Borst and Euler, 2011 Borst and Euler, 2011 4