1
play

1 Prof. S. Ben-Yaakov , DC-DC Converters [1-4] Example (Cont.) % - PDF document

Sep 16, 2023 •319 likes •388 views

Prof. S. Ben-Yaakov , DC-DC Converters [1-1] The problem of Power Conversion Electricity Electricity Power AC-DC Rectifier Conversion DC-DC Power Type A Type B converter Uniderectional Power DC-AC Inverter Or AC-AC

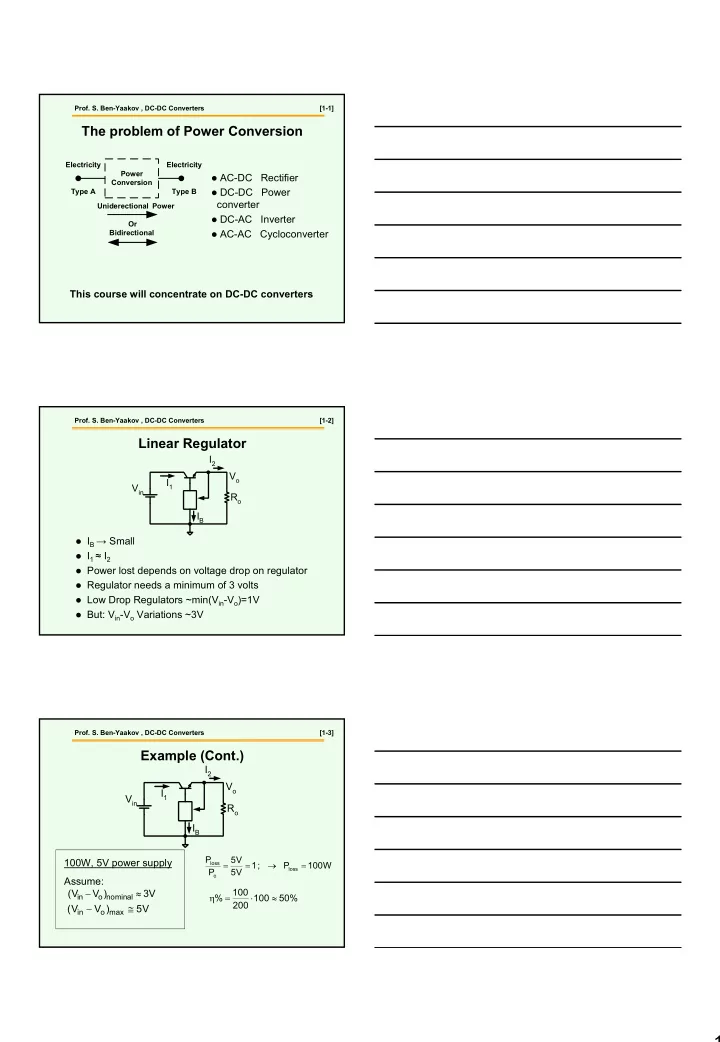
  1. Prof. S. Ben-Yaakov , DC-DC Converters [1-1] The problem of Power Conversion Electricity Electricity Power � AC-DC Rectifier Conversion � DC-DC Power Type A Type B converter Uniderectional Power � DC-AC Inverter Or � AC-AC Cycloconverter Bidirectional This course will concentrate on DC-DC converters Prof. S. Ben-Yaakov , DC-DC Converters [1-2] Linear Regulator I 2 V o I 1 V in R o I B � I B → Small � I 1 ≈ I 2 � Power lost depends on voltage drop on regulator � Regulator needs a minimum of 3 volts � Low Drop Regulators ~min(V in -V o )=1V � But: V in -V o Variations ~3V Prof. S. Ben-Yaakov , DC-DC Converters [1-3] Example (Cont.) I 2 V o I 1 V in R o I B P 5 V 100W, 5V power supply = = → = loss 1 ; P 100 W loss P 5 V o Assume: − ≈ ( V V ) 3 V 100 η = ⋅ ≈ in o no min al % 100 50 % 200 ( V − V ) ≅ 5 V in o max 1

  2. Prof. S. Ben-Yaakov , DC-DC Converters [1-4] Example (Cont.) η % = 50 % It is 100W ! The problem is not • Battery -> efficiency • Line operation -> heat dissipation • Cooling -> size, expense Prof. S. Ben-Yaakov , DC-DC Converters [1-5] Modern Power Conversion Systems Requirements Source Load S � High efficiency L � Small size C � Cost L, C: Reactive elements S: “On” Resistance → 0 Low Losses “Off” Resistance → ∞ Prof. S. Ben-Yaakov , DC-DC Converters [1-6] Disadvantages � More Expensive (in general) � Noisy � Less Reliable � Switching Losses Switching Size frequency 500kHz Power 100kHz Switching level frequency 1kHz 10 2 W 10 4 W 10 6 W 200 kHz 2

  3. Prof. S. Ben-Yaakov , DC-DC Converters [1-7] PWM Prof. S. Ben-Yaakov , DC-DC Converters [1-8] Inductor_1 V dI = V L dt L I In most Power Electronics cases V=constant over time period of interest ∆ I = V V ∆ = ∆ ; I t ; ∆ t L L L Slope=V/L I + V in I - t Prof. S. Ben-Yaakov , DC-DC Converters [1-9] Inductor _2 V L V L V 1 V 2 V 1 V 2 I L I L V L V 2 V L V 1 V 1 t V 2 I L V 1 I L V 1 L L V 2 V 2 L t L t s t s 3

  4. Prof. S. Ben-Yaakov , DC-DC Converters [1-10] Inductor_3 V L V L V 1 V 1 I L I L real case V L V L V 1 V 1 V 2 t t I L I L V 1 V 1 L L t t s t s Prof. S. Ben-Yaakov , DC-DC Converters [1-11] Average Signals dI = V Most important equation in Power Electronics: dt L d I V = Correct for average too: dt L V V m V _ L V t on V 1 t s t t T s V 2 I L T 1 d I ∫ = X Xdt 2 T dt d I 0 1 X - average t s dt t ⋅ V t = = V m on V D m on T s Prof. S. Ben-Yaakov , DC-DC Converters [1-12] Implication For any practical system in steady state: Average voltage on inductor V L = 0 Proof: V L ≠ → ∞ 0 I If then L That is: System must be designed such that: V L = 0 4

  5. Prof. S. Ben-Yaakov , DC-DC Converters [1-13] Inductor current interruption L I L dI S spark = ∞ dt dI I L V in = ∞ → = ∞ V t L dt t S V L V 1 t t S − ∞ Prof. S. Ben-Yaakov , DC-DC Converters [1-14] Inductor current interruption What is the polarity? The imaginary resistor method L S V L I L V 1 V in R t t S Current continuity − ∞ 5

Recommend

More recommend