Z 01010 B = P + Z 01 P + Z 0101 P 1 + 2 zB ( z ) = B ( z ) + P ( z - PowerPoint PPT Presentation

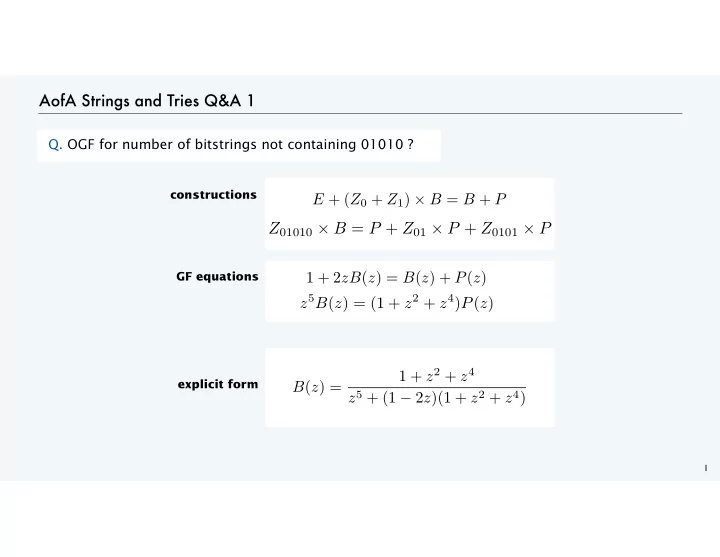
AofA Strings and Tries Q&A 1 Q. OGF for number of bitstrings not containing 01010 ? constructions E + ( Z 0 + Z 1 ) × B = B + P Z 01010 × B = P + Z 01 × P + Z 0101 × P 1 + 2 zB ( z ) = B ( z ) + P ( z ) GF equations z 5 B ( z ) = (1 + z 2 + z 4 ) P ( z ) 1 + z 2 + z 4 explicit form B ( z ) = z 5 + (1 − 2 z )(1 + z 2 + z 4 ) 1

AofA Strings and Tries Q&A 2 Q. Rank these patterns by expected wait time in a random bit string. 62 00000 00001 32 01000 34 2 P c (1 / 2) 01010 36 10101 36 2

AofA Words and Mappings Q&A 1 Q. Find the probability that a random mapping has no singleton cycles . C = Z ? SET ( C ) M = SET ( CY C > 1 ( C )) constructions e − C ( z ) 1 ⇣ ⌘ C ( z ) = ze C ( z ) M ( z ) = exp ln 1 − C ( z ) − C ( z ) = EGF equations 1 − C ( z ) coefficients via 
 Lagrange inversion NOT AN EXAM QUESTION 
 ✗ (too much calculation) asymptotic 
 result 3

AofA Words and Mappings Q&A 1 (improved) Q. Give the EGF for random mappings with no singleton cycles . 
 C ( z ) = ze C ( z ) Express your answer as a function of the Cayley function C = Z ? SET ( C ) M = SET ( CY C > 1 ( C )) constructions 1 ⇣ ⌘ C ( z ) = ze C ( z ) M ( z ) = exp ln 1 − C ( z ) − C ( z ) EGF equations e − C ( z ) = 1 − C ( z ) 4

AofA Words and Mappings Q&A 1 (another version) Q. Find the probability that a random mapping has no singleton cycles . 
 Hint : Do not use generating functions. A. Each entry can have any value but its own index, so the number of 
 N -mappings with no singleton cycles is ( N − 1) N ( N − 1) N 1 − 1 � N � = N N N ∼ 1 e 5

Related problems (stay tuned) Q. Find the probability that a random mapping has no singleton or doubleton cycles . EGF probability (asymptotic) e − C ( z ) e − 1 M 1 ( z ) = all cycle lengths > 1 1 − C ( z ) M 2 ( z ) = e − C ( z ) − C ( z ) 2 / 2 all cycle lengths > 2 e − 3 / 2 1 − C ( z ) Rigorous proof requires full mechanism of singularity analysis in the complex plane (stay tuned) 6

Q&A example: cyclic bitstrings Def. A cyclic bitstring is a cycle of bits 0 1 0 0 0 1 1 0 0 1 1 0 0 1 0 0 1 1 0 0 1 0 0 1 1 1 0 0 0 1 1 C 1 = 2 1 0 1 1 0 0 1 C 3 = 4 1 0 1 1 C 2 = 3 1 0 C 4 = 6 C 5 = 8 Q. How many N -bit cyclic bitstrings ? 8

Q&A example: cyclic bitstrings Q. How many N -bit cyclic bitstrings ? 0 1 One possibility 0 0 1 1 • Solution is “easy”. • Create an exam question with appropriate hints. 0 1 0 1 Another possibility 1 0 0 1 • Solution is “difficult” or “complicated”. 0 1 • Figure out a way to simplify. 0 0 • Or, think about a different problem. 1 0 1 1 1 0 Third possibility • Problem you thought of is a “classic”. C 4 = 6 • Use OEIS. 9