y ( y log x x a ) a is equivalent to f(x) = log a x - PowerPoint PPT Presentation

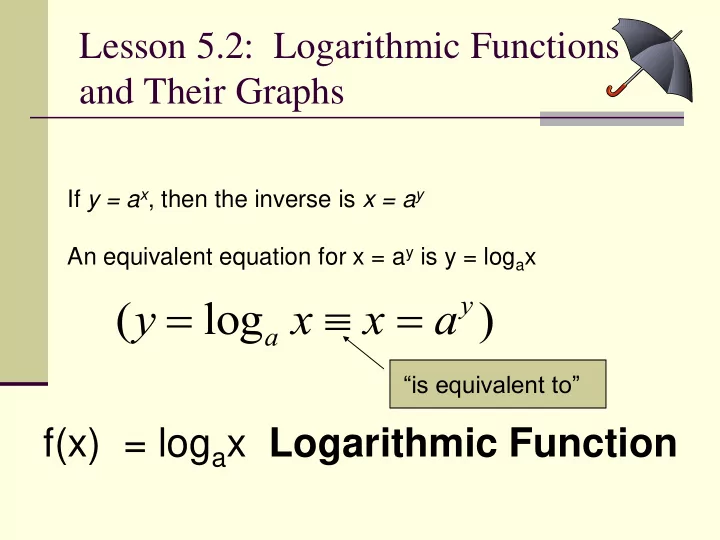
Lesson 5.2: Logarithmic Functions and Their Graphs If y = a x , then the inverse is x = a y An equivalent equation for x = a y is y = log a x    y ( y log x x a ) a “is equivalent to” f(x) = log a x Logarithmic Function

   y y log x x a a Ex 1: Evaluate. a)f(x) = log 2 x, when x = 32  y  5 y  log 2 32 y 32 2 b)f(x) = log 3 x, when x = 1 y  0  y  log 3 1 y 1 3 c)f(x) = log 4 x, when x = 2 y  1 y  log 4 2  y 2 4 2

Common Log Function: f(x) = log 10 x Ex 2: Evaluate f(x) = log 10 x when 1  f x ( ) log a) x = 10 c) x = 1/3 10 3  f x ( ) log 10 10   0 f x ( ) .477 ( )  1 f x b) x = 2.5 d) x = -2   f x ( ) log ( 2 )  f x ( ) log 10 2 5 . 10  0 398 f x ( ) . No Re al solution

Properties of Logarithms  1 .) log 1 0  0 ( Because 1 a ) a  1  ( Because a a ) 2 .) log a 1 a   x log x 3 .) log a a x a a   4 .) If log x log y then x , y . a a

Ex 3: Use the properties of logs. a)Solve for x. log 2 x = log 2 3 x  3   If log x log y then x , y . a a b)Solve for x. log 4 4 = x x  1 log a a  1 x  c)Simplify. log 5 5 x x log a a x  log a x a x log 6 20 20 d ) 6

Natural Log Function When f(x) = e x , then the inverse is x = e y . f(x) = log e x = ln x Ex 4: Use log properties to simplify. 1  0 ) ln 1 a ) ln 3  0  c  log e e 1 e 3   1  2 1 ( )  2 d ) 2 ln e  e  5 log 5 ln 5 b e ) e

Ex 5: Find the value of x for f(x) = ln x.  ln2  0 693 . a)x = 2  ln . 0 3   1204 . b) x = 0.3   ln( 1 ) No Re al solution c) x = -1   2  0 881 ln( 1 ) . d) x = 1 + sqrt(2) Homework: p.386 #1-29 odd