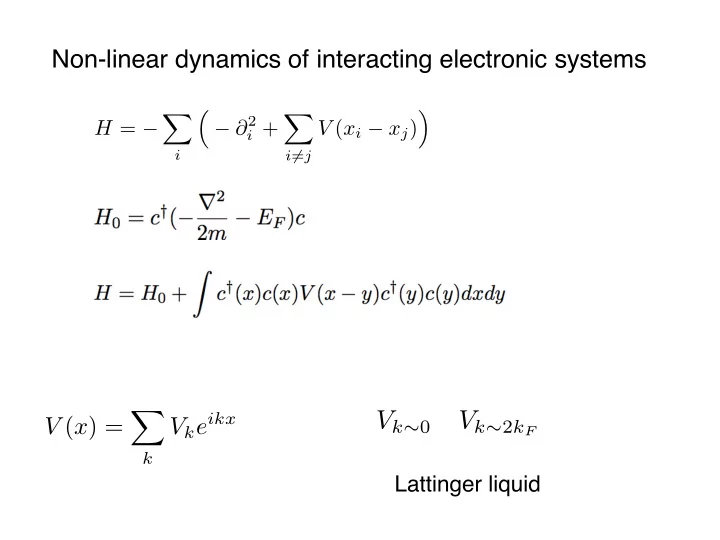

Non-linear dynamics of interacting electronic systems ≥ ¥ X X − ∂ 2 H = − i + V ( x i − x j ) i i 6 = j X V k ∼ 0 V k ∼ 2 k F V k e ikx V ( x ) = k Lattinger liquid
Model Hamiltonian: Elliptic Calogero-Sutherland model ≥ ¥ X X − ∂ 2 H = i + λ ( λ − 1) ℘ ( x i − x j ) i j 1 V ( x ) = ℘ ( x ) → 1 1 ∇ δ ( x ) sin 2 x, x 2 , sinh 2 x, Interpolates between Lattinger liquid and Calogero model - quantum wires, edge states of FQHE The last and the major unsolved integrable model
Free fermions: ϕ + ( ∂ x ϕ ) 2 = 0 ˙ X a k e ikx ρ ( x ) = −∇ x ϕ = ρ 0 + k ρ k = ρ † [ a k , a k 0 ] = kδ k + k 0 , − k
≥ ¥ X X − ∂ 2 H = i + λ ( λ − 1) ℘ ( x i − x j ) i j ϕ = 1 2( ∂ x ϕ ) 2 + ∂ 2 chiral sector ˙ x ˜ ϕ ϕ = 1 cot x − x 0 Z ℘ ( x + iL ) = ℘ ( x ) ϕ ( x 0 ) dx 0 ˜ πL L
Chiral sector - long-time asymptotes of non-linear waves
ϕ = 1 2( ∂ x ϕ ) 2 + ( λ − 1) ∂ 2 ˜ ˙ ϕ ILW-equation ϕ = 1 cot x − x 0 Z ϕ ( x 0 ) dx 0 ˜ πL L ϕ = 1 2( ∂ x ϕ ) 2 + ∂ 3 L → 0 : ˙ x ϕ KdV-equation ϕ = 1 ϕ ( x 0 ) Z 2( ∂ x ϕ ) 2 + ∂ 2 x − x 0 dx 0 L → ∞ : ˙ x Benjamin-Ono equation
On the relation between Calogero model and CFT ϕ = T xy ˙ T xy = ( ∂ x ϕ ) 2 + α 0 ∂ 2 x ˜ ϕ Flux of energy through the boundary √ √ α 0 = λ − 1 / λ λ ( λ − 1) ( x i − x j ) 2
Period of oscillations is (interaction) × ( δρ ) − 1 >> k − 1 F Quantum Non-linear Equations can be treated semiclassically
Trigonometric Calogero-Sutherland model λ ( λ − 1) X X ∂ 2 H = − i + Model for edge states of the FQHE sin 2 ( x i − x j ) i j H Ψ = E Ψ Y ( e ix i − e ix j ) λ J Y ( x 1 , . . . , x N ) Ψ( x 1 , . . . , x N ) = i>j Jack symmetric polynomial Ψ( ...x i ...x j ... ) = e 2 πiλ Ψ( ...x j ...x i ... ) λ = 0 − bosons λ = 1 − fermions
λ ( λ − 1) X X ∂ 2 H = − i + sin 2 ( x i − x j ) i j ϕ = 1 2( ∂ x ϕ ) 2 + ( λ − 1) ∂ 2 ˜ ˙ ϕ e ikx X ϕ ( x ) = [ a k , a − k 0 ] = λkδ kk 0 k a k , k X e ikx i a k> 0 = i
Properties of Benjamin-Ono Equation ϕ + ( ∂ x ϕ ) 2 + ν∂ 2 x ϕ H = 0 ˙ Properties: 1 ) Integrable ( despite being non - local ) ; 2 ) Its solitons carry a quantized fractional charge: Z Z ρ dx = d ϕ = integer × ν 3 ) Solitons have Lorentzian shape: ρ s ( x, t ) = 1 v ν v 2 ( x − vt ) 2 + ν 2 π
Soliton - collective excitation of particles
Shock wave: competition between non - linear term and dispersion term ϕ + ( ∂ x ϕ ) 2 + ν∂ 2 x ϕ H = 0 ˙
Long time asymptote: Soliton train
time Soliton Train space
1/4 of quantum A single soliton ( area is 1/12 ) ( area is 1/3 )
N=7 N=20 Soliton trains
Separation between hole ( moving right ) and particles ( moving left )
Conclusions: 1 ) Dynamics of the edge state is essentially non - linear; 2 ) Solitons of non - linear dynamics carry fractional charge; 3 ) A propagation of any front evolves to a shock wave and further in a fractionally quantized soliton train. Quantum shocks in BEC
Quantum Hydrodynamics of Calogero-Sutherland model λ ( λ − 1) x i = p i ˙ X p i = ˙ ( x i − x j ) 3 j λ λ X X p i = − x i − y k x i − x k k k λ λ X X x i = ˙ − x i − y k x i − x k k k λ λ X X − ˙ y i = − y i − x k y i − y k k k
λ λ X X x i = ˙ − x i − y k x i − x k k k λ λ X X − ˙ y i = − y i − x k y i − y k k k X X ϕ ( z ) = λ log( z − x k ) + λ log( z − y k ) i i X X ϕ ( z ) = λ ˜ log( z − x k ) − λ log( z − y k ) i i ϕ = 1 2( ∂ x ϕ ) 2 + ( λ − 1) ∂ 2 ˜ ˙ ϕ
Density and velocity ≥ ¥ ϕ ( x + i 0) − ϕ ( x − i 0) = − 2 λπρ ( x ) ∂ x ≥ ¥ ϕ ( x + i 0) + ϕ ( x − i 0) = v − 2 iλ∂ x log ρ ∂ x ρ + ∂ x ( ρv ) = 0 ˙
ρ + ∂ x ( ρv ) = 0 ˙ ≥ v 2 ¥ v + ∂ x ˙ 2 + w ( ρ ) = 0 w ( ρ ) = λ 2 π 2 ρ 2 − λ ( λ − 1) 1 √ ρ + πλ ( λ − 1) ∂ x ρ H √ ρ∂ 2 x 2 2 Z ρ H ( x ) = coth( x − x 0 ) ρ ( x 0 ) dx 0
Chiral reduction ≥ πρ + ∂ x (log √ ρ ) H ¥ v = λ two equations become one Chiral non-linear equation ≥ πρ 2 + ρ∂ x (log √ ρ ) H ¥ ρ t + λ∂ x = 0 ρ ≈ ρ 0 + u + . . . u + uu x + ( λ − 1)˜ ˙ u xx = 0 Benjamin-On equation
Recommend
More recommend