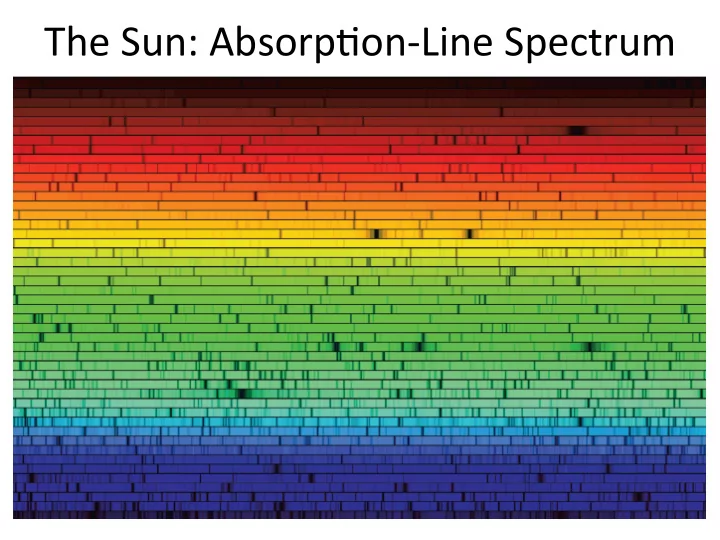
The Sun: Absorp/on-Line Spectrum Atomic spectral lines - PowerPoint PPT Presentation

The ¡Sun: ¡Absorp/on-­‑Line ¡Spectrum ¡

Atomic spectral lines

Kirchhoff’s Laws ¡ • A hot, dense gas or hot solid object produces a continuous spectrum (e.g., a blackbody spectrum). ¡ • A hot, diffuse gas produces bright spectral lines (emission lines), an emission spectrum. ¡ • A cool, diffuse gas in front of a continuous spectrum produces dark spectral lines (absorption lines) in the continuous spectrum, an absorption spectrum. ¡ ¡

Stellar Spectra • Stars emit an absorption spectrum • The hot inner core emits thermal (continuous) radiation • The cooler atoms in the outer atmosphere of the star absorb light at the specific wavelengths corresponding to the transitions within those atoms • The continuous part of this spectrum can be used to find stellar temperatures (Wein’s Law) • We will see soon how the spectral lines can be used to study stellar composition composition

Electron ¡Double-­‑Slit ¡Experiment: ¡ Diffrac/on ¡and ¡Interference ¡ If ¡a ¡screen ¡is ¡placed ¡ here ... ¡ ...we’ll ¡see ¡a ¡pa1ern ¡like ¡this ¡on ¡ the ¡screen ¡

Uncertainty ¡Principle ¡ position x momentum p uncertainty σ 2 ¡“probability ¡waves” ¡in ¡space: ¡ p = h / λ known! ¡ A ¡compromise! ¡ Superposi9on ¡of ¡ many ¡sine ¡waves. ¡ But ¡ x NOT ¡known ¡at ¡all! ¡

Visualizing ¡Electron ¡Orbitals ¡in ¡Hydrogen-­‑Like ¡Atoms ¡

Some ¡energy ¡levels ¡of ¡He ¡(the ¡next-­‑ simplest ¡atom ¡aRer ¡H) ¡

Example ¡problem: ¡Electrons ¡as ¡de ¡Broglie ¡Waves ¡ Complete ¡and ¡hand ¡in ¡for ¡par9cipa9on ¡credit ¡ Let’s ¡model ¡the ¡electron ¡in ¡a ¡ hydrogen ¡atom ¡as ¡a ¡de ¡Broglie ¡wave. ¡ The ¡circumference ¡of ¡the ¡electron’s ¡ orbit ¡must ¡equal ¡an ¡integer ¡number ¡of ¡ wavelengths, ¡ nλ ¡ (see ¡figure ¡at ¡right). ¡ ¡ (a) ¡ Why ¡must ¡this ¡quan/zed ¡ wavelength ¡requirement ¡be ¡obeyed? ¡ Explain ¡in ¡a ¡sentence ¡or ¡two. ¡ ¡ (b) ¡ Show ¡that ¡this ¡requirement ¡leads ¡ to ¡Bohr’s ¡quan/zed ¡angular ¡ momentum, ¡ L ¡= ¡nh/2π. ¡