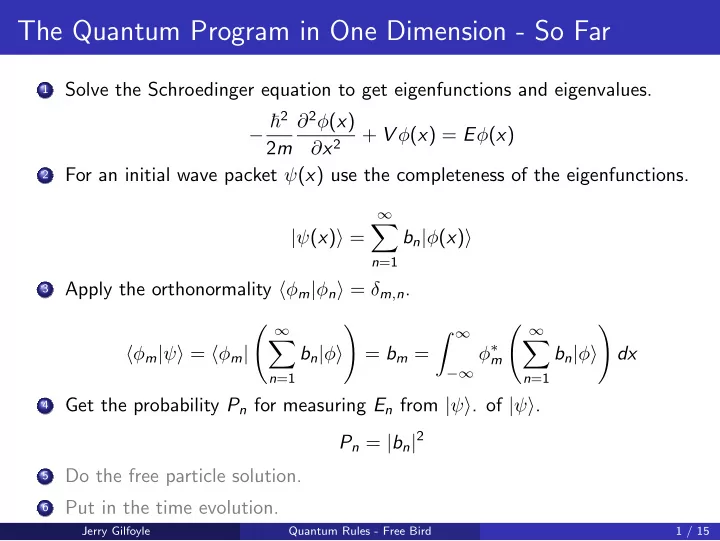
The Quantum Program in One Dimension - So Far Solve the Schroedinger - PowerPoint PPT Presentation

The Quantum Program in One Dimension - So Far Solve the Schroedinger equation to get eigenfunctions and eigenvalues. 1 − � 2 ∂ 2 φ ( x ) + V φ ( x ) = E φ ( x ) ∂ x 2 2 m For an initial wave packet ψ ( x ) use the completeness of the eigenfunctions. 2 ∞ � | ψ ( x ) � = b n | φ ( x ) � n =1 Apply the orthonormality � φ m | φ n � = δ m , n . 3 � ∞ � ∞ � ∞ � � � � φ ∗ � φ m | ψ � = � φ m | b n | φ � = b m = b n | φ � dx m −∞ n =1 n =1 Get the probability P n for measuring E n from | ψ � . of | ψ � . 4 P n = | b n | 2 Do the free particle solution. 5 Put in the time evolution. 6 Jerry Gilfoyle Quantum Rules - Free Bird 1 / 15

The Free Particle Problem Consider a free particle ( V = 0) which has an initial wave packet that is described by a gaussian function. 1 (2 πσ 2 ) 1 / 4 e − x 2 / 4 σ 2 | Ψ( x , 0) � = What is the spectrum of momenta that form this wave packet? How wide is that distribution? The Initial Gaussian Wave 0.5 0.4 0.3 2 | ψ 0.2 0.1 0.0 - 4 - 2 0 2 4 x Jerry Gilfoyle Quantum Rules - Free Bird 2 / 15

2.0 red - x = 2.0 green - x = 0.5 1.5 blue - x = 0.2 Sin ( Δ k x )/ Δ k 1.0 0.5 0.0 - 0.5 - 20 - 10 0 10 20 Δ k Jerry Gilfoyle Quantum Rules - Free Bird 3 / 15

Jerry Gilfoyle Quantum Rules - Free Bird 4 / 15

� ∆ kmax sin(∆ k x ) lim d (∆ k ) x →∞ ∆ k − ∆ kmax x ∆ k max Integral 0.01 10000 3.12445 1.0 10000 3.14178 2.0 10000 3.14151 4.0 10000 3.14158 10.0 10000 3.14161 100.0 10000 3.14159 1000.0 10000 3.14159 10000.0 10000 3.14159 100000.0 10000 3.14159 Jerry Gilfoyle Quantum Rules - Free Bird 4 / 15

The Dirac Delta Function � ∆ kmax sin(∆ k x ) lim d (∆ k ) x →∞ ∆ k − ∆ kmax x ∆ k max Integral 0.01 10000 3.12445 1.0 10000 3.14178 2.0 10000 3.14151 4.0 10000 3.14158 10.0 10000 3.14161 100.0 10000 3.14159 1000.0 10000 3.14159 10000.0 10000 3.14159 100000.0 10000 3.14159 Jerry Gilfoyle Quantum Rules - Free Bird 5 / 15

Dirac Delta Function Demonstration 1.2 Use straight � ∆ k max 1.0 sin(∆ k x ) line for b ( k ) 2 b ( k ) lim d (∆ k ) = Input Functions 0.8 Dirac δ ∆ k x →∞ − ∆ k max 0.6 representation � ∆ k max sin(∆ k x ) 0.4 2 b ( k ′ ) lim d (∆ k ) ∆ k x →∞ 0.2 − ∆ k max 0.0 - 0.2 2 b ( k = 0) = 1 . 0 - 30 - 20 - 10 0 10 20 30 Δ k x on l . h . s . ∆ k max l . h . s r . h . s 0.01 1000 3.31670 3.14159 0.6 Product of input 1.0 1000 3.14047 3.14159 Output Function functions 0.4 2.0 1000 3.14196 3.14159 10.0 1000 3.14178 3.14159 0.2 100.0 1000 3.14161 3.14159 0.0 1000.0 1000 3.14159 3.14159 10000.0 1000 3.14159 3.14159 - 0.2 100000.0 1000 3.14159 3.14159 - 30 - 20 - 10 0 10 20 30 Δ k Jerry Gilfoyle Quantum Rules - Free Bird 6 / 15

Comparison of Bound and Free Particles Particle in a Box Free Particle The potential The potential V =0 0 < x < a V = 0 = ∞ otherwise Eigenfunctions and eigenvalues Eigenfunctions and eigenval- ues E = � 2 k 2 1 e ± ikx | φ ( k ) � = √ 2 m � E n = n 2 � 2 π 2 2 π 2 � n π x � | φ n � = a sin 2 ma 2 a Superposition � ∞ Superposition | ψ � = b ( k ) φ ( k ) dk ∞ −∞ � | ψ � = b n | φ n � � φ m | φ n � = δ m , n � φ ( k ′ ) | φ ( k ) � = δ ( k − k ′ ) n =1 Getting the coefficients Getting the coefficients P n = | b n | 2 P ( k ) dk = | b ( k ) | 2 dk b ( k ) = � φ ( k ) | ψ � b n = � φ n | ψ � Jerry Gilfoyle Quantum Rules - Free Bird 7 / 15

The Free Particle Problem Consider a free particle ( V = 0) which has an initial wave packet that is described by a gaussian function. 1 (2 πσ 2 ) 1 / 4 e − x 2 / 4 σ 2 | Ψ( x , 0) � = What is the spectrum of momenta that form this wave packet? How wide is that distribution? The Initial Gaussian Wave 0.5 0.4 0.3 2 | ψ 0.2 0.1 0.0 - 4 - 2 0 2 4 x Jerry Gilfoyle Quantum Rules - Free Bird 8 / 15

From The Homework (3.10) In the solution to 3.10 (∆ x ) 2 = � x 2 � − � x � 2 � x 2 � = a 2 + x 2 � x � 2 = x 2 and and 0 0 so (∆ x ) 2 = a 2 + x 2 0 − x 2 0 = a 2 Jerry Gilfoyle Quantum Rules - Free Bird 9 / 15

∞  From The Homework (3.10) Effect of Changing σ on Gaussian Shape 0.5 Blue: σ = 0.6 f ( x ) ⅆ x = 1 Green: σ = 1.2 - ∞ 0.4 Red: σ = 2.4 x 0 = 0 0.3 f ( x ) 0.2 0.1 0.0 - 10 - 5 0 5 10 x Jerry Gilfoyle Quantum Rules - Free Bird 10 / 15

Initial Wave Packet and the Spectral Distribution The Initial Gaussian Wave 0.5 0.4 0.3 2 | ψ 0.2 0.1 0.0 - 4 - 2 0 2 4 x Spectral Distribution 0.8 2 0.6 b ( k ) 0.4 0.2 0.0 - 4 - 2 0 2 4 k ( inverse length ) Jerry Gilfoyle Quantum Rules - Free Bird 11 / 15

Probabilities of Different Final States Rectangular Wave in a Square Well 0.4 a = 1.0 Å x 0 = 0.3 Å 0.3 x 1 = 0.5 Å Probability 0.2 0.1 0.0 0 500 1000 1500 2000 2500 3000 Energy ( eV ) Jerry Gilfoyle Quantum Rules - Free Bird 12 / 15

Spectral Distribution for One-Dimensional Nuclear Fusion Nuclear Fusion 0.6 0.5 0.4 2 0.3 | b n 0.2 0.1 0.0 0 50 100 150 200 E n ( units of E 1 ) Jerry Gilfoyle Quantum Rules - Free Bird 13 / 15

Spectral Distribution for One-Dimensional Nuclear Fusion Nuclear Fusion 0.6 0.5 0.4 2 0.3 | b n 0.2 0.1 0.0 0 200 400 600 800 1000 E n ( units of E 1 ) Jerry Gilfoyle Quantum Rules - Free Bird 14 / 15

Spectral Distribution for One-Dimensional Nuclear Fusion Nuclear Fusion 1 Only non - zero values b n = 0 for n even, except n = 8 0.100 0.010 2 | b n 0.001 10 - 4 10 - 5 0 200 400 600 800 1000 E n ( units of E 1 ) Jerry Gilfoyle Quantum Rules - Free Bird 15 / 15