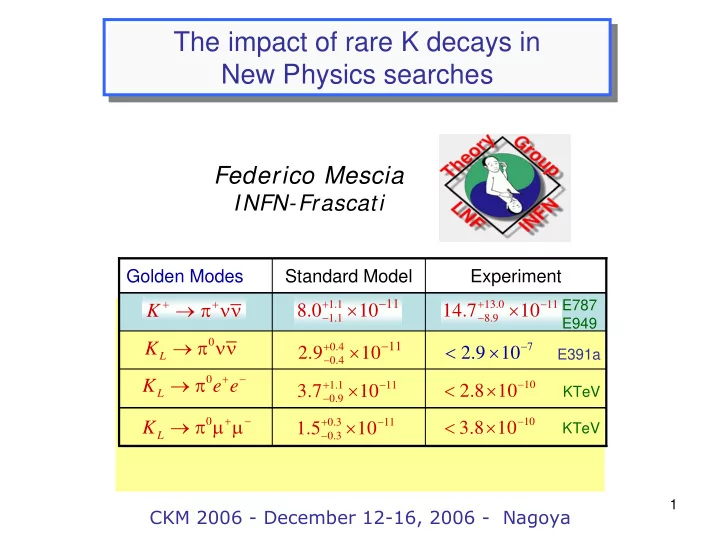

The impact of rare K decays in The impact of rare K decays in New Physics searches New Physics searches Federico Mescia INFN-Frascati Golden Modes Standard Model Experiment − + + + + − → π νν × × 11 E787 1.1 13.0 11 K 8.0 10 14.7 10 − − 1.1 8.9 E949 K → π νν 0 − + 2.9 10 − × < × 11 0.4 7 2.9 10 E391a L − 0.4 → π + − 0 2.8 10 − + × − < × K e e 1.1 11 10 3.7 10 KTeV L − 0.9 → π µ µ + − 3.8 10 − + × − < × 0 0.3 11 10 K 1.5 10 KTeV − L 0.3 1 CKM 2006 - December 12-16, 2006 - Nagoya
6 6 K L →πνν ÷ K + →πνν: uncertainties at 15% due to present CKM accuracy 6 0 0 0 0 0 0 2 2 2 + + + − →π νν = × 13.0 11 B K ( ) 14.7 10 [E787-E949] − 8.9 2 → π νν ≤ + → π νν + 0 B K ( ) 4.4 ( B K ) [Grosm ann - Nir Bound] L
at 5% uncertainties with CKM updates from Babar/Belle/LHCb 0 0 0 1 1 1 0 0 0 2 2 K L →π 0 νν − K + →π + νν: high-precision discovery lens! 2 large room for New Physics essential exp. info 3
large unexplored room in principle, but 1 is it still possible to expect deviations, despite constraints from the large • amount of processes compatible with the Standard Model? reminder tree-level processes → disfavoured for NP searches, normally (M W / Λ ) 2 ≤ 1% � ( Mind at special cases talk ) Mind at special cases, , Paride’s Paride’s talk FCNC loop processes → suitable for NP, only measured ∆ B= 2, ∆ S= 2 and ∆ B= 1 � transitions K rare decays → s → d coupling and highest CKM suppression → like ε ’ /ε � very clean → like sin2 β 2 in any case, LHC will saturate the room left, won’t it? ATLAS-CMS → new particles at the TeV scale by flavour conserving channels � complementarity information @TeV LHCb at work → B s →µµ and B d →µµ, information on b → s/b → d couplings � K →πνν & K →π �� can give some surprise, with small effects in B and EWPT Moreover, clean probe to higher scale Λ flav ~100 TeV 4 Let’s not forget “The definitive answer is from experiments” G. Galileo G. Galileo
Two classes of “ Beyond SM” scenarios: 1. Minimal Flavour Violation: 2. New sources of Flavour Symmetry breaking arising at the TeV scale flavour breaking induced only by SM Yukawa couplings, Y U & Y D. ( Y: Wilson coefficient at Λ flav » 1 TeV ) • s → d new couplings no longer O ( λ 5 ) suppressed • SM hierarchy of FV couplings: (s → d) MFV = O ( λ 5 ) × [ SM + new d.o.f ] (s → d) BMFV = O ( λ 5 ) × SM + O ( 1 ) × (new d.o.f) • Specific realisations in SUSY, UED, LH, • Many proposed models already killed from EFT present data (B, K, EWPT & DM) • Small deviations in specific models: B (K L →π 0 νν) ≤ O (20%-30%) • One order of magnitude enhancement still → Cecilia & Buras possible in MSSM and LHT B (K L →π 0 νν) ≤ 510 −10 • In specific models, stringent correlations can rise with either B physics ( B → �� , in reach of E391a upgrade B → X �� , B → X νν) or EWPT ( ∆ρ ) Pattern : effects on B( K L →π 0 νν) > B ( K + →π + νν) > B ( K L →π 0 �� ) 5 →π π 0 µµ − − K →π π 0 correlation L → 0 µµ L → 0 ee ee correlation Peculiarity: Peculiarity: K K L K L
Two classes of “ Beyond SM” scenarios: 1. Minimal Flavour Violation: 2. New sources of Flavour Symmetry breaking arising at the TeV scale flavour breaking induced only by SM Yukawa couplings, Y U & Y D. ( Y: Wilson coefficient at Λ flav » 1 TeV ) • s → d new couplings no longer O ( λ 5 ) suppressed • SM hierarchy of FV couplings: (s → d) MFV = O ( λ 5 ) × [ SM + new d.o.f ] (s → d) BMFV = O ( λ 5 ) × SM + O ( 1 ) × (new d.o.f) • Specific realisations in SUSY, UED, LH, • Many proposed models already killed from EFT present data (B, K, EWPT & DM) • Small deviations in specific models: B (K L →π 0 νν) ≤ O (20%-30%) • One order of magnitude enhancement still → Cecilia & Buras possible in MSSM and LHT B (K L →π 0 νν) ≤ 510 −10 • In specific models, stringent correlations can rise with either B physics ( B → �� , in reach of E391a upgrade B → X �� , B → X νν) or EWPT ( ∆ρ ) Pattern : effects on B( K L →π 0 νν) > B ( K + →π + νν) > B ( K + →π + �� ) 6 →π π 0 µµ − − K →π π 0 correlation L → 0 µµ L → 0 ee ee correlation Peculiarity: Peculiarity: K K L K L
Two classes of “ Beyond SM” scenarios: 1. Minimal Flavour Violation: 2. New sources of Flavour Symmetry breaking arising at the TeV scale flavour breaking induced only by SM Yukawa couplings, Y U & Y D. ( Y: Wilson coefficient at Λ flav » 1 TeV ) • s → d new couplings no longer O ( λ 5 ) suppressed • SM hierarchy of FV couplings: (s → d) MFV = O ( λ 5 ) × [ SM + new d.o.f ] (s → d) BMFV = O ( λ 5 ) × SM + O ( 1 ) × (new d.o.f) • Specific realisations in SUSY, UED, LH, • Many proposed models already killed from EFT present data (B, K, EWPT & DM) • Small deviations in specific models: B (K L →π 0 νν) ≤ O (20%-30%) • One order of magnitude enhancement still → Cecilia & Buras possible in MSSM and LHT B (K L →π 0 νν) ≤ 510 −10 • In specific models, stringent correlations can rise with either B physics ( B → �� , in reach of E391a upgrade B → X �� , B → X νν) or EWPT ( ∆ρ ) Pattern : effects on B( K L →π 0 νν) > B ( K + →π + νν) > B ( K + →π + �� ) 7 →π π 0 µµ − − K →π π 0 correlation L → 0 µµ L → 0 ee ee correlation Peculiarity: Peculiarity: K K L K L
Minim al Flavour Violation → U(3) 5 EFT at TeV D’Ambrosio,Giudice,Isidori,Strumia (02) ⋅ 6 6 ∑ c O + + … L = L i i (A ,Q ,H) + Q Y D H Q Y U H + ���� � ����� � Λ eff gauge i i L D R L U R c 2 i fla v Y D & Y U regulate U(3) 5 flavour violation: U(3) 5 flavour group L new d.o.f new d.o.f @ TeV @ TeV SM O 6 → functions of SM fields and Y D -Y U spurions, made invariant of U(3) 5 ≡ SU(3) 5 ⊗ B ⊗ L ⊗ CP. - c 6 → universal and real coef. see Grinstein’s talk 8
Minim al Flavour Violation → U(3) 5 EFT at TeV D’Ambrosio,Giudice,Isidori,Strumia (02) ⋅ 6 6 c O ∑ + + … L = L i i (A ,Q ,H) + Q Y D H Q Y U H + ���� � ����� � Λ eff gauge i i L D R L U R c 2 i fla v K- -rare rare K decays decays 13 operators 13 operators � � ( ) ( ) ( ) ( ) ( ) ( ( ) ( ) ) ⎫ + + + + γ νγ ν + γ γ + τγ τ 6 6 * L * V V X s d V V K s d ll c Q Y Y Q H D H c Q Y Y Q H D H µ µ µ µ µ µ µ ⎪ ts td L ts t d V A / L 1 L U U L 2 L U U L L L V A / � � ( ) ( ) ( ) ( ) ⎪ ( ) ( ) ( ) ( ) + γ γ + + τγ γ τ → γ + γ 6 6 ⎬ * * ll V V K b d ll c Q Y Y Q L L c Q Y Y Q L L V V K b s µ L µ µ L µ µ µ 3 L U U L L 4 L U U L L tb ts V A / L tb td V A / L L L ⎪ V A / V A / ( ) ( ) ( ) ( ) ( ) ( ) + µν + + ⎪ σ + γ γ σ µν + γ γ 6 6 * * c D Y Y Y Q F c Q Y Y Q Q Y Y Q V V m C b s F V V B s d s d ⎭ R µ µ µ R µ µ µ 5 D U U v L 6 L U U L L U U L tb ts b 7 v L ts td L L L L ( ) + ∝ D’Ambrosio,Giudice,Isidori,Strumia (02) ... * 2 2 Y Y V V m / m ti t j U U t W ij K- -rare decays rare decays K →πνν →π ε �� 0 K , K , K 1. CKM suppression ( O ( λ 5 )) still on; → → γ �� B X , B X / / s d s d 2. the X coefficient unbounded from B processes or ε K 2 β * 2 → π νν → π νν V V X sin 0 0 B K ( ) B K ( ) [ ] ∝ × + ε ts td S M � L L 1 sign X + → π νν + [ ] + → π νν + c 2 + ε B K ( ) B K ( ) * V V X 1 sign X SM ts t d c 9
→ π νν → π νν 0 0 MFV B K ( ) B K ( ) [ ] = × + ε L L 1 sign X model + → π νν + + → π νν + c B K ( ) B K ( ) independent ap. MFV SM 10 MFV enhancement: B ( K L →π 0 νν) ≤ 4.6 B SM B ( K + →π + νν)/ B ( K L →π 0 νν) ∼ SM
MFV- Specific Scenarios In a given model implementation, X bounded trough EWPT & B data. Deviations from SM can get smaller 1 . MFV- Phenom enological Model (CMFV) Buras,Gambino,Gorbahn,Jäger,Silvestrni (00) • only Standard Model operators • box and g-peng. d.o.f frozen Bobeth,Bona,Buras,Ewerth,Pierini,Silvetrini,Weiler (05) to their SM value ⎫ 2 →πνν → → γ ≅ ⎧ ⎛ ⎞ e f f ⎪ ⎪ = ≅ B ( K ) X B B ( X ) C Y E 0 Y X ⎯⎯⎯⎯⎯ → ⎯⎯⎯ ⎯⎯ → s 7 ⎬ ⎨ ⎜ ⎟ Model d. Model d. →π → ⎪ ⎝ ⎠ �� Z ⎩ ⎪ → → B K ( ) Y Z , , E �� ef f ⎭ B B ( X ) Y , , , Z E C s 7 X -> constrained by B processes X -> constrained by B processes = + νν ll Y X B - B gauge invariant ( ) 1 νν = + - 4 Z X D B s.d. couplings γ 4 11
Recommend
More recommend