Summary Basics how the oscillator works heuristic approach to the - PowerPoint PPT Presentation

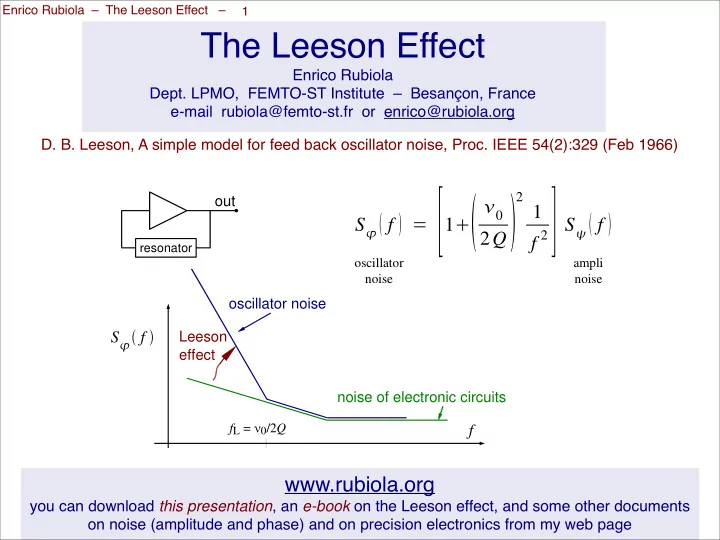
Enrico Rubiola – The Leeson Effect – 1 The Leeson Effect Enrico Rubiola Dept. LPMO, FEMTO ­ ST Institute – Besançon, France e ­ mail rubiola@femto ­ st.fr or enrico@rubiola.org D. B. Leeson, A simple model for feed back oscillator noise, Proc. IEEE 54(2):329 (Feb 1966) S ! " f # $ % 1 & " f 2 ( S ) " f # 2 Q # 2 out ' 0 1 resonator oscillator ampli noise noise oscillator noise S ! " f # Leeson effect noise of electronic circuits f L = ! 0 /2 Q f www.rubiola.org you can download this presentation , an e-book on the Leeson effect, and some other documents on noise (amplitude and phase) and on precision electronics from my web page

Enrico Rubiola – The Leeson Effect – 2 Summary ● Basics • how the oscillator works • heuristic approach to the Leeson effect • analysis of commercial oscillators • Theory • Laplace transforms • proof of the Leeson formula • Advanced topics • detuned resonator • delay-line oscillator • phase noise in lasers

Enrico Rubiola – The Leeson Effect – 3 Basics Oscillator

basics – oscillator Enrico Rubiola – The Leeson Effect – 4

basics – oscillator Enrico Rubiola – The Leeson Effect – 5 ω = 2 πν

basics – oscillator Enrico Rubiola – The Leeson Effect – 6

Enrico Rubiola – The Leeson Effect – 7 Basics Heuristic approach to the Leeson effect

basics – heuristic approach Enrico Rubiola – The Leeson Effect – 8

basics – heuristic approach Enrico Rubiola – The Leeson Effect – 9

basics – heuristic approach Enrico Rubiola – The Leeson Effect – 10

basics – heuristic approach Enrico Rubiola – The Leeson Effect – 11

basics – heuristic approach Enrico Rubiola – The Leeson Effect – 12

basics – heuristic approach Enrico Rubiola – The Leeson Effect – 13

basics – heuristic approach Enrico Rubiola – The Leeson Effect – 14 Resonator instability The resonator instability may be larger than the noise of the electronics (high-performance xtal oscillators) Type 1 f L > f c Type 2 f L < f c frequency rw frequency rw of the resonator of the resonator total noise total noise frequency flicker lower − noise resonat. S ϕ (f) ϕ (f) frequency flicker of the resonator of the resonator S Leeson effect intersection f > f L intersection amplifier f > f L Leeson effect amplifier f f f c f f L f c L

basics – heuristic approach Enrico Rubiola – The Leeson Effect – 15 r. w. freq. S ϕ (f) f −4 b −4 f −3 b −3 phase noise flicker freq. white freq. f −2 b −2 flicker phase. f −1 b −1 b 0 white phase f f 2 / 2 ν 0 x S y (f) h 2 f 2 f −2 h −2 frequency noise white phase f −1 r. w. freq. h −1 h 1 f h 0 flicker phase flicker freq. white freq. f Allan variance σ (τ) 2 y white σ 2 ( τ ) = h 0 /2 τ freq. flicker σ 2 ( τ ) = 2ln(2) h -1 drift flicker phase white phase r.walk σ 2 ( τ ) = ((2 π ) 2 /6) h 0 τ white freq. r. w. freq. flicker freq. τ

Enrico Rubiola – The Leeson Effect – 16 Basics Analysis of some commercial oscillators The purpose of this section is to help to understand the oscillator inside from the phase noise spectra, plus some technical information. I have chosen some commercial oscillators as an example. The conclusions about each oscillator represent only my understanding based on experience and on the data sheets published on the manufacturer web site. You should be aware that this process of interpretation is not free from errors. My conclusions were not submitted to manufacturers before writing, for their comments could not be included. I have modified some the web pages reproduced here, with the only purpose of making “logos” visible after zooming in. Please look for the original on the manufacturer web page.

basics – commercial oscillators Enrico Rubiola – The Leeson Effect – 17 Courtesy of Miteq (handwritten notes are mine). The DRO test data are available at the URL http://www.miteq.com/micro/fresourc/d210b/dro/droTyp.html

basics – commercial oscillators Enrico Rubiola – The Leeson Effect – 18 Poseidon Scientific Instruments − Shoebox 10 GHz sapphire whispering-gallery resonator oscillator −90 Poseidon Shoebox 10 GHz sapphire WG resonator −100 noise correction −110 oscillator phase noise, dBc/Hz −120 f −3 b −3 −130 −140 d ~ 6dB −150 instrument background f −1 b −1 −160 −170 −180 f L =2.6kHz 100 1000 10000 100000 Fourier frequency, Hz f L = v 0 /2Q = 2.6 kHz → Q = 1.8 × 10 6 This incompatible with the resonator technology. Typical Q of a sapphire whispering gallery resonator: 2 × 10 5 @ 295K (room temp), 3 × 10 7 @ 77K (liquid N), 4 × 10 9 @ 4K (liquid He). In addition, d ~ 6 dB does not fit the power-law. The interpretation shown is wrong, and the Leeson frequency is somewhere else. the plot is reconstructed from data available on the manufacturer's web site http://www.psi.com.au

basics – commercial oscillators Enrico Rubiola – The Leeson Effect – 19 Poseidon Scientific Instruments − Shoebox 10 GHz sapphire whispering-gallery resonator oscillator −90 Poseidon Shoebox 10 GHz sapphire WG resonator −100 noise correction f −1 to f −3 conversion −110 phase noise, dBc/Hz oscillator −120 f c =850Hz f 0 to f −2 −130 conversion ) buffer =−120dBrad 2 /Hz (b −1 −140 ) ampli =−140dBrad 2 /Hz (b −1 −150 (b 0 ) ampli =−169 instr. background −160 dBrad 2 /Hz −170 −180 f L =25kHz 100 1000 10000 100000 Fourier frequency, Hz The 1/f noise of the output buffer is higher than that of the sustaining amplifier (a compex amplifier with interferometric noise reduction) In this case both 1/f and 1/f 2 are present white noise − 169 dBrad 2 /Hz, guess F = 5 dB (interferometer) → P 0 = 0 dBm buffer flicker − 120 dBrad 2 /Hz @ 1 Hz → good microwave amplifier f L = v 0 /2Q = 25 kHz → Q = 2 × 10 5 (quite reasonable) f c = 850 Hz → flicker of the interferometric amplifier − 139 dBrad 2 /Hz @ 1 Hz the plot is reconstructed from data available on the manufacturer's web site http://www.psi.com.au

basics – commercial oscillators Enrico Rubiola – The Leeson Effect – 20 Poseidon Scientific Instruments 10 GHz dielectric resonator oscillator (DRO) Phase noise of two PSI DRO−10.4−FR −50 −30dB/dec −60 dBrad 2 /Hz b −3 =+4 SSB phase noise, dBc/Hz −70 D R O −80 D − 1 R 0 O . 4 − − 1 −90 F 0 R . slope close to −25dB/dec 4 − 7dB X P −100 L −110 slope −120 −25 dB/dec −20dB/dec −130 −140 3dB difference b −1 =−165dBrad 2 /Hz −150 −160 b 0 =−165dBrad 2 /Hz −170 −180 10 2 10 3 10 4 10 5 10 6 10 7 f c =9.3kHz Fourier frequency, Hz f L =3.2MHz Slopes are not in agreement with the theory. In this case I am unable to say what is going on inside the oscillator. the plot is reconstructed from data available on the manufacturer's web site http://www.psi.com.au

basics – commercial oscillators Enrico Rubiola – The Leeson Effect – 21 Oscilloquartz OCXO 8600 outstanding stability oscillator based on a 5 MHz AT-cut BVA (electrodless) resonator # y ( $ ) = 3 × 10-13 for $ = 0.2 ÷ 30 s stability 3 × 10 -12 /day aging Courtesy of Oscilloquartz (handwritten notes are mine). The specifications, which include this spectrum, are available at the URL http://www.oscilloquartz.com/file/pdf/8600.pdf ANALYSIS ! 1 – floor S ! 0 = –155 dBrad 2 /Hz, guess F = 1 dB P 0 = –18 dBm ! 2 – ampli flicker S ! = –132 dBrad 2 /Hz @ 1 Hz good RF amplifier 3 – merit factor Q = " 0 /2f L = 5·10 6 /5 = 10 6 (seems too low) 4 – take away some flicker for the output buffer: * flicker in the oscillator core is lower than –132 dBrad 2 /Hz @ 1 Hz * f L is higher than 2.5 Hz * the resonator Q is lower than 10 6 This is inconsistent with the resonator technology (expect Q > 10 6 ). The true Leeson frequency is lower than the frequency labeled as f L The 1/f 3 noise is attributed to the fluctuation of the quartz resonant frequency

basics – commercial oscillators Enrico Rubiola – The Leeson Effect – 22 Wenzel 501-04623 G - Lowest phase noise 100 MHz SC-cut oscillator manufacturer specs, phase noise -130 dBc/Hz @ 100 Hz -158 dBc/Hz @ 1 kHz -176 dBc/Hz @ 10 kHz -176 dBc/Hz @ 20 kHz ! 1 – floor S ! 0 = –173 dBrad 2 /Hz, guess F = 1 dB P 0 = 0 dBm 2 – merit factor Q = " 0 /2f L = 10 8 /7 × 103 = 1.4 × 10 4 (seems too low) From the literature, one expects Q ~ 10 5 . The true Leeson frequency is lower than the frequency labeled as f L The 1/f 3 noise is attributed to the fluctuation of the quartz resonant frequency

basics – commercial oscillators Enrico Rubiola – The Leeson Effect – 23 Courtesy of OEwaves (handwritten notes are mine). Cut from the oscillator specifications available at the URL http://www.oewaves.com/products/pdf/TDALwave_Datasheet_012104.pdf

Enrico Rubiola – The Leeson Effect – 24 Theory Laplace transforms most (electrical) engineers are familiar with the generalized impedance Z = 1 Z = R Z = sL sC C R L

theory – Laplace transforms Enrico Rubiola – The Leeson Effect – 25

theory – Laplace transforms Enrico Rubiola – The Leeson Effect – 26