Special Relativity Peter, who is standing on the ground, starts his - PDF document

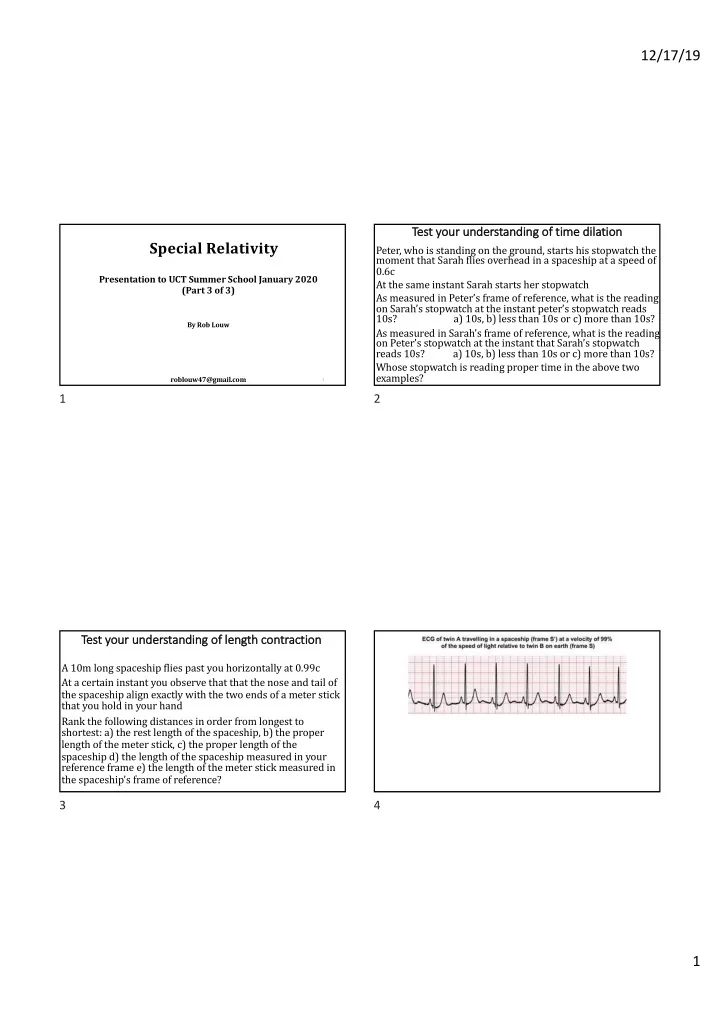
12/17/19 Te Test your understanding of time dilation Special Relativity Peter, who is standing on the ground, starts his stopwatch the moment that Sarah flies overhead in a spaceship at a speed of 0.6c Presentation to UCT Summer School January 2020 At the same instant Sarah starts her stopwatch (Part 3 of 3) As measured in Peter’s frame of reference, what is the reading on Sarah’s stopwatch at the instant peter’s stopwatch reads 10s? a) 10s, b) less than 10s or c) more than 10s? By Rob Louw As measured in Sarah’s frame of reference, what is the reading on Peter’s stopwatch at the instant that Sarah’s stopwatch reads 10s? a) 10s, b) less than 10s or c) more than 10s? Whose stopwatch is reading proper time in the above two examples? roblouw47@gmail.com 1 1 2 Te Test your understanding of length contraction A 10m long spaceship flies past you horizontally at 0.99c At a certain instant you observe that that the nose and tail of the spaceship align exactly with the two ends of a meter stick that you hold in your hand Rank the following distances in order from longest to shortest: a) the rest length of the spaceship, b) the proper length of the meter stick, c) the proper length of the spaceship d) the length of the spaceship measured in your reference frame e) the length of the meter stick measured in the spaceship’s frame of reference? 3 4 1

12/17/19 As we saw yesterday, space and time have become intertwined and we can no longer say that length and time have absolute meanings independent of the frame of reference Time and the three dimensions of space collectively for a four-dimensional entity called spacetime and we call x,y,z and t together the spacetime coordinates of an event Using the Lorentz coordinate transformations we can derive a set of Lorentz velocity transformations The result (without derivation) is shown in the next slide 6 5 6 The Lorentz velocity transformation shows that a body with a v x ’ = (v x – u)/(1- uv x /c 2 ) Lorentz velocity transformation speed less than c in one frame of reference always has a speed less than c in every other frame of reference In the extreme case where v x = c we get This is one reason for concluding that no material body may travel with a speed greater than or equal to the speed of light in a vacuum, relative to any inertial reference frame v x ’ = (c-u)/( 1 -uc/c 2 ) = c( 1 -u/c)/( 1 -u/c) = c This means that anything moving at c measured in S is also travelling at c when measured in S’ despite the relative motion of the two frames 7 10 7 10 2

12/17/19 Let's consider an example of the velocity limit which any Re Relative rocket ship speeds observer can reach relative to some other observer 1 Rocket speeds relative to speed of light c as as observed on earth 0 .9 If we had a set of five spaceships stacked like Russian dolls 0 .8 0 .7 where each ship could launch the remaining ships at a 0 .6 velocity equal to the relative velocity of the launching ship as 0 .5 observed from earth what relative velocities could the 0 .4 various ships achieve relative to the earth observer? 0 .3 0 .2 The following slide shows the velocity profiles of the five 0 .1 Mot her sh ip Ro cket 1 Ro cket 2 Ro cket 3 Ro cket 4 Ro cket 5 spaceships relative to an earth observer 0 0 0 .1 0 .2 0 .3 0 .4 0 .5 0 .6 0 .7 0 .8 0 .9 1 Rocket speeds relative to speed of light c observed by successive ship 11 observers when u=v 12 11 12 Relative rocket ship speeds Re Re Relative rocket ship speeds 1 1 Rocket speeds relative to speed of Rocket speeds relative to speed of light c as as observed on earth light c as as observed on earth 0 .9 0 .9 0 .8 0 .8 0 .7 0 .7 0 .6 0 .6 0 .5 0 .5 0 .4 0 .4 0 .3 0 .3 0 .2 0 .2 0 .1 0 .1 Mot her sh ip Ro cket 1 Ro cket 2 Ro cket 3 Ro cket 4 Ro cket 5 Mot her sh ip Ro cket 1 Ro cket 2 Ro cket 3 Ro cket 4 Ro cket 5 0 0 0 0 .1 0 .2 0 .3 0 .4 0 .5 0 .6 0 .7 0 .8 0 .9 1 0 0 .1 0 .2 0 .3 0 .4 0 .5 0 .6 0 .7 0 .8 0 .9 1 Rocket speeds relative to speed of light c observed by successive ship Rocket speeds relative to speed of light c observed by successive ship observers when u=v 13 observers when u=v 14 13 14 3

12/17/19 Relative rocket ship speeds Re Re Relative rocket ship speeds 1 1 Rocket speeds relative to speed of Rocket speeds relative to speed of light c as as observed on earth light c as as observed on earth 0 .9 0 .9 0 .8 0 .8 0 .7 0 .7 0 .6 0 .6 0 .5 0 .5 0 .4 0 .4 0 .3 0 .3 0 .2 0 .2 0 .1 0 .1 Mot her sh ip Ro cket 1 Ro cket 2 Ro cket 3 Ro cket 4 Ro cket 5 Mot her sh ip Ro cket 1 Ro cket 2 Ro cket 3 Ro cket 4 Ro cket 5 0 0 0 0 .1 0 .2 0 .3 0 .4 0 .5 0 .6 0 .7 0 .8 0 .9 1 0 0 .1 0 .2 0 .3 0 .4 0 .5 0 .6 0 .7 0 .8 0 .9 1 Rocket speeds relative to speed of light c observed by successive ship Rocket speeds relative to speed of light c observed by successive ship observers when u=v 15 observers when u=v 16 15 16 Re Relative rocket ship speeds Relative rocket ship speeds Re 1 1 Rocket speeds relative to speed of Rocket speeds relative to speed of light c as as observed on earth light c as as observed on earth 0 .9 0 .9 0 .8 0 .8 0 .7 0 .7 0 .6 0 .6 No matter how many 0 .5 0 .5 successive rockets are 0 .4 0 .4 0 .3 0 .3 launched their velocity 0 .2 0 .2 will never exceed c ! 0 .1 0 .1 Mot her sh ip Ro cket 1 Ro cket 2 Ro cket 3 Ro cket 4 Ro cket 5 Mot her sh ip Ro cket 1 Ro cket 2 Ro cket 3 Ro cket 4 Ro cket 5 0 0 0 0 .1 0 .2 0 .3 0 .4 0 .5 0 .6 0 .7 0 .8 0 .9 1 0 0 .1 0 .2 0 .3 0 .4 0 .5 0 .6 0 .7 0 .8 0 .9 1 Rocket speeds relative to speed of light c observed by successive ship Rocket speeds relative to speed of light c observed by successive ship observers when u=v 17 observers when u=v 18 17 18 4

12/17/19 Here we go with another thought experiment involving the Re Relativistic kinematics and the Doppler use of a high-speed train ef effect for electromagnetic wave ves A source of light is moving towards Stanley with constant speed u who is in a stationery inertial reference frame S The source emits light emits light waves of frequency f 0 as Measured in its rest frame Stanley receives light waves of frequency f as shown below 34 33 34 Here we go with another thought experiment involving the use of a high-speed train A source of light is moving towards Stanley with constant speed u who is in a stationery inertial reference frame S The source emits light waves of frequency f 0 as measured in its rest frame Stanley receives light waves of frequency f as shown in the next slide 36 37 36 37 5

12/17/19 With an electromagnetic source approaching an observer, With light, unlike sound, there is no distinction between the relativistic blue shift Doppler formula can be derived motion of source and motion of observer, only the relative using the appropriate Lorentz transforms and is velocity of the two is significant f = (𝐝 + 𝐯)/(𝐝 − 𝐯) f 0 Doppler formula (blue shift) The following slide illustrates the Doppler blue shift effect The doppler blue shift equation indicates that f increases i.e. the wavelength gets shorter (bluer) as u approaches the speed of light c 39 40 39 40 Do Doppl ppler effect- so source e ap approac aching obser server er With electromagnetic waves moving away from an observer, the relativistic red shift Doppler formula can be derived f/f 0 using the appropriate Lorentz transforms 2 0 As the source velocity - u 1 8 f/f 0 = (𝒅 + 𝒗)/(𝒅 − 𝒗) approaches the speed of f = (𝐝 − 𝐯)/(𝐝 + 𝐯) f 0 Doppler formula (red shift) 1 6 light, f/f 0 approaches 1 4 1 2 infinity (BLUE SHIFT) The doppler red shift equation indicates that f decrease i.e. 1 0 8 the wavelength gets longer (redder) as u approaches the 6 speed of light c 4 2 0 0 0 .1 0 .2 0 .3 0 .4 0 .5 0 .6 0 .7 0 .8 0 .9 1 Speed v relative to the speed of light c (v/c) 42 43 42 43 6

12/17/19 Doppl Do ppler effect- so source e movi ving away from obser server er Note that in deriving the Doppler equations, 𝛿 has cancelled out f/f 0 1 0 .9 The Doppler red shift effect is shown in the next few slides f/f 0 = (𝒅 + 𝒗)/(𝒅 − 𝒗) 0 .8 0 .7 0 .6 As the source velocity u 0 .5 0 .4 approaches the speed of 0 .3 light, f/f 0 approaches 0 .2 zero (red SHIFT) 0 .1 0 0 0 .1 0 .2 0 .3 0 .4 0 .5 0 .6 0 .7 0 .8 0 .9 1 Speed v relative to the speed of light c (v/c) 44 46 44 46 Hubble Queen Mary 2’s radar photograph of antennae a fast moving, Doppler blue shifted jet emanating from a black hole at the centre of Galaxy M87 47 48 47 48 7

12/17/19 Radar equipment installation at an airport 49 50 49 50 Re Relativistic particle phy hysics 51 51 52 8