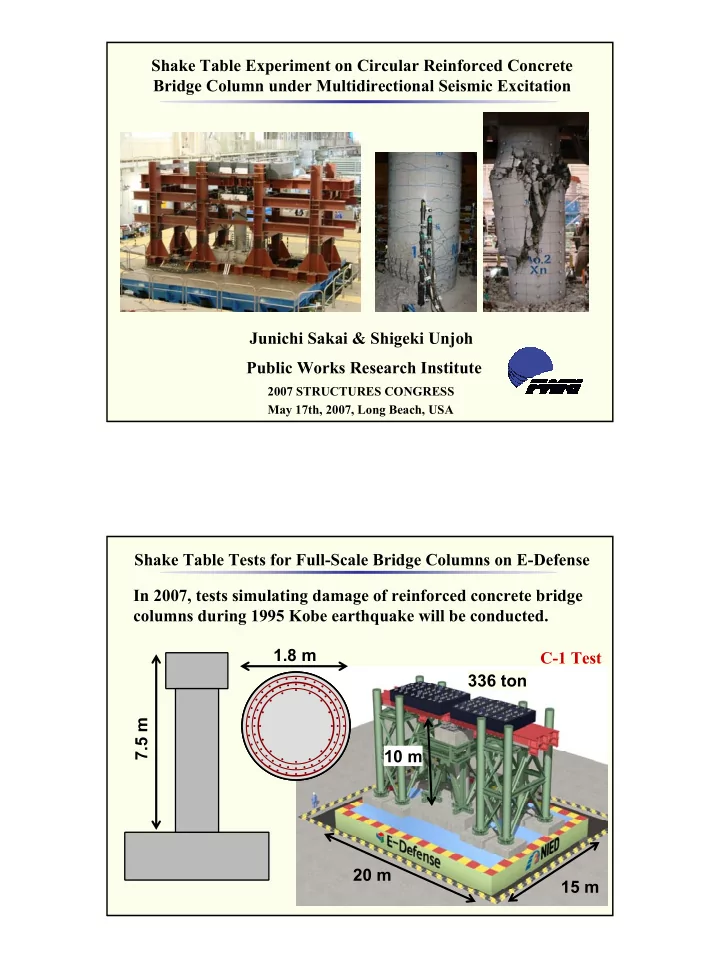
Shake Table Experiment on Circular Reinforced Concrete Bridge Column - PDF document

Shake Table Experiment on Circular Reinforced Concrete Bridge Column under Multidirectional Seismic Excitation Junichi Sakai & Shigeki Unjoh Public Works Research Institute 2007 STRUCTURES CONGRESS May 17th, 2007, Long Beach, USA Shake Table Tests for Full-Scale Bridge Columns on E-Defense In 2007, tests simulating damage of reinforced concrete bridge columns during 1995 Kobe earthquake will be conducted. 1.8 m C-1 Test 336 ton 軸方向鉄筋 SD295 軸方向鉄筋 SD295 7.5 m D29-32本×2.5段 D29-32本×2.5段 横拘束筋 SD295 横拘束筋 SD295 外： D13@150 外： D13@150 中： D13@300 中： D13@300 内： D13@300 内： D13@300 10 m 20 m 15 m

Full-Scale and Small-scaled Bridge Models E-Defense Shake table tests 336 ton of Small-scaled models have been conducted on PWRI shake table. 7.5 m PWRI 38 ton 2.5 m 15 m 20 m 8 m 8 m Loading Capacity: 1200 tf Loading Capacity: 300 tf Purpose of Shake Table Tests for Small-Scaled Models � To investigate dynamic behavior and failure mode of reinforced concrete bridge column under multidirectional loading � To provide test data to investigate the specimen size effects by comparison of results between small and full size specimens � To provide data to calibrate analytical models to simulate C-1 model tests on E-Defense � To check how the test setup of E-Defense test works Bearings, Instrumentation 1. Test for cantilever type specimen (in January 2006) 2. Test using similar setup of C-1 tests (in December 2006) a. Column that fails in flexure at bottom of column b. Column that fails in shear at cut-off point of longitudinal bars

Shake Table Tests for Cantilever-Type Specimen 1/4 Scaled Model 600 mm (23.6 in.) C.G. f = 41.6 MPa co 3 m 0.6 m Longitudinal: Hoops: 40@D10 (No. 3) D6 (No.2)@75 mm ρ ρ = 1.01% = 0.31% l s f f = 351 MPa = 340 MPa sy sy Natural Period: 0.3 sec (X, Y) 0.08 sec (Z) Input Ground Motion 1983 Nihonkai Chubu, Japan, earthquake One of Design Ground Motions of JRA (Type I, G.C.-III) Amplitude = 400% Time = 50% X (LG): PGA= 11.1 m/sec 2 10 (2.8 m/sec 2 ) Acceleration (m/sec 2 ) 0 -10 Y (TR): PGA= 9.5 m/sec 2 10 (2.4 m/sec 2 ) 0 -10 Z (UD): PGA= 8.2 m/sec 2 5 (2.1 m/sec 2 ) 0 Time (sec) -10 0 10 20 30 40 50

Global Response of Cantilever-Type Specimen Z Xn 400% of Tsugaru Bridge Yn Yp Response Ductility = 12~13 (Movie) Xp Local Response of Cantilever-Type Specimen Local Response (Xn Face) 400% of Tsugaru Bridge Yp Yn (Movie)

Response Displacement and Damage Progress 0.2 0.2 X direction Orbit Displacement (m) 0.1 d u Disp. in Y (m) d u 3 0 0 2 d u 1 -0.1 1 Time (sec) 2 3 -0.20 -0.2 -0.2 10 20 30 -0.2 0 0.2 Disp. in X (m) 1 2 3 Calibration of Analytical Model Damping Assumption 0.1 Rayleigh Damping Damping Ratio ξ = 2% ξ = 0.1% 0.05 0 (Hz) 0 10 20 30 40 50 Concrete Model 60 Stress (MPa) Mander Fiber Element 40 20 Hoshikuma (JRA) Cover Strain 0 Buckling, Fracture are not considered. 0 0.005 0.01

Accuracy of Analysis -Effect of Damping- 400% of Tsugaru Bridge Hoshikuma et al. (1997) Rebar Buckling 0.2 X direction Test 0 Displacement (m) Rayleigh Damping ξ = 2% ξ = 0.1% -0.2 Rebar Fracture 0.2 Y direction 0 -0.2 0 5 10 15 20 Time (sec) Accuracy of Analysis -Effect of Concrete Model- ξ = 0.1% 400% of Tsugaru Bridge Rebar Buckling 0.2 X direction Test 0 Displacement (m) Concrete Models Hoshikuma -0.2 Mander 0.2 Rebar Fracture Y direction 0 -0.2 0 5 10 15 20 Time (sec)

Test for Small-Scaled Models Using Similar Setup of C-1 Tests 1. Test for cantilever type specimen (in January 2006) 2. Test using similar setup of C-1 tests (in December 2006) a. Column that fails in flexure at bottom of column b. Column that fails in shear at cut-off point of longitudinal bars E-Defense 336 ton 1/3 Scaled Model PWRI 38 ton 7.5 m 2.5 m 8 m 8 m 15 m 20 m Details of Boundary Conditions � Safety Consideration � 3 Dimensional Earthquake Excitation END-SUPPORT END-SUPPORT SPECIMEN FIX 端部支点 端部支点 橋脚模型 橋脚模型 端部支点 端部支点 MOVE FIX : FIX for LG & TR; FREE for Rotation : FIX for TR; FREE for LG & Rotation : Slide Bearing Inertia Force in LG: Only Specimen Carries Inertia Force in TR: Specimen and End Supports Carry Axial Force: Depends on Locations of Weights

Specimens Flexural Failure Model Shear Failure Model Hoops Longitudinal Longitudinal Hoops D3 D10 D10 D3 O: 50 O: 50 M: 100 40 bars O: 100 I : 100 80 bars Cut-Off 2 m O: 100 80 bars O: 100 M: 100 M: 100 Cut-Off O: 100 I : 100 M: 100 100 bars I : 100 O: 50 M: 100 O: 50 I : 100 M: 100 I : 100 O: Outside M: Middle I : Inside Global Response of Flexural Failure Model 80% of JR Takatori (Movie)

Damage Progress of Flexural Failure Model 80% of JR Takatori 2.6 sec 3.1 sec (Movie) 3.6 sec 4.4 sec Response Displacement of Flexural Failure Model 0.2 80% of JR Takatori 0.2 Displacement (m) Disp in TR (m) LG 0.1 0 0 -0.1 TR -0.2 -0.2 -0.2 0 0.2 0 2 4 6 8 10 Disp in LG (m) 3.1 sec 3.6 sec 4.4 sec

Global Response of Shear Failure Model 80% of JR Takatori (Movie) Damage Progress of Shear Failure Model 80% of JR Takatori (Movie) 2.9 sec 3.0 sec 3.1 sec 3.7 sec 4.4 sec

Response Displacement of Shear Failure Model 80% of JR Takatori 0.2 Displacement (m) LG At 3 sec, specimen hit 0.1 the supporting frame. 0 At 4.4 sec, specimen is -0.1 TR totally supported by the frame due to shear failure. -0.2 0 2 4 6 8 10 2.9 sec 3.0 sec 3.1 sec 3.7 sec 4.4 sec Conclusions • Dynamic behavior and damage progress of reinforced concrete columns under multidirectional earthquake excitation are investigated through shake table tests. • Failure mechanisms of bridge columns that damaged during 1995 Kobe earthquake are simulated on shake table. • Analytical models are calibrated using the test results. Analytical simulation of shake table tests of full-scale bridge model on E-Defense is being conducted.

APPENDICES Shake Tables E-Defense PWRI Table Size 20 m × 15 m 8 m × 8 m Loading Capacity 1200 tf 300 tf Max. Acceleration 9 (15) m/sec 2 20 (10) m/sec 2 Max. Velocity 2 (0.7) m/sec 2 (1) m/sec Max. Displacement ± 1 (0.5) m ± 0.6 (0.3) m ( ): Capacity for Vertical Direction

Scaling Factors Diameter of Column E-Defense: 1.8 m; PWRI: 0.6 m Scaling Factor Target Length （ L ） S 3 Time （ T ） S 1.73 Mass （ M ） S 2 9 Acceleration （ LT -2 ） 1 1 Velocity （ LT -1 ） S 1.73 Displacement （ L ） S 3 Elastic Modulus （ ML -1 T -2 ） 1 1 Strain （ 1 ） 1 1 Force （ MLT -2 ） S 2 9 Stiffness （ MT -2 ） S 3 Moment （ ML 2 T -2 ） S 3 27