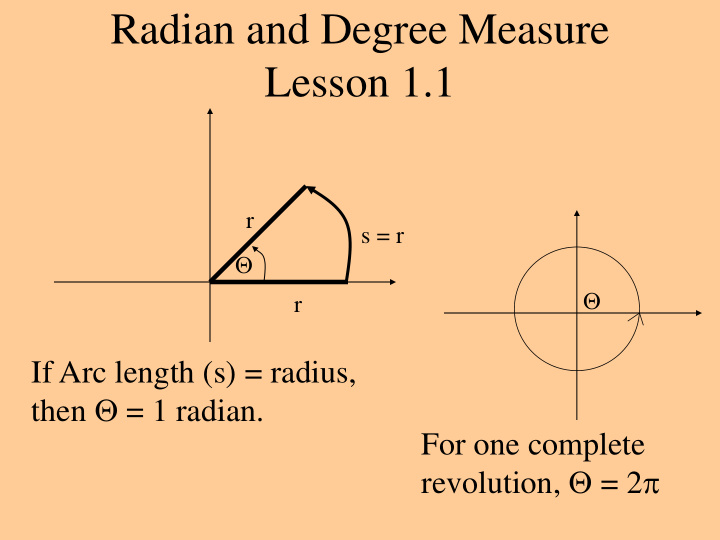
Radian and Degree Measure Lesson 1.1 r s = r r If Arc length - PowerPoint PPT Presentation

Radian and Degree Measure Lesson 1.1 r s = r   r If Arc length (s) = radius, then  = 1 radian. For one complete revolution,  = 2 

 /2  1.57 rad Quadrant II Quadrant I 2 1 3  0, 2   6.35 rad  3.14 rad 6 Quadrant III 4 Quadrant IV 5 3  /2  4.72 rad For positive angles

- 3  /2  - 4.72 rad Quadrant II Quadrant I - 5 - 4 - 6 -  0, - 2  -  - 3.14 rad  -6.35 rad - 3 - 1 Quadrant III Quadrant IV - 2 -  /2  - 1.57 rad For negative angles

Ex 1: Estimate the angle to the nearest 1/2 radian. A. C. 2.5 rad - 1 rad B. 3.5 rad

Ex 2: Determine the quadrant in which each angle lies. A.  /5 B. 7  /5  0 Quad I Quad III C. -  /12 D. - 3.5 Quad II Quad IV

Acute Angles - angles that have a measure 0 <  <  /2 radians Obtuse Angles - angles that have a measure  /2 <  <  radians

Ex 3: Sketch each angle in standard position. A. 2  /3 B. 5  /4 C. - 7  /4 D. 3

Coterminal - two angles that share the same terminal side.     One positive angle Two positive angles + One negative angle

Ex 4: Determine two co-terminal angles (one positive and one negative) for each angle.    2 6 A.   12 + 2   /6   6 6   13 6    12   2   6 6 6    11 6

Ex 4 (cont’d): Determine two co -terminal angles (one positive and one negative) for each angle. B. 5  /6  5  5   2   2 Negative: Positive: 6 6   5 12   5 12     6 6 6 6    7   17 6 6

C. - 2  /3     2 2 6  4       2 Positive: 3 3 3 3    2 6   8  2    Negative:    2 3 3 3 3   25    24 D.  /12     2 Positive: 12 12 12 12     24   23     2 Negative: 12 12 12 12

Complementary angles - two angles whose sum is  /2 radians Supplementary angles - two angles whose sum is  radians Ex 5: Find, if possible, the complement and supplement of each angle A.  /3         3 2   x     x Compl.: 6 3 2 2 3 6 6   2 Suppl.:     3 3    x      x 3 3 3 3

Ex 5 (cont’d): Find, if possible, the complement and supplement of each angle B. 3  /4   3  Complementary angle does not exist. Compl.: 4 2       Suppl.: 3 3 4 3    x    4   x 4 4 4 4

Ex 5 (cont’d): Find, if possible, the complement and supplement of each angle C. 1 D. 2 Compl.: Compl.:     Does not   x   1 x 1 2 2 2 2 exist . Suppl.: 1    x Suppl.: 2    x x    1 x    2 Homework: p.138 #2-24 even