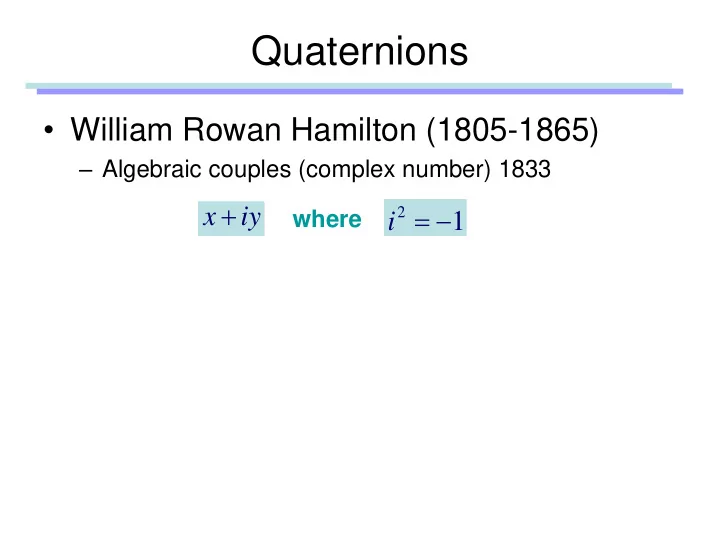

Quaternions • William Rowan Hamilton (1805-1865) – Algebraic couples (complex number) 1833 x iy 2 where i 1
Quaternions • William Rowan Hamilton (1805-1865) – Algebraic couples (complex number) 1833 x iy 2 where i 1 – Quaternions 1843 2 2 2 w ix jy kz i j k ijk 1 where ij k , jk i , ki j ji k , kj i , ik j
Quaternions William Thomson “… though beautifully ingenious, have been an unmixed evil to those who have touched them in any way.” Arthur Cayley “… which contained everything but had to be unfolded into another form before it could be understood.”
Unit Quaternions • Unit quaternions represent 3D rotations 3 S q w ix jy kz ( w , x , y , z ) ( w , v ) 2 2 2 2 w x y z 1
Rotation about an Arbitrary Axis ˆ • Rotation about axis by angle v ˆ v ( x , y , z ) ( x , y , z ) v ˆ q cos , sin 2 2 1 p ( 0 , x , y , z ) p qpq where Pur urely y Im Imaginary Qua uaternion
Unit Quaternion Algebra • Identity q ( 1 , 0 , 0 , 0 ) q q ( w , v )( w , v ) • Multiplication 1 2 1 1 2 2 ( w w v v , w v w v v v ) 1 2 1 2 1 2 2 1 1 2 1 2 2 2 2 q ( w , x , y , z ) /( w x y z ) • Inverse 2 2 2 2 ( w , x , y , z ) /( w x y z ) • Unit quaternion space is – closed under multiplication and inverse, – but not closed under addition and subtraction
Unit Quaternion Algebra • Antipodal equivalence – q and – q represent the same rotation R R ( p ) ( p ) q q 3 – 2-to-1 mapping between S and SO(3) – Twice as fast as in SO(3)
Rotation Composition v • Rotation by a matrix Mv Mv • Rotation by a unit quaternion 1 v qvq • Composition of Matrices (or Unit quaternions) is simple multiplication 1 1 v q q vq vq q v M M v 2 1 1 2 2 1
Acknowledgement • Acknowledgement: Some materials come from the lecture slides of – Prof. Jehee Lee, SNU, http://mrl.snu.ac.kr/courses/CourseGraphics/index_2017spring.html
Recommend
More recommend