QUASILINEAR PREFERENCES Utility additive, y and linear in y : U ( - PDF document

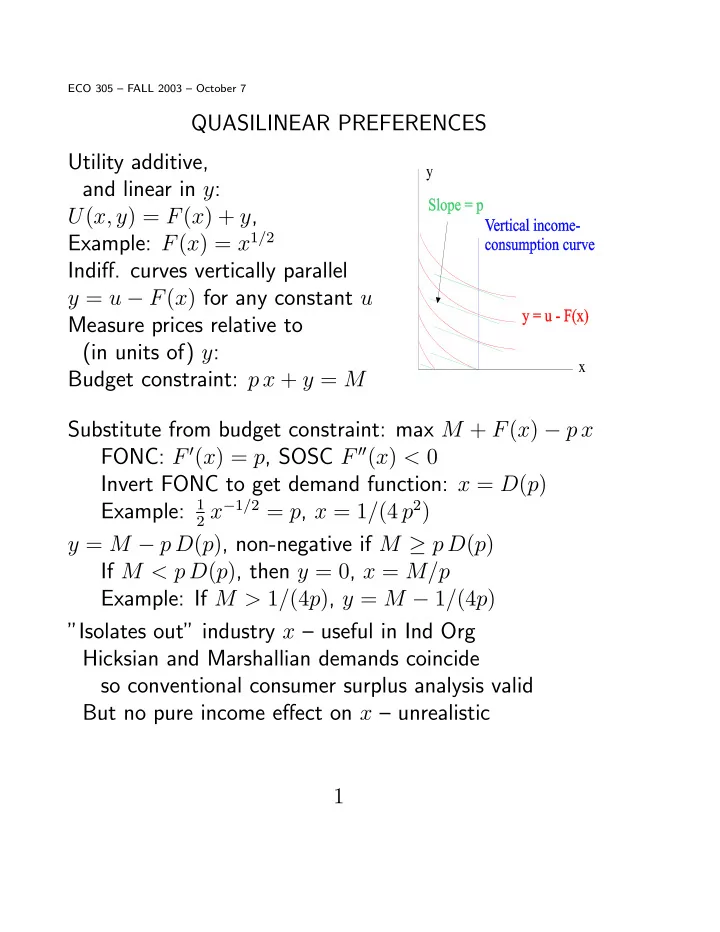
ECO 305 — FALL 2003 — October 7 QUASILINEAR PREFERENCES Utility additive, y and linear in y : U ( x, y ) = F ( x ) + y , Example: F ( x ) = x 1 / 2 Indi ff . curves vertically parallel y = u − F ( x ) for any constant u Measure prices relative to (in units of) y : x Budget constraint: p x + y = M Substitute from budget constraint: max M + F ( x ) − p x FONC: F 0 ( x ) = p , SOSC F 00 ( x ) < 0 Invert FONC to get demand function: x = D ( p ) 2 x − 1 / 2 = p , x = 1 / (4 p 2 ) Example: 1 y = M − p D ( p ) , non-negative if M ≥ p D ( p ) If M < p D ( p ) , then y = 0 , x = M/p Example: If M > 1 / (4 p ) , y = M − 1 / (4 p ) ”Isolates out” industry x — useful in Ind Org Hicksian and Marshallian demands coincide so conventional consumer surplus analysis valid But no pure income e ff ect on x — unrealistic 1

REVEALED PREFERENCE Inferring indi ff erence map from observed demands Vector notation: Budget constraint P · x ≤ M Demand function x = D ( P , M ) . If these satisfy [1] Adding up: P · D ( P , M ) ≡ M [2] Homogeneity: D ( k P , k M ) = D ( P , M ) [3] Internal Consistency: axiom SARP derived below then there are underlying preferences being maximized DEFINITION: Suppose x a = D ( P a , M a ) . Call x a revealed preferred to x b if x b is on or within the budget constraint that led to the choice of x a . More formally: x a RP x b if P a · x b ≤ P a · x a y WARP satisfied violated b a x WARP “Weak axiom of revealed preference”: If x a RP x b is true, then x b RP x a should be false, that is, If P a · x b ≤ P a · x a , then P b · x a > P b · x b 2

Can construct chain of revealed preferences. Revealed indi ff erence curve through x a is traced out by envelope of the budget lines as chain gets fi ner and fi ner. x 2 c c c P .X = M d X c X a a a b P .X = M X a X b b b x P .X = M 1 Consistency of preferences requires SARP “strong axiom of revealed preference”: for any chain a , b , c , . . . j , k , If x a RP x b , x b RP x c , . . . x j RP x k , then x k RP x a false 3

ANOMALIES Psychologists and experimental researchers fi nd behavior inconsistent with rational choice Framing and endowment e ff ects: Preference depends on how posed and status quo, not just on actual fi nal consumption Co ff ee-mug experiments Lost-ticket vs. lost-money fi ndings Recent experiments show endowment e ff ect decreases as trading experience increases Taxi drivers’ daily target income behavior refuted by Prof. Farber Time inconsistency: trade-o ff between day 2 and day 3 looks di ff erent on day 2 than it did on day 1 Example — When you are 20, you plan to save a lot of your income in your 30’s, but when the 30’s come along . . . Other anomalies later: (1) choices under uncertainty, game interactions e.g. prisoner’s dilemma General lesson — use standard theory as your starting point, but may need to supplement/modify 4