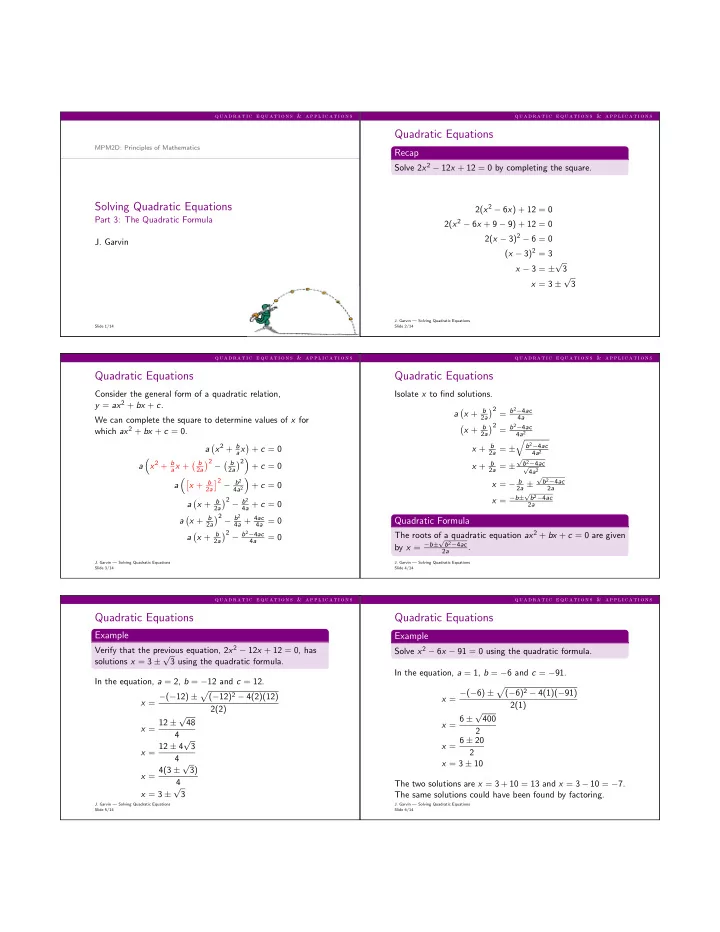
Quadratic Equations MPM2D: Principles of Mathematics Recap Solve 2 - PDF document

q u a d r a t i c e q u a t i o n s & a p p l i c a t i o n s q u a d r a t i c e q u a t i o n s & a p p l i c a t i o n s Quadratic Equations MPM2D: Principles of Mathematics Recap Solve 2 x 2 − 12 x + 12 = 0 by completing the square. Solving Quadratic Equations 2( x 2 − 6 x ) + 12 = 0 Part 3: The Quadratic Formula 2( x 2 − 6 x + 9 − 9) + 12 = 0 2( x − 3) 2 − 6 = 0 J. Garvin ( x − 3) 2 = 3 √ x − 3 = ± 3 √ x = 3 ± 3 J. Garvin — Solving Quadratic Equations Slide 1/14 Slide 2/14 q u a d r a t i c e q u a t i o n s & a p p l i c a t i o n s q u a d r a t i c e q u a t i o n s & a p p l i c a t i o n s Quadratic Equations Quadratic Equations Consider the general form of a quadratic relation, Isolate x to find solutions. y = ax 2 + bx + c . � 2 = b 2 − 4 ac � x + b a 2 a 4 a We can complete the square to determine values of x for � 2 = b 2 − 4 ac which ax 2 + bx + c = 0. � x + b 2 a 4 a 2 � x 2 + b b 2 − 4 ac � � x + b a a x + c = 0 2 a = ± 4 a 2 √ � b � 2 − � b � x 2 + b � 2 � b 2 − 4 ac x + b a x + + c = 0 2 a = ± a √ 2 a 2 a 4 a 2 √ � 2 − b 2 �� � b 2 − 4 ac x = − b x + b 2 a ± a + c = 0 2 a 2 a 4 a 2 √ x = − b ± b 2 − 4 ac � 2 − b 2 � x + b a 4 a + c = 0 2 a 2 a � 2 − b 2 4 a + 4 ac � x + b a 4 a = 0 Quadratic Formula 2 a The roots of a quadratic equation ax 2 + bx + c = 0 are given � 2 − b 2 − 4 ac � x + b a = 0 √ 2 a 4 a b 2 − 4 ac by x = − b ± . 2 a J. Garvin — Solving Quadratic Equations J. Garvin — Solving Quadratic Equations Slide 3/14 Slide 4/14 q u a d r a t i c e q u a t i o n s & a p p l i c a t i o n s q u a d r a t i c e q u a t i o n s & a p p l i c a t i o n s Quadratic Equations Quadratic Equations Example Example Verify that the previous equation, 2 x 2 − 12 x + 12 = 0, has Solve x 2 − 6 x − 91 = 0 using the quadratic formula. √ solutions x = 3 ± 3 using the quadratic formula. In the equation, a = 1, b = − 6 and c = − 91. In the equation, a = 2, b = − 12 and c = 12. ( − 6) 2 − 4(1)( − 91) � x = − ( − 6) ± � ( − 12) 2 − 4(2)(12) x = − ( − 12) ± 2(1) 2(2) √ √ x = 6 ± 400 x = 12 ± 48 2 4 √ x = 6 ± 20 x = 12 ± 4 3 2 4 √ x = 3 ± 10 x = 4(3 ± 3) 4 The two solutions are x = 3 + 10 = 13 and x = 3 − 10 = − 7. √ x = 3 ± 3 The same solutions could have been found by factoring. J. Garvin — Solving Quadratic Equations J. Garvin — Solving Quadratic Equations Slide 5/14 Slide 6/14

q u a d r a t i c e q u a t i o n s & a p p l i c a t i o n s q u a d r a t i c e q u a t i o n s & a p p l i c a t i o n s Quadratic Equations Quadratic Equations Example Determine the x -intercepts of the parabola defined by � 6 2 − 4(3)( − 15) x = − 6 ± y = − 3 x 2 − 6 x + 15. 2(3) √ x = − 6 ± 216 The x -intercepts correspond to the solutions to the quadratic equation − 3 x 2 − 6 x + 15 = 0. 6 √ x = − 6 ± 6 6 Since moving all three terms to the other side of the 6 equation results in the equation 3 x 2 + 6 x − 15 = 0, this √ x = − 1 ± 6 equation may also be used. It has the advantage of avoiding a negative denominator. The x -intercepts are approximately − 3 . 45 and 1 . 45. J. Garvin — Solving Quadratic Equations J. Garvin — Solving Quadratic Equations Slide 7/14 Slide 8/14 q u a d r a t i c e q u a t i o n s & a p p l i c a t i o n s q u a d r a t i c e q u a t i o n s & a p p l i c a t i o n s Quadratic Equations Quadratic Equations Example Solve 2 x 2 − x + 5 = 0. In the equation, a = 2, b = − 1 and c = 5. � ( − 1) 2 − 4(2)(5) x = − ( − 1) ± 2(2) x = 1 ± √− 39 4 At this point there is a problem, since √− 39 is not a real number. Therefore, there can be no real solutions to this equation. J. Garvin — Solving Quadratic Equations J. Garvin — Solving Quadratic Equations Slide 9/14 Slide 10/14 q u a d r a t i c e q u a t i o n s & a p p l i c a t i o n s q u a d r a t i c e q u a t i o n s & a p p l i c a t i o n s The Discriminant The Discriminant Let D = b 2 − 4 ac , the expression inside of the radical sign in To determine the number of real solutions to a quadratic the quadratic formula. relation, it is only necessary to calculate the value of the discriminant. This expression is known as the discriminant , and it can be used to give us information about both the number of Determining the Number of Real Roots real-valued solutions for a quadratic equation. The number of real solutions to a quadratic equation √ ax 2 + bx + c = 0 can be determined using the discriminant, If D < 0, then D will be undefined for any real numbers. D = b 2 − 4 ac . √ If D = 0, then D = 0, and the quadratic formula will 2 a , for which there is one ∗ real solution. • If D < 0, there are no real solutions. become x = − b • If D = 0, there is one real solution. √ If D > 0, then the quadratic formula is x = − b ± D , for 2 a • If D > 0, there are two distinct real solutions. which there are two distinct real solutions. J. Garvin — Solving Quadratic Equations J. Garvin — Solving Quadratic Equations Slide 11/14 Slide 12/14

q u a d r a t i c e q u a t i o n s & a p p l i c a t i o n s q u a d r a t i c e q u a t i o n s & a p p l i c a t i o n s The Discriminant Questions? Example Determine the number of real solutions to 4 x 2 − 20 x + 25 = 0. Since D = ( − 20) 2 − 4(4)(25) = 0, there is one real solution. Example Determine the number of real solutions to 3 x 2 + 5 x + 4 = 0. Since D = 5 2 − 4(3)(4) = − 23, there are no real solutions. J. Garvin — Solving Quadratic Equations J. Garvin — Solving Quadratic Equations Slide 13/14 Slide 14/14