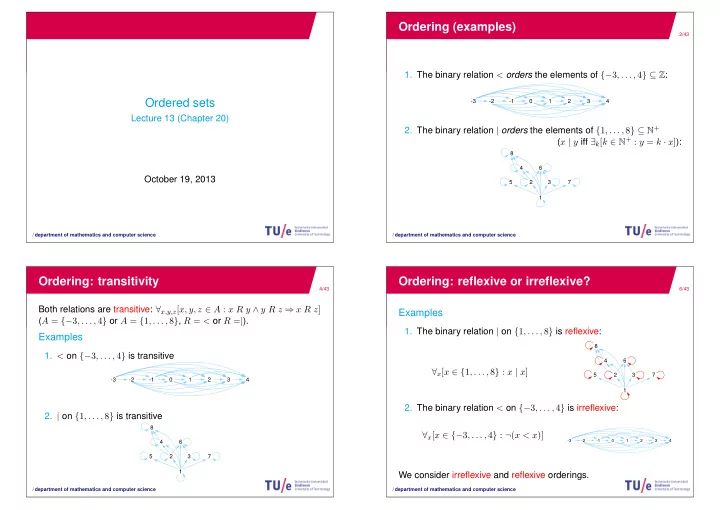

Ordering (examples) 2/43 1. The binary relation < orders the elements of { � 3 , . . . , 4 } ✓ Z : Ordered sets -3 -2 -1 0 1 2 3 4 Lecture 13 (Chapter 20) 2. The binary relation | orders the elements of { 1 , . . . , 8 } ✓ N + ( x | y iff 9 k [ k 2 N + : y = k · x ] ): 8 4 6 October 19, 2013 5 2 3 7 1 / department of mathematics and computer science / department of mathematics and computer science Ordering: transitivity Ordering: reflexive or irreflexive? 4/43 6/43 Both relations are transitive: 8 x,y,z [ x, y, z 2 A : x R y ^ y R z ) x R z ] Examples ( A = { � 3 , . . . , 4 } or A = { 1 , . . . , 8 } , R = < or R = | ). 1. The binary relation | on { 1 , . . . , 8 } is reflexive: Examples 8 1. < on { � 3 , . . . , 4 } is transitive 4 6 8 x [ x 2 { 1 , . . . , 8 } : x | x ] 5 2 3 7 -3 -2 -1 0 1 2 3 4 1 2. The binary relation < on { � 3 , . . . , 4 } is irreflexive: 2. | on { 1 , . . . , 8 } is transitive 8 8 x [ x 2 { � 3 , . . . , 4 } : ¬ ( x < x )] -3 -2 -1 0 1 2 3 4 4 6 5 2 3 7 1 We consider irreflexive and reflexive orderings. / department of mathematics and computer science / department of mathematics and computer science
Irreflexive is stronger than Not Reflexive Ordering: (strict) antisymmetry 7/43 9/43 irreflexive: 8 x [ x 2 A : ¬ ( x R x )] 8 x,y [ x, y 2 A : x R y ^ y R x ) NO ] . not reflexive: ¬ 8 x [ x 2 A : x R x ] x y R not reflexive does not imply R irreflexive : Define R on Z for all x 2 Z by: Example 1 The binary relation < on { � 3 , . . . , 4 } x R y if, and only if, y = 3 x . Then: -3 -2 -1 0 1 2 3 4 I R is not reflexive, for we have ¬ (1 R 1) , but I R is not irreflexive either, for we have 0 R 0 . is strictly antisymmetric: 8 x,y [ x, y 2 A : x R y ^ y R x ) False ] | {z } ¬ ( x R y ^ y R x ) R irreflexive does imply R not reflexive . / department of mathematics and computer science / department of mathematics and computer science Ordering: (strict) antisymmetry Reflexive orderings, Irreflexive orderings 10/43 11/43 I. Reflexive ordering h A, R i : 8 x,y [ x, y 2 A : x R y ^ y R x ) NO ] . I.1 reflexive: 8 x [ x 2 A : x R x ] x y I.2 antisymmetric: 8 x,y [ x, y 2 A : x R y ^ y R x ) x = y ] I.3 transitive: 8 x,y,z [ x, y, z 2 A : x R y ^ y R z ) x R z ] II. Irreflexive ordering h A, R i : Example 2 II.1 irreflexive: 8 x [ x 2 A : ¬ ( x R x )] The binary relation | on { 1 , . . . , 8 } II.2 strictly antisymmetric: 8 x,y [ x, y 2 A : ¬ ( x R y ^ y R x )] II.3 transitive: 8 x,y,z [ x, y, z 2 A : x R y ^ y R z ) x R z ] 8 NB: II.2 follows from II.1+II.3 (see Ex. 20.2); we may therefore omit 4 6 requirement II.2 from the definition. 5 2 3 7 Whenever you need to prove that a relation is an irreflexive ordering, 1 you only need to prove that it is irreflexive and transitive; you may omit the proof of strict antisymmetry. is antisymmetric: 8 x,y [ x, y 2 A : x R y ^ y R x ) x = y ] / department of mathematics and computer science / department of mathematics and computer science
h P ( A ) , ✓i is a reflexive ordering Examples 12/43 13/43 Proof: We need to prove that ✓ on P ( A ) is (1) reflexive, (2) antisymmetric and (3) transitive: I h N , i , h Z , i and h R , i are reflexive orderings 1. For arbitrary X 2 P ( A ) , X ✓ X follows directly from the I h N , < i , h Z , < i and h R , < i are irreflexive orderings definition of ✓ . Hence, ✓ on P ( A ) is reflexive. I h N + , | i is a reflexive ordering 2. Let X, Y 2 P ( A ) and suppose that X ✓ Y and Y ✓ X ; then [For proof of reflexivity: see the book; we prove antisymmetry; do X = Y follows directly from the definition of = on sets. transitivity yourself.] Hence, ✓ on P ( A ) is antisymmetric. I h P ( A ) , ✓i is a reflexive ordering 3. Let X, Y, Z 2 P ( A ) and suppose that X ✓ Y and Y ✓ Z . [Proof on next slide.] By the Property of ✓ , it holds for every element x 2 X that x 2 Y , so, again by the Property of ✓ , we get x 2 Z . It follows that X ✓ Z . Hence, ✓ on P ( A ) is transitive. / department of mathematics and computer science / department of mathematics and computer science Comparable Comparable (example 1) 14/43 15/43 Let h A, R i be a reflexive or irreflexive ordering, and let x, y 2 A . I h N + , | i Then there are three possibilities: 9 x y comparable? > > x R y ? yes/no > 3 15 X > > > y R x ? yes/no = 15 3 X sometimes ‘yes’, sometimes ‘no’ x = y ? yes/no 3 3 X > > > ⇥ 3 7 > > x and y are comparable if we get at least one time ‘yes’. > ; 7 3 ⇥ x and y are incomparable if we get three times ‘no’. / department of mathematics and computer science / department of mathematics and computer science
Comparable (example 2) Linear orderings (definition) 16/43 17/43 A (reflexive or irreflexive) ordering h A, R i is linear if every two elements are comparable , i.e., I h Z , i 8 x,y [ x, y 2 A : x R y _ y R x _ x = y ] | {z } may be omitted if R is reflexive 9 x y comparable? Examples > > > 3 15 X > > > = I h Z , i is a linear reflexive ordering and h Z , < i is a linear 15 3 X always ‘yes’! 3 3 X irreflexive ordering > > > 3 7 X > I h N + , | i is not linear > > ; 7 3 X NB: If h A, R i is a linear ordering, then the elements of A can be arranged on a straight line such that x 2 A is left of y 2 A iff xRy . [Example: recall the arrangement of h Z , < i on slide 2.] / department of mathematics and computer science / department of mathematics and computer science Direct successors/predecessors Direct successors 18/43 19/43 Examples 1. Let h A, R i be an irreflexive ordering I In h N , < i : • every n 2 N has a direct successor n + 1 , and y 2 A is a direct successor of x 2 A if • every n 2 N \{ 0 } has a direct predecessor n � 1 . x R y ^ ¬ 9 z [ z 2 A : x R z ^ z R y ] I In h R , < i there are no direct successors. y I In h N + , | i , every n 2 N has infinitely many direct successors. z 2. Let h A, R i be a reflexive ordering direct successors of 3 : 6 , 9 , 15 , . . . examples of numbers that are not direct y 2 A is a direct successor of x 2 A if x successors of 3 : • 3 (because direct successor should be distinct), x R y ^ x 6 = y ^ • 12 (because 3 | 6 | 12 ), ¬ 9 z [ z 2 A ^ z 6 = x ^ z 6 = y : x R z ^ z R y ] • 18 (because 3 | 6 | 18 and also 3 | 9 | 18 ), • 4 (because 3 - 4 ). NB: In h N + , | i , the number n is a direct successor of m if n = p · m with p prime. / department of mathematics and computer science / department of mathematics and computer science
Hasse diagrams Maximum/minimum 20/43 23/43 Orderings with direct predecessors/successors we can arrange as Let h A, R i be an ordering, and let A 0 ✓ A . Hasse diagrams. m 2 A 0 is the maximum of A 0 if I h { 2 , 3 , 4 , 5 , 6 } , | i ⇢ 8 x [ x 2 A 0 ^ x 6 = m : x R m ] A Hasse diagram has a connection for an irreflexive ordering between x and a y above it if, and 8 x [ x 2 A 0 : x R m ] for a reflexive ordering . 4 6 only if, y is a direct successor of x . m 2 A 0 is the minimum of A 0 : analogous 2 3 5 I h { 2 , 4 , 6 , 8 , 10 , 12 , 14 , 16 , 18 } , | i Examples: I h P ( { 0 , 1 } ) , ✓i 16 I Consider h { 2 , 3 , 4 , 5 , 6 } , | i { 0 , 1 } 8 12 18 maximum { 2 , 3 , 6 } : 6 4 6 minimum { 2 , 3 , 6 } : no minimum { 0 } { 1 } 10 4 6 14 maximum { 2 , 3 , 5 , 6 } : no maximum 2 3 5 ; 2 minimum { 2 , 3 , 5 , 6 } : no minimum / department of mathematics and computer science / department of mathematics and computer science Maximum/minimum Maximum/minimum 24/43 25/43 Let h A, R i be an ordering, and let A 0 ✓ A . Let h A, R i be an ordering, and let A 0 ✓ A . m 2 A 0 is the maximum of A 0 if m 2 A 0 is the maximum of A 0 if ⇢ 8 x [ x 2 A 0 ^ x 6 = m : x R m ] ⇢ 8 x [ x 2 A 0 ^ x 6 = m : x R m ] for an irreflexive ordering for an irreflexive ordering 8 x [ x 2 A 0 : x R m ] 8 x [ x 2 A 0 : x R m ] for a reflexive ordering . for a reflexive ordering . m 2 A 0 is the minimum of A 0 : analogous m 2 A 0 is the minimum of A 0 : analogous Examples: Examples: { 0 , 1 } I Consider h P ( { 0 , 1 } ) , ✓i I Consider h Z , i maximum P ( { 0 , 1 } ) : { 0 , 1 } maximum N : no maximum { 0 } { 1 } minimum P ( { 0 , 1 } ) : ; minimum N : 0 maximum Z : no maximum ; minimum Z : no minimum / department of mathematics and computer science / department of mathematics and computer science
Recommend
More recommend